PRACTICE PROBLEMS ON SIMILAR TRIANGLES

(1) Find the unknown values in each of the following figures. All lengths are given in centimeters (all measures are not in scale)

Solution

Solution

Solution

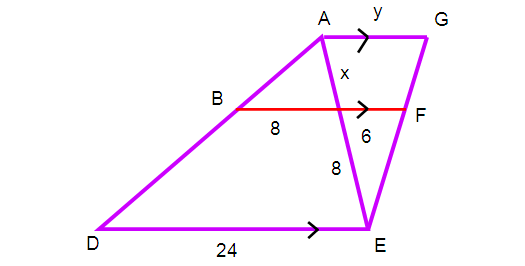
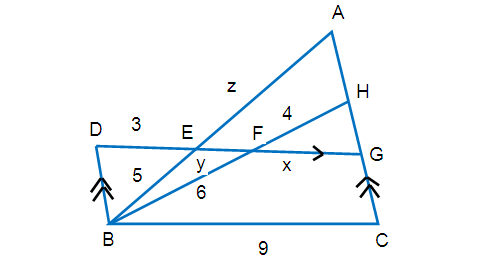
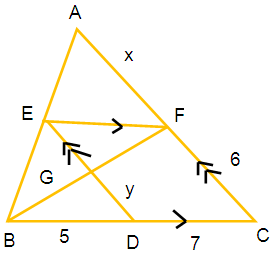
(2) A girl is in the beach with her father. She spots a swimmer drowning. She shouts to her father who is 50 m due west of her. Her father is 10 m nearer to a boat than the girl. If her father uses the boat to reach the swimmer, he has to travel a distance 126 m from the boat. At the same time, the girl spots a man riding a water craft who is 98 m away from the boat. The man n the water craft is due east of the swimmer. How far must the man travel to rescue the swimmer?       Solution

(3) P and Q are points on sides AB and AC respectively, of triangle ABC. If AP = 3 cm, PB = 6 cm, AQ = 5 cm and QC = 10 cm, show that BC = 3 PQ.       Solution

(4) In triangle ABC, AB = AC and BC = 6 cm. D is a point on the side AC such that AD = 5 cm and CD = 4 cm. Show that triangle BCD and triangle ACB are congruent and hence find BD.     Solution

(5) The points D and E are on the sides AB and AC of triangle ABC respectively, such that DE and BC are parallel. If AB = 3 AD and the area triangle ABC is 72 cm², then find the area of the quadrilateral DBCE. 

Solution

(6) The lengths of the three sides of triangle ABC are 6 cm, 4 cm and 9 cm. Triangle PQR ad BC are congruent. One of the lengths of the sides of triangle PQR is 35 cm. What is the greatest perimeter possible for triangle PQR

Solution

(7) In the figure, the sides DE and BC are parallel and (AD/B) = 3/5, calculate the value of

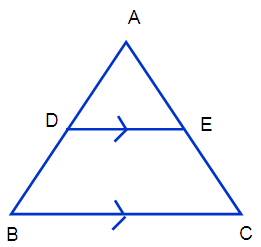
(i) area of triangle ADE/are of triangle ABC

(ii) area of trapezium BCED/area of triangle ABC

Solution

(8) The government plans to develop a new industrial zone in an unused portion of land in a city.

The shaded portion of the map shown given below indicates the area of the new industrial zone. Find the area of the new industrial zone.

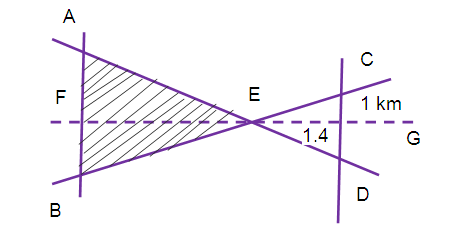
(9) A boy is designing a diamond shaped kite, as shown in the figure where AE = 16 cm, EC = 81 cm. He wants to use a straight cross bar BD. How long should it be? 

Solution

(10) A student wants to determine the height of flag pole. He placed a small mirror on the ground so that he can see reflection of the top of the flagpole. The distance of the mirror from him is 0.5 m and the distance of the flagpole from the mirror is 3 m .If t he eyes are 1.5 m above the ground level, then find the height of the flagpole (The foot of student, mirror and the foot of flagpole lie along the a straight line)      Solution

(11) A roof has a cross section as shown in the diagram

(i) Identify the similar triangles

 (ii) Find the height h of the roof.       Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.