COMPOUND INTEREST

We might have already learned about the stuff simple interest in solving interest and investment problems. We know that if the principal remains the same for the entire period or time then interest is called as simple interest.

We can define the compound interest as the interest accrues when earning for each specified period of time added to the principal thus increasing the principal base on which subsequent interest is computed.

The picture given below clearly explains the difference between compound and simple interest.

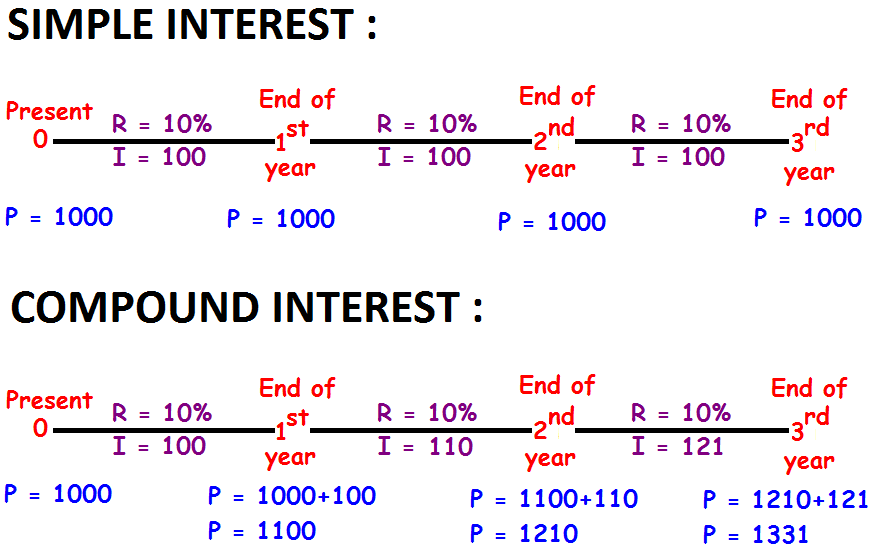
Important Note :

When we look at the above picture, it is clear that interest earned in S.I and C.I is same ($100) for the 1st year when interest is compounded annually in C.I.

The formula given below can be used to find accumulated value in C.I.

A = P(1 + i)ⁿ

A = Accumulated value (final value of an investment)
P = Principal (initial value of an investment)

How to find the value of 'i' :

To find the value of 'i', first convert the rate of interest in to decimal form. Then divide the decimal value by the number of conversion periods per year.

For example, in an investment rate of interest is 15% and compounded quarterly. First we write 15% as decimal form. That is 0.15. Now we have to divide this value by 4 (because compounded quarterly).

Finally, i = 0.15/4 = 0.0375.

How to find the value of 'n' :

n = (no. of years) x (no. of conversion periods per year)

For example, in an investment no. of years = 2 and compounded quarterly. The value of n = 2x4 = 8.

Example 1 :

$800 is invested in compound interest where the rate of interest is 20% per year. If interest is compounded semi annually, what will be the accumulated value and compound interest after 2 years?

Solution :

The formula to find accumulated value in compound interest is

A = P(1 + i)ⁿ

Here,

P = 800

i = 20%/2 = 0.2/2 = 0.1

n = 2 x 2 = 4

Then, the accumulated value is

A = 800(1 + 0.1)⁴

A = 800(1.1)⁴

A = 800 x 1.4641

A = $1171.28

C.I  = A - P 

C.I = 1171.28 - 800

C.I = $371.28

Example 2 :

A sum of money placed at compound interest doubles itself in 3 years. In how many years will it amount to four times itself?

Solution :

Let P be the amount invested initially.

From the given information, P becomes 2P in 3 years.

Since the investment is in compound interest, the principal in the 4th year will be 2P.

And 2P becomes 4P (it doubles itself) in the next 3 years. 

Therefore, at the end of 6 years accumulated value will be 4P.

Hence, the amount deposited will amount to 4 times itself in 6 years.

Example 3 :

The compound interest and simple interest on a certain sum for 2 years is $ 1230 and $ 1200 respectively. The rate of interest is same for both compound interest and simple interest and it is compounded annually. What is the principle?

Solution :

Simple interest for two years  is 1200 and interest for one year is 600.

So, compound interest for 1st year is 600 and for 2nd year is 630.

(Since it is compounded annually, simple interest and compound interest for 1st year would be same)

When we compare the compound interest for 1st year and 2nd year, it is clear that the interest earned in 2nd year is 30 more than the first year.

Because, interest 600 earned in 1st year earned this 30 in 2nd year.

It can be considered as simple interest for one year.

That is, principal = 600, interest = 30.

I = Pit

30 = 600i(1)

0.05 = i

5% = i

In the given problem, simple interest earned in two years is 1200.

I = Pit

1200 = P x 0.05 x 2

1200 = P x  0.1

Divide both sides by 0.1.

1200/0.1 = P

12000 = P

Hence, the principal is $ 12,000.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.