DECIMALS

We have learnt about very big numbers (a number with more number of digits) and fractions which is less than 1. We often use fractions like 1/4, 1/2, 3/4.

By addition or subtraction of fractions, we got fractions like 3/8, 5/8, 7/16.

Very numbers also can be written as fractions. Why can't we use fractions to represent all small numbers ?

It is because of the difficulties in using fractions. 

(2/3) + (3/4) = ?

We have to convert them into like fractions by finding equivalent fractions and then add.

It is easy, if all the fractions are in the form of 1/10, 1/100, 1/1000...

(15/100) + (235/1000) can be easily added as

(150/1000) + (235/1000) = 385/1000

It was easy to use multiples of 10 in measurements. It will be easy if small numbers can be written as fractions with multiples of ten as denominators.  

One Tenths (1/10)

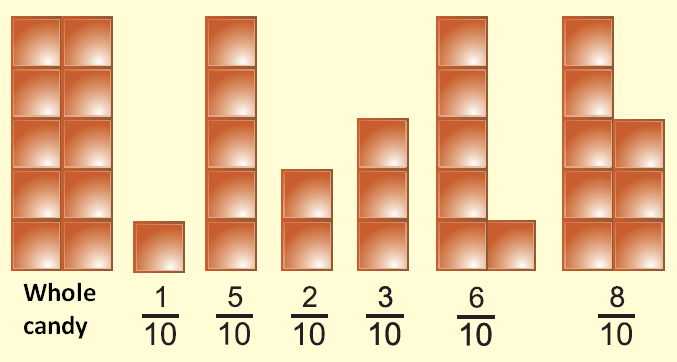
David has 6 candy bars, each connected with 10 connected pieces. 

He gave some pieces to his friends and he finds that 

1 piece out of 10 from the first bar

5 pieces out of 10 from the second bar

2 piece out of 10 from the third bar

3 pieces out of 10 from the fourth bar

6 piece out of 10 from the fifth bar

8 pieces out of 10 from the sixth bar

We can write them as 

1/10, 5/10, 2/10, 3/10, 6/10, 8/10 in fractions. 

This can be written as 0.1, 0.5, 0.2, 0.3, 0.6, 0.8 in decimal numbers. 

0.1 is read as zero point one. The point between between the numbers is called decimal point. 

Definition :

A number in which we have 'point' is called as decimal number. 

A decimal number has two parts namely an integral part and a decimal part. 

Examples :

1)  Consider the decimal number 0.6

0.6 can be written as 0 + 0.6 

integral part = 0, decimal part = 6

2)  Consider the decimal number 7.2.

7.2 can be written as 7 + 0.2

integral part = 7, decimal part = 2

In a decimal number the digits to the left of the decimal point is the integral part. 

The digits to the right of the decimal point is the decimal part. 

The value of all the decimal parts is less than 1. 

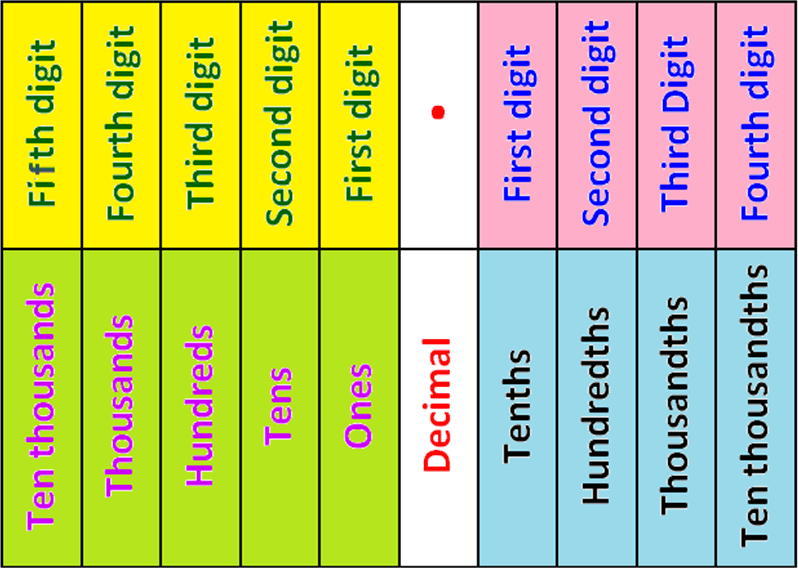
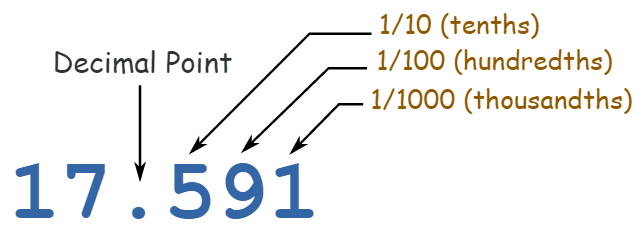
Decimal Place Value

Decimal place value is the one which explains the position of each digit which comes after the decimal point.

Problem 1 :

How many parts of a day is 3 minutes 36 seconds?

a) 1/200    b) 1/300    c) 1/400

Solution :

1 minute = 60 seconds 

3 minutes = 3(60)

= 180 minutes

180 + 36 ==> 216 minutes

In a day, 24 hours = 24 (3600)

= 86400 minutes

= 216/86400

= 1/400

So, option c is correct.

Problem 2 :

0.213 ÷ 0.00213 = ?

a)  0     b)  1      c)  10     d)  100

Solution :

0.213 ÷ 0.00213

= 0.213/0.00213

Since we have 5 digits after the decimal, to convert it as integer we have to multiply by 100000

= (0.213/0.00213) x (100000/100000)

= 21300/213

= 100

So, the answer is option d.

Problem 3 :

3889 + 12.952 - ? = 3854.002

a)  47.095          b)  47.752     c)  47.932     d)  47.95

Solution :

3889 + 12.952 - ? = 3854.002

3901.952 - ? = 3854.002

? = 3901.952 - 3854.002

? = 47.95

So, option d is correct.

Problem 4 :

0.04 x 0.0162 is equal to:

a)  6.48 x 10^-3        b)  6.48 x 10^-4

c)  6.48 x 10^-5         d)  6.48 x 10^-6

Solution :

0.04 x 0.0162

= 0.04 x (100/100) x 0.0162 x (10000/10000) 

= (4 x 162)/(100 x 10000)

= 648/(100 x 10⁴)

= 6.48 x 10^-4

Problem 5 :

Which of the following fractions is the smallest?

a) 11/9    b) 11/7   c) 11/10    d) 11/6

Solution :

Considering the given options, for all we have same numerator. 

Dividing by large numerical values, we will get small value. Then option c is correct.

Problem 6 :

0.7625 lies between

a)  0.7 and 0.76          b)  0.77 and 0.78

c)  0.76 and 0.761       d)  0.76 and 0.763

Solution :

So, option d is correct.  

Problem 7 :

Fill in the blanks using < or >

8/45  ____ 16/89

Solution :

Case 1 :

To compare two fractions in general, we should make the denominators same.

Case 2 :

When numerators are same, comparison can be done more simply.

By observing the fractions, doing this problem in case 2 is easy.

= (8/45) x 2/2

= 16/90

Now the fractions 16/90 and 16/89 are having the same numerators. Then dividing the number by large value, we can make the numerical value smaller.

16/90 < 16/89

8/45  < 16/89

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.