FINDING THE EQUATION OF A LINEAR RELATIONSHIP WORKSHEET

Problem 1 :

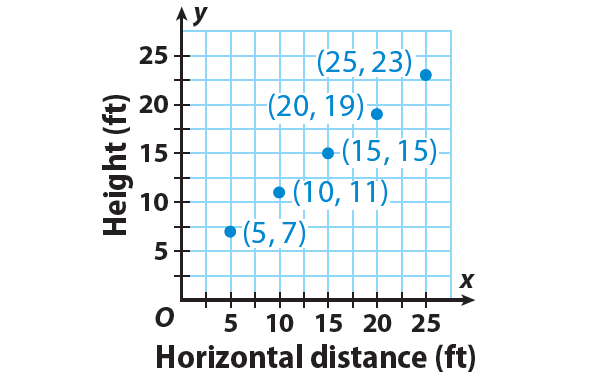
A handrail runs alongside a stairway. As the horizontal distance from the bottom of the stairway changes, the height of the handrail changes. Show that the relationship is linear, and then find the equation for the relationship.

Problem 2 :

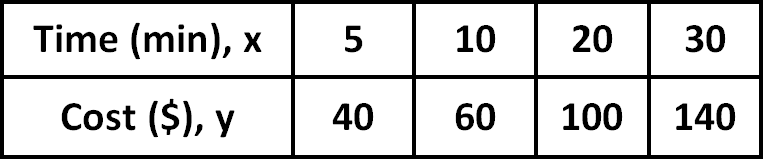
The table shows the relationship between time and cost. Show that the relationship is linear, and then find the equation for the relationship.

Answers

1. Answer :

Step 1 :

Connect the points in the graph given above.

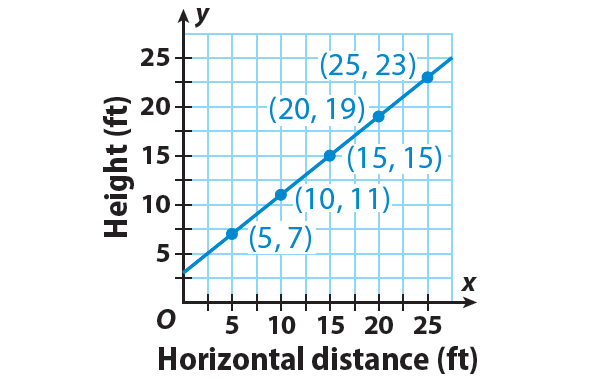
Since all of the points (5, 7), (10, 11), (15, 15), (20, 19), and (25, 23) lie on the same line, the relationship is linear.

Step 2 :

Choose any two points in the form (x, y), from the graph to find the slope :

For example, let us choose (5, 7) and (10, 11).

Use the slope formula. 

m  =  (y₂ - y₁)/(x₂ - x₁)

Substitute (5, 7) for (x₁, y₁) and (10, 11) for (x₂, y₂).

m  =  (11 - 7)/(10 - 5)

m  =  4/5

m  =  0.8

Step 3 : 

Choose a point and use the slope to find the value of "b" (y-intercept b). 

Substitute the point (5, 7) and m = 0.8 in the equation

y  =  mx + b

7  =  (0.8)(5) + b

7  =  4 + b

3  =  b

Step 4 : 

To get the equation of the given linear relationship, substitute m = 0.8 and b = 3 in the equation y = mx + b.

y  =  0.8x + 3

2. Answer :

Step 1 :

From the table, write the points in the form (x, y). 

(5, 40), (10, 60), (20, 100) and (30, 140)

Step 2 :

Plot all the points in a graph paper and connect them. 

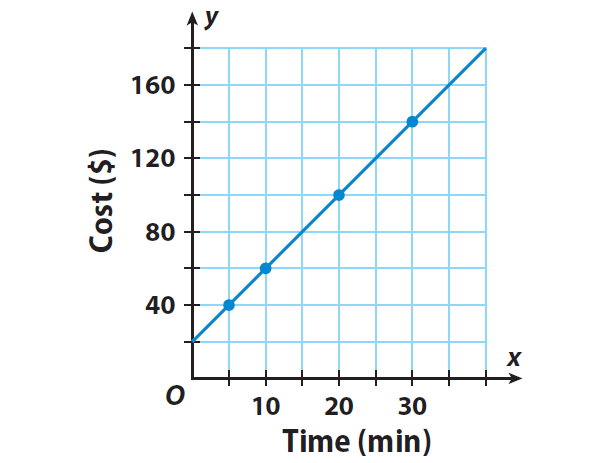
Since all of the points (5, 40), (10, 60), (20, 100) and (30, 140) lie on the same line, the relationship is linear.

Step 3 :

Choose any two points in the form (x, y), from the graph to find the slope :

For example, let us choose (5, 40) and (10, 60).

Use the slope formula. 

m  =  (y₂ - y₁) / (x₂ - x₁)

Substitute (5, 40) for (x₁, y₁) and (10, 60) for (x₂, y₂).

m  =  (60 - 40) / (10 - 5)

m  =  20 / 5

m  =  4

Step 4 : 

Choose a point and use the slope to find the value of "b" (y-intercept b). 

Substitute the point (5, 40) and m = 4 in the equation

y  =  mx + b

40  =  (4)(5) + b

40  =  20 + b

20  =  b

Step 5 : 

To get the equation of the given linear relationship, substitute m = 4 and b = 20 in the equation y = mx + b.

y  =  4x + 20

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.