NATURE OF ROOTS WORKSHEET1

About "Nature of roots worksheet1"

"Nature of roots worksheet1"is nothing but the pdf document which contains questions and answers on the above mentioned stuff.

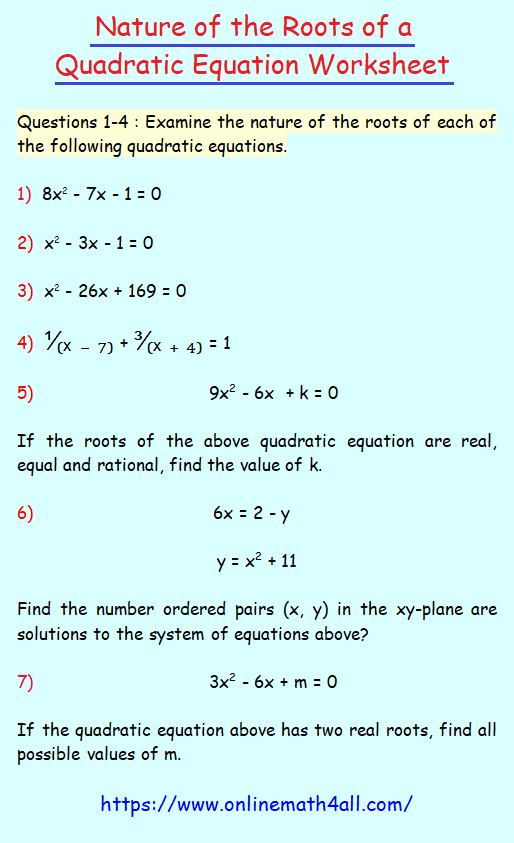
To download "Nature of the roots of a quadratic equation worksheet " as a pdf document,

please click here

If you want to practice the problems in the above pdf document, first you have to know the following stuff.

Now let us look at some examples on "Nature of roots worksheet 1"

Examples

Now, let us see the step by step solutions for the problems you find in "Nature of roots worksheet1".

Example 1 :

Examine the nature of the roots of the following quadratic equation.

x² + 5x + 6 =0

Solution :

If x² + 5x + 6 =0 is compared to the general form ax² + bx + c =0,

we get a = 1, b = 5 and c = 6.

Now, let us find the value of the discriminant "b² - 4ac"

b² - 4ac = 5² - 4(1)(6)

b² - 4ac = 25 - 24

b² - 4ac = 1 (>0 and also a perfect square)

Hence, the roots are real, distinct and rational.

Let us look at the next example on "Nature of roots worksheet1"

Example 2 :

Examine the nature of the roots of the following quadratic equation.

2x² - 3x + 1 =0

Solution :

If 2x² - 3x + 1 =0 is compared to the general form ax² + bx + c =0,

we get a = 2, b = -3 and c = 1.

Now, let us find the value of the discriminant "b² - 4ac"

b² - 4ac = (-3)² - 4(2)(-1)

b² - 4ac = 9 + 8

b² - 4ac = 17 (>0 but not a perfect square)

Hence, the roots are real, distinct and irrational.

Let us look at the next example on "Nature of roots worksheet1"

Example 3 :

Examine the nature of the roots of the following quadratic equation.

x² - 16x + 64 =0

Solution :

If x² - 16x + 64 =0 is compared to the general form ax² + bx + c =0,

we get a = 1, b = -16 and c = 64.

Now, let us find the value of the discriminant "b² - 4ac"

b² - 4ac = (-16)² - 4(1)(64)

b² - 4ac = 256 - 256

b² - 4ac = 0

Hence, the roots are real, equal and rational.

Let us look at the next example on "Nature of roots worksheet1"

Example 4 :

Examine the nature of the roots of the following quadratic equation.

3x² + 5x + 8 =0

Solution :

If 3x² + 5x + 8 =0 is compared to the general form ax² + bx + c =0,

we get a = 3, b = 5 and c = 8.

Now, let us find the value of the discriminant "b² - 4ac"

b² - 4ac = 5² - 4(3)(8)

b² - 4ac = 25- 96

b² - 4ac = -71 (negative)

Hence, the roots are imaginary.

Now we are going to look at some quiet different problems on "Nature of roots worksheet1".

Example 5 :

If the roots of the equation 2x² + 8x - m³ = 0 are equal , then find the value of "m"

Solution :

If 2x² + 8x - m³ =0 is compared to the general form ax² + bx + c =0,

we get a = 2, b = 8 and c = -m³.

Since the roots are equal, we have

b² - 4ac = 0

8² - 4(2)(-m³) = 0

64 + 8m³ = 0

8m³ = -64

m³ = -8

m³ = (-2)³

m = - 2

Hence, the value of "m" is "-2".

Let us look at the next example on "Nature of roots worksheet1"

Example 6 :

If the roots of the equation x² - (p+4)x + 2p + 5 = 0 are equal , then find the value of "p"

Solution :

If x² - (p+4)x + 2p + 5 = 0 is compared to the general form ax² + bx + c =0,

we get a = 1, b = - (p+4) and c = 2p+5

Since the roots are equal, we have

b² - 4ac = 0

[-(p+4)]² - 4(1)(2p+5) = 0

(p+4)² - 8p - 20 = 0

p² + 8p + 16 -8p -20 = 0

p² - 4 =0

p² = 4

p = ± 2

Hence, the value of "p" is " ±2 ".

Let us look at the next example on "Nature of roots worksheet1"

Example 7 :

If the roots of the equation x² + (2p-1)x + p² = 0 are real , then find the value of "p"

Solution :

If x² + (2p-1)x + p² = 0 is compared to the general form ax² + bx + c =0,

we get a = 1, b = 2p-1 and c = p²

Since the roots are real, we have

b² - 4ac ≥ 0

(2p-1)² - 4(1)(p²) ≥ 0

4p² - 4p +1 -4p² ≥ 0

- 4p +1 ≥ 0

1 ≥ 4p (or) 4p ≤ 1

p ≤ 1/4

Hence, the value of "p" is less than or equal to "1/4".

Let us look at the next example on "Nature of roots worksheet1"

Example 8 :

If the roots of the equation x² - 16x + k =0 are real and equal, then find the value of "k"

Solution :

If x² -16x + k = 0 is compared to the general form ax² + bx + c =0,

we get a = 1, b = -16 and c = k

Since the roots are real, we have

b² - 4ac = 0

(-16)² - 4(1)(k) = 0

64 - 4k = 0

64 = 4k

16 = k

(or) k = 4

Hence, the value of "k" is "4".

Let us look at the next example on "Nature of roots worksheet1"

Example 9 :

Examine the nature of the roots of the following quadratic equation.

x² - 5x = 2(3x+1)

Solution :

First, let us write the given equation in general form.

x² - 5x = 2(3x+1)

x² - 5x = 6x+2

x² - 11x -2 = 0

If x² -11x - 2 = 0 is compared to the general form ax² + bx + c =0,

we get a = 1, b = -11 and c = -2

Now, let us find the value of the discriminant "b² - 4ac"

b² - 4ac = (-11)² - 4(1)(-2)

b² - 4ac = 121 + 8

b² - 4ac = 129 (>0, but not a perfect square)

Hence, the roots are real, distinct and irrational

Let us look at the next example on "Nature of roots worksheet1"

Example 10 :

Examine the nature of the roots of the following quadratic equation. nature of the roots of quadratic equations worksheet pdf

Solution :

If 2x² - 9x -6 = 0 is compared to the general form ax² + bx + c =0,

we get a = 2, b = -9 and c = -6.

Now, let us find the value of the discriminant "b² - 4ac"

b² - 4ac = (-9)² - 4(2)(-6)

b² - 4ac = 81+ 48

b² - 4ac = 129 (>0, but not a perfect square)

Hence, the roots are real, distinct and irrational.

We hope that the student would have understood the problems and solutions given on "nature of roots worksheet1"

Apart from "nature of roots worksheet1", you can also visit the following web pages to know more about quadratic equation. Because learning the below stuff would be much helpful for you to do problems on "nature of roots worksheet1".

2. Relationship between zeros and coefficients of a quadratic polynomial

3. Word problems on quadratic equation

4. Quadratic equation online solver

Apart from the problems given on "nature of roots worksheet", if you would like to have more problems on "nature of roots worksheet1", please click here.

HTML Comment Box is loading comments...