PLAYING CARDS PROBABILITY

A pack of 52 playing cards always plays a key role in probability concept. Whenever we go through the stuff probability in statistics, we will definitely have examples with a well shuffled pack of 52 playing cards. 

Basic Stuff

Before we enter in to the stuff probability, we must be aware of the pack of 52 playing cards. 

There are four types of cards in the pack of 52 cards. Two types in black color and other two types in red color. 

The four different types of cards are shown in the picture given below. 

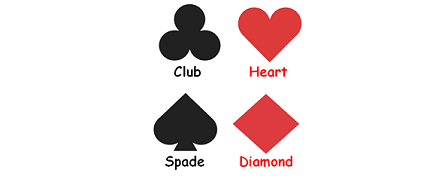
In the above four types, we will have 13 cards in each type.

It is shown in the picture given below.

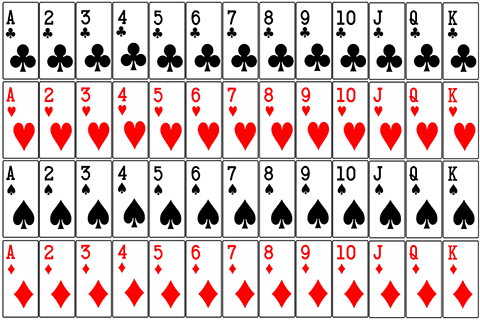
Let us come to know the following stuff related the pack of 52 playing cards. 

1.  Club  -  13 cards

2.  Heart  -  13 cards

3.  Spade  -  13 cards

4.  Diamond  -  13 cards

5.  No. of black cards  -  26

6.  No. of red cards  -  26

7.  No. of Ace cards (named as "A")  -  4

8.  No. of Jack cards (named as "J"  -  4

9.  No. of Queen cards (named as "Q")  -  4

10.  No. of King cards (named as "K")  -  4

11. No. of face cards (named as "J", "Q" and "K")  -  12

(In each type, there three face cards. So there are 12 cards in total of all the four types)

Probability Formula

We can use the formula from classic definition to find probability with playing cards. 

or

Because there are 52 playing cards in total,

n(S) = 52

Solved Problems

Problem 1 :

A card is drawn at random from a well shuffled pack of 52 cards. What is the probability that the drawn card is king ?

Solution :

Let A be the event of drawing a king card. 

There are 4 king cards in total. 

Then, 

n(A)  =  4

And also

n(S)  =  52

Required probability is

P(A)  =  n(A) / n(S)

P(A)  =  4/52  =  1/13

So, the probability of getting a kind card is 1/13.

Problem 2 :

A card is drawn at random from a well shuffled pack of 52 cards. Find the probability that the drawn card is not king.

Solution :

Let A be the event of drawing a card that is not king. 

There are 4 king cards in the pack of 52 cards.

Then, the number of cards which are not king :

n(A)  =  52 - 4

n(A)  =  48

And also

n(S)  =  52

Required probability is

P(A)  =  n(A) / n(S)

P(A)  =  48/52

P(A)  =  12/13

So, the probability of getting a kind card is 12/13.

Problem 3 :

A card is drawn at random from a well shuffled pack of 52 cards. What is the probability that the card is neither a spade nor a Jack ?

Solution :

Let A be the event of drawing a card that is neither spade nor Jack. 

There are 13 spade cards in a pack of 52 cards. 

(Jack in spade is already included in these 13 cards)

In the remaining 3 types (club, heart, diamond), there are 3 jack cards. 

Number of spade cards and jack cards is

=  13 + 3

=  16 

So, no.of cards which are neither spade nor Jack  are

=  52 - 16

=  36

Then,

n(A)  =  36

And also

n(S)  =  52

Required probability is

P(A)  =  n(A) / n(S)

P(A)  =  36/52

P(A)  =  9/13

So, the required probability is 9/13.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.