RISE OVER RUN FORMULA

The formula for slope is referred to rise over run,

Because the fraction consists of the rise (the change in y, going up or down) divided by the run (the change in x, going from left to the right).

The pictures shown below illustrate this.

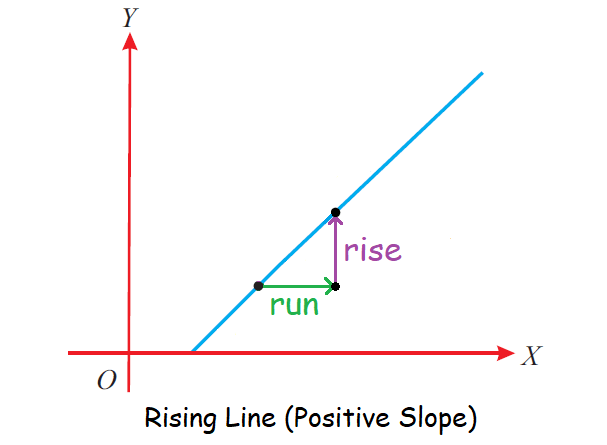
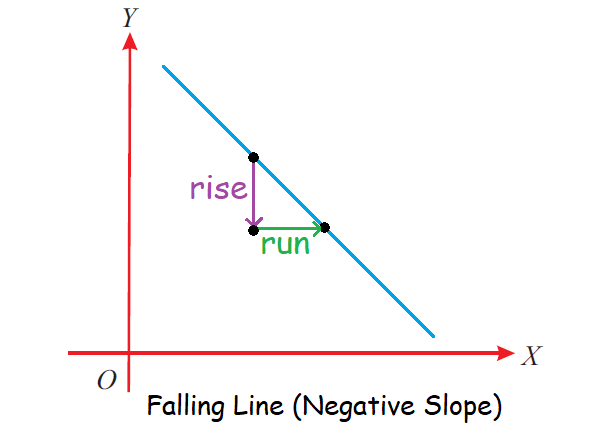
The simplest way to look at the slope is

ʳⁱˢᵉ⁄ᵣᵤₙ

(rise over run)

In the formula (ʳⁱˢᵉ⁄ᵣᵤₙ), we can "rise" up or down... but, we ALWAYS "run" to the right.

If the "rise" is up, it will have a positive value and if the "rise" is down, it will have a negative value.

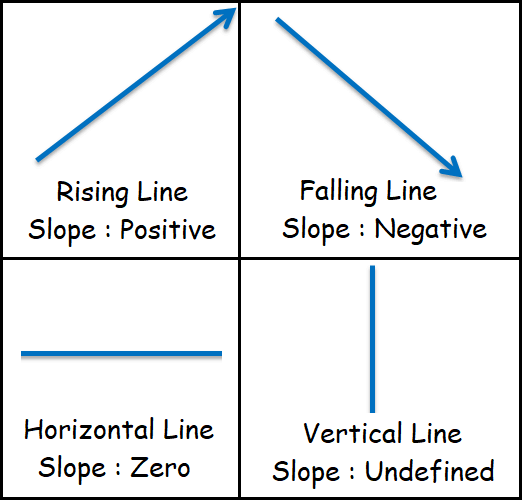
Slope of a Line - Positive or Negative or Zero or Undefined

To know the sign of the slope of a straight line, always we have to look at the straight line from left to right.

(i) If the line is going (from left to right) towards up, then the line is called rising line and its slope will be a positive value.

(ii) If the line is going (from left to right) towards down, then the line is called falling line and its slope will be a negative value.

(iii) If the line is horizontal, the slope will be zero.

(iv) If the line is vertical, the slope will be undefined.

Solved Examples

Find the slope of each of the following lines using rise over run formula.

Example 1 :

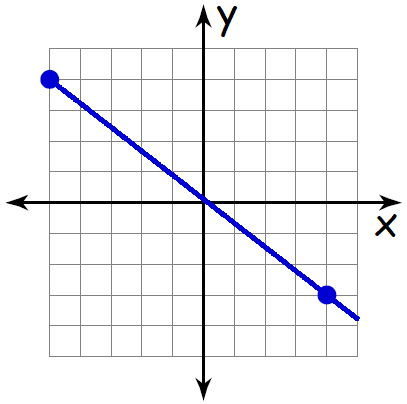
Solution :

The above line is a falling line. So, its slope will be a negative value.

Measure the rise and run.

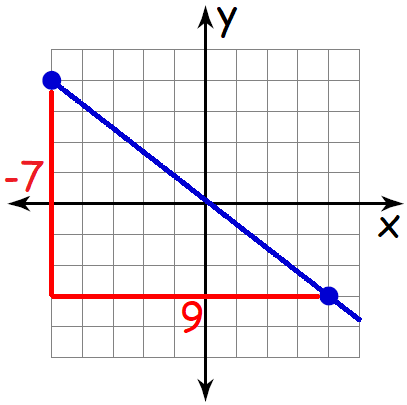
For the above line,

rise = -7

run = 9

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= -⁷⁄₉

Example 2 :

Find the slope of the line shown below using rise over run formula.

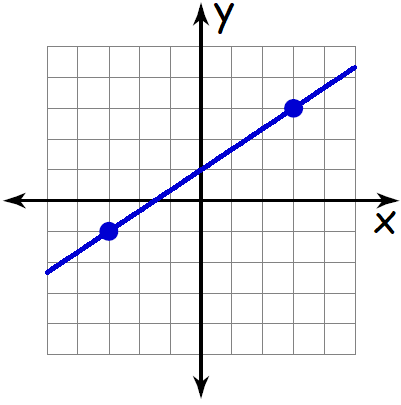
Solution :

The above line is a rising line. So, its slope will be a positive value.

Measure the rise and run.

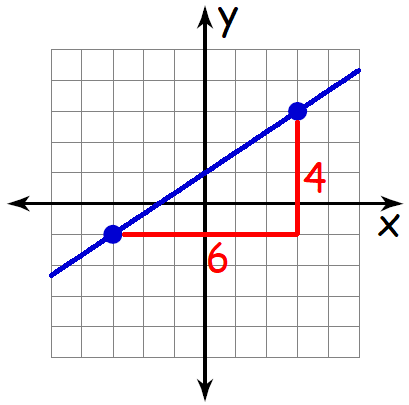
For the above line,

rise = 4

run = 6

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= ⁴⁄₆

= ⅔

Example 3 :

Find the slope of the line shown below using rise over run formula.  

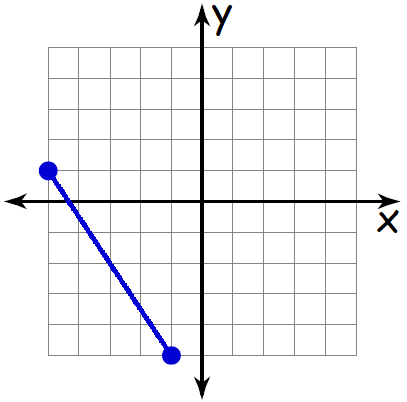
Solution :

The above line is a falling line. So, its slope will be a negative value.

Measure the rise and run. 

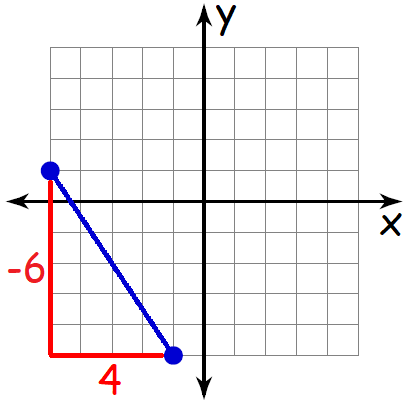
For the above line,

rise = -6

run = 4

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= -⁶⁄₄

= -³⁄₂

Example 4 :

Find the slope of the line shown below using rise over run formula.

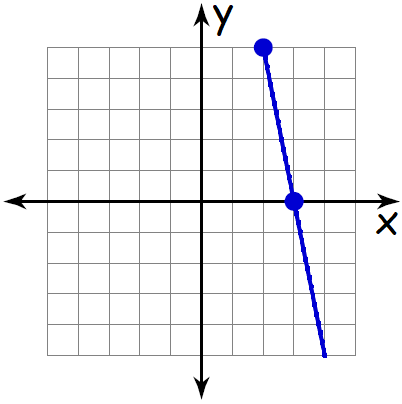
Solution :

The above line is a falling line. So, its slope will be a negative value.

Measure the rise and run.

For the above line,

rise = -5

run = 1

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= -⁵⁄₁

= -5

Example 5 :

Find the slope of the line shown below using rise over run formula.

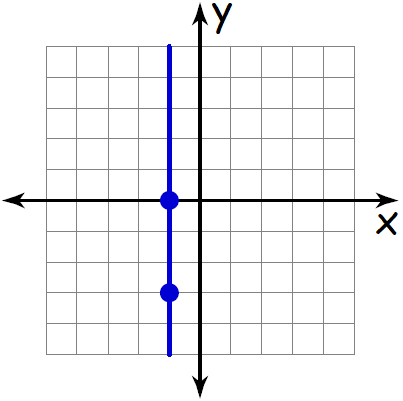
Solution :

The above line is a vertical line.

Measure the rise and run.

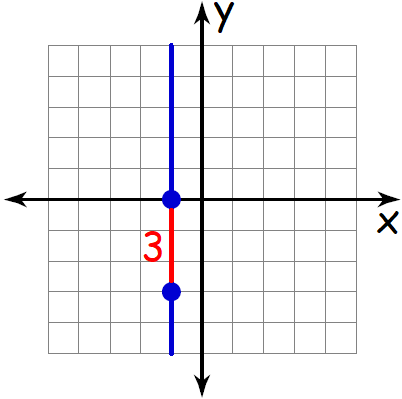
For the above line,

rise = 3

run = 0

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= ³⁄₀

= Undefined

Note :

The slope of a vertical line is always undefined.

Example 6 :

Find the slope of the line shown below using rise over run formula.

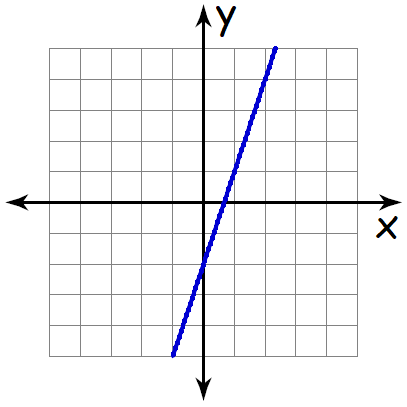
Solution :

The above line is a rising line. So, its slope will be a positive value.

Measure the rise and run.

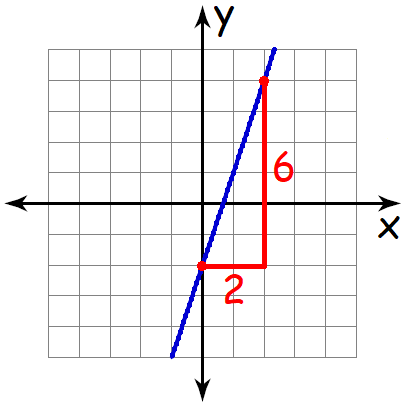
For the above line,

rise = 6

run = 2

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= ⁶⁄₂

= 3

Example 7 :

Find the slope of the line shown below using rise over run formula.

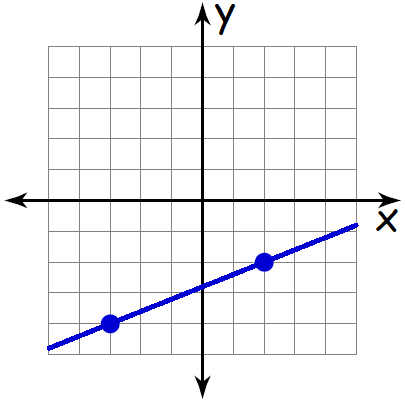
Solution :

The above line is a rising line. So, its slope will be a positive value.

Measure the rise and run.

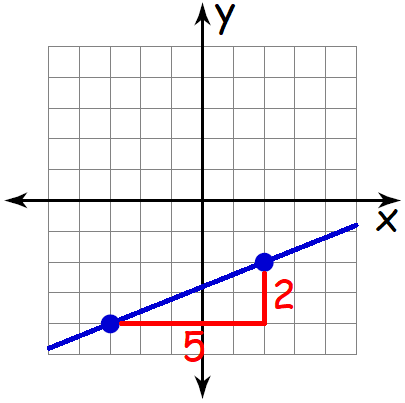
For the above line,

rise = 2

run = 5

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= ⅖

Example 8 :

Find the slope of the line shown below using rise over run formula.

Solution :

The above line is an horizontal line.

Measure the rise and run.

For the above line,

rise = 0

run = 4

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

= ⁰⁄₄

= 0

Note :

The slope of an horizontal line is always zero.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.