PSAT MATH QUESTIONS PRACTICE

Question 1 :

Given that 0 < x < 1, and set A = {x, x², x³, x⁴}, what is the smallest value in set A ?

(A)  x  (B)  x²  (C)  x³  (D)  x⁴ 

 (E)  cannot be determined 

Solution :

Let  x = 0.5

x²  =  0.25

x³  =  0.125

x⁴  =  0.0625

When the power is being increased, the answer get decreased. Hence the smallest value of the given set is x⁴

Question 2 :

Sammy has a faulty clock. Every 15 degrees that one of the hands moves, 5 minutes passes. If a hand is initially 5 : 35 PM, in how long will be the hand be at that same position ?

(A)  65  minutes  (B)  2 hours  (C)  1 hour 

(D)  45 minutes   (E)  1 hour and 15 minutes

Solution :

There are 360 degree that the hands must move to complete a full revolution and to be at the same time of 5 :35, if every 15 degree, 5 minutes passes, then (24 ⋅5) minutes will have passed by the time it is 5 : 35 again. This is 2 hours. 

Question 3 :

If (x - 2) (x + 2)  =  ax² + bx + c, what is the sum of a, b and c ?

(A)  -4  (B)  -3  (C)  0  (D)  1  (E)  5

Solution :

ax² + bx + c  =  (x - 2)(x + 2)

  =  x² - 2x + 2x - 4

  =  x² - 0x - 4

a = 1, b = 0 and c = -4

a + b + c = 1 + 0 + (-4)

a + b + c  =  -3

Question 4 :

Natalie walks in a special way. After every 2 steps she takes, she takes 1 step in the opposite direction. She starts at point A and walks forward. When she is 7 steps away from the point A, she has reached her destination point B. How many steps in total did she take to get from point A to B.

(A)  7  (B)  8  (C)  17  (D)  15  (E)  18

Solution :

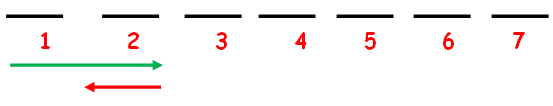
When she takes two steps forward, she has to go 1 step back ward.

From the picture given above, she has taken 3 steps.

Question 5 :

If Cmn  =  C(m + n), for what value of n is Cmn neither positive nor negative ?

(A)  -C  (B)  -m  (C)  2m  (D)  0  (E)  1/m

Solution :

We must pick value of n that makes the expression O, since O neither positive nor negative. This occurs when n is the negative of m.

Hence the value of n is -m.

Question 6 :

Two sides of a triangle at 6 and 8. What is the length of the third side ?

(A)  2  (B)  4  (C)  5  (D)  10  (E)  Cannot be determined

Solution :

The sum of length of 2 sides will be greater than the other side. But we could not say the exact length of third side.

Hence, we cannot determine.

Question 7 :

2 + (1/3)  =  14/b, what is b ?

(A)  3  (B)  6  (C)  7  (D)  9  (E)  28

Solution :

2 + (1/3)  =  14/b

(6 + 1)/3  =  14/b

7/3  =  14/b

b  =  14 (3)/7

b  =  6

Question 8 :

The radius of circle A is x and the radius of circle B is 2. If the circumference of circle A is two times the circumference of the circle B, find the value of x.

(A)  1  (B)  2  (C)  3  (D)  4  (E)  5

Solution :

2πx  =  2[2π(2)]

2πx  =  2(4π)

2πx  =  8π

Divide each side by 2π. 

x  =  4

Question 9 :

In the formula V = r²h, if h is doubled and r is tripled, then V is multiplied by ?

(A)  6  (B)  9  (C)  12  (D)  18  (E)  36

Solution :

h = 2h, r = 3r

V = r²h

V = (3r)²(2h)

V = 9r²(2h)

V = 18r²h

Question 10 :

Max A returns the largest value in the set A, min A returns the lowest value in the set A. For example, max {1, 2, 3} = 3 and min {0, 4, 5} = 0. What is max {min{x, 2x, 3x}, max{x/2, x/4,x/8}} ?

(A)  x   (B)  2x  (C)  x/2  (D)  3x

  (E)  cannot be determined.

Solution :

The answer cannot be uniquely determined, since we do not know if x is negative or positive.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.