SHSAT MATH PRACTICE FOR 9TH GRADERS

Question 1 :

Five apples and 6 bananas together cost as much as 8 bananas and 9 pears.  One apple costs as much as 2 pears.  For the same price as 1 pear, how many bananas could be bought?

(A)  1  (B)  2  (C)  3  (D)  4  (E)  6

Solution :

Let "x", "y" and "z" be the cost pf one apple, one banana and one pears.

5x + 6y =  8y + 9z

5x + 6y - 8y  =  9z

  5x - 2y  =  9z  ---(1)

Given that : 

One apple costs as much as 2 pears. 

x  =  2z

Applying the value of x in (1), we get

5(2z) - 2y  =  9z

10z - 9z  =  2y

z  =  2y

Cost of 1 pear is equal too 2 bananas.

Question 2 :

Let R = 8 · 9 · 7 · 13 and S = 4 · 6 · 11 · 49.  The greatest common factor of R and S is

(A)  12  (B)  42  (C)  56  (D)  168  (E)  504

Solution :

R = 8 · 9 · 7 · 13 and S = 4 · 6 · 11 · 49

R = 2³ · 3² · 7 · 13, S = 2³ · 3 · 11 · 7²

Greatest common factor of R and S  =  2³ · 3 · 7

  =  168

Hence the required GCF is 168.

Question 3 :

What is the value of 4|x – y| - 3|xy| if x = -5 and y = 2?

(A)  -42  (B)  -18  (C)  -2  (D)  42  (E)  58

Solution :

  =   4|x – y| - 3|xy| 

  =   4|-5 – 2| - 3|(-5)(2)| 

  =   4|-7| - 3|(-10)| 

  =   4(7) - 3(10)

  =  28 - 30

  =  -2

Question 4 :

In the following sequence of eight numbers, each number has one more “1” than the number before it:

2, 12, 112, 1112,. . . . , 11111112

What is the hundreds digit in the sum of all eight of these numbers?

(A)  0  (B)  6  (C)  7  (D)  8  (E)  9

Solution :

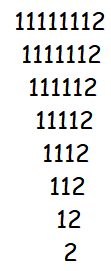
In 8th column, we have 8 two's. By adding these two's we get 16.

We write 6 in the unit place and carry 1.

In ten's place, we have 7 one's. So we have to write 7 + 1  =  8.

In hundreds place, we have six 1's.So the required answer is 6.

Question 5 :

In Ms. Hsiao’s class there are twice as many boys as there are girls.  Half of the boys are in the math club and all of the girls are in the math club.  What percent of Ms. Hsiao’s class is in the math club?

(A)  25%  (B)  33  (1/3)%  (C)  50%

(D)  66 (2/3)%  (E)  75%

Solution :

Let "x" be the number of girls

"2x" be the number of boys.

Total number of students = x + 2x  =  3x

Number of boys in math club  =  (1/2) 2x  =  x

Number of students in math club  =  x + x  =  2x

Percentage of students in math club 

  =  (2x/3x)⋅100%

  =  200/3

  =  66  2/3 %

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.