ADDITIVE INVERSE OF A RATIONAL NUMBER

The opposite, or additive inverse, of a number is the same distance from 0 on a number line as the original number, but on the other side of 0. Zero is its own additive inverse.

In other words, the additive inverse of a rational number is the same number with opposite sign. 

For example : Additive inverse of 2/3 is -2/3. 

Practice Problems

Problem 1 : 

A football team loses 3.5 yards on their first play. On the next play, they gain 3.5 yards. What is the overall increase or decrease in yards?

Solution : 

Step 1 : 

Use a positive number to represent the gain in yards and a negative number to represent the loss in yards.

Step 2 : 

Find -3.5 + 3.5.

Step 3 : 

Start at -3.5.

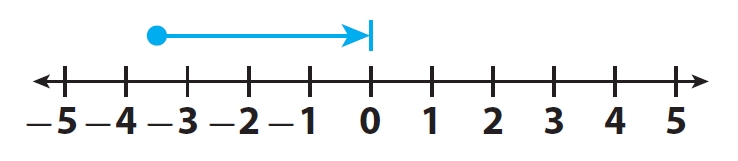
Step 4 : 

Move | 3.5 | = 3.5 units to the right, because the second addend is positive.

The result is 0. This means the overall change is 0 yards.

Problem 2 : 

Add the rational number 2/3 and its additive inverse.

Solution : 

Step 1 : 

The additive inverse of 2/3 is -2/3. 

Then, we have to find (2/3) + (-2/3). 

Step 2 : 

In the above addition, since the two rational numbers have different signs, we have to find the absolute difference of them without the actual signs. 

|2/3 - 2/3|  =  |0|  =  0

So, 

(2/3) + (-2/3)  =  0

Problem 3 : 

Add the rational number 4/9 and the additive inverse of 2/9.

Solution : 

Step 1 : 

The additive inverse of 2/9 is -2/9. 

Then, we have to find (4/9) + (-2/9). 

Step 2 : 

In the above addition, since the two rational numbers have different signs, we have to find the absolute difference of them without the actual signs. 

|4/9 - 2/9|  =  |2/9|  =  2/9

Step 3 : 

In (4/9) + (-2/9), the sign of the bigger number is positive. So, we have to take positive sign to the answer. 

So,

(+4/9) + (-2/9)  =  +2/9

Problem 4 : 

Add the rational number -4/3 and the additive inverse of -1/3.

Solution : 

Step 1 : 

The additive inverse of -1/3 is +1/3. 

Then, we have to find (-4/3) + (+1/3). 

Step 2 : 

In the above addition, since the two rational numbers have different signs, we have to find the absolute difference of them without the actual signs. 

|4/3 - 1/3|  =  |3/3|  =  1

Step 3 : 

In (-4/3) + (+1/3), the sign of the bigger number is negative. So, we have to take negative sign to the answer. 

So,

(-4/3) + (+1/3)  =  -1

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.