ADJACENT ANGLES

Two angles are adjacent if they have a common vertex and a common side.

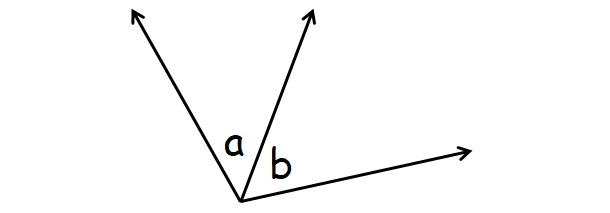
In the diagram below, the two angles a and b are adjacent.

Solved Examples

Examples 1-2 : Identify the two pairs of adjacent angles in the given figure.

Example 1 :

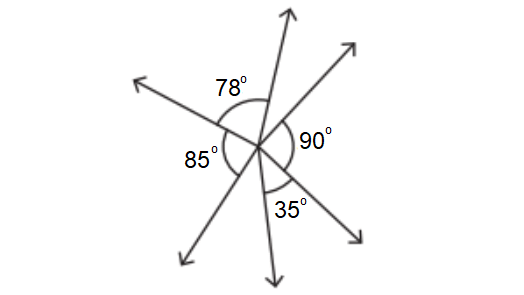
Solution :

78° and 85°

35° and 90°

Example 2 :

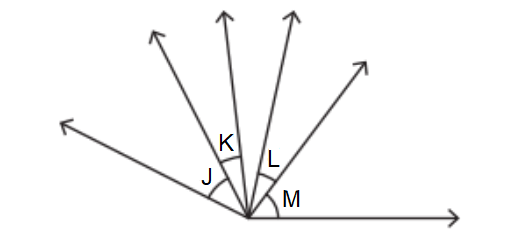
Solution :

∠J and ∠K

∠L and ∠M

Example 3 :

In the figure given below, if ∠ABC is a right angle, find the value of x.

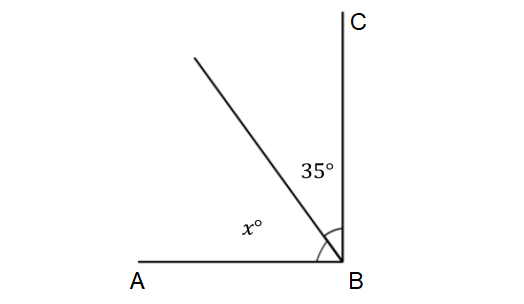
Solution :

In the figure above, x° and 35° are adjacent angles and they add up to m∠ABC.

x° + 35° = m∠ABC

Since ∠ABC is a right angle, substitute m∠ABC = 90°.

x° + 35° = 90°

Subtract 35° from both sides.

(x° + 35°) - 35° = 90° - 35°

x° + 35° - 35° = 55°

x° + 0° = 55°

x° = 55° 

The value of x is 65.

Example 4 :

In the figure given below, if ∠XYZ is a right angle, find the value of x.

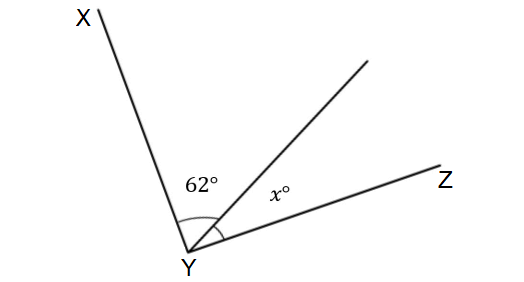
Solution :

In the figure above, x° and 62° are adjacent angles and they add up to m∠XYZ.

x° + 62° = m∠XYZ

Since ∠XYZ is a right angle, substitute m∠XYZ = 90°.

x° + 62° = 90°

Subtract 62° from both sides.

(x° + 62°) - 62° = 90° - 62°

x° + 62° - 62° = 28°

x° + 0° = 28°

x° = 28° 

The value of x is 28.

Example 5 :

In the figure given below, if ∠PQR is a straight angle, find the value of x.

Solution :

In the figure above, 145° and x° are adjacent angles and they add up to m∠PQR.

145° + x° = m∠PQR

Since ∠PQR is a straight angle, substitute m∠PQR = 180°.

145° + x° = 180°

Subtract 145° from both sides.

(145° + x°) - 145° = 180° - 145°

145° + x° - 145° = 35°

x° = 35°

The value of x is 35.

Example 6 :

In the figure given below, if ∠JKL is a straight angle, find the value of x.

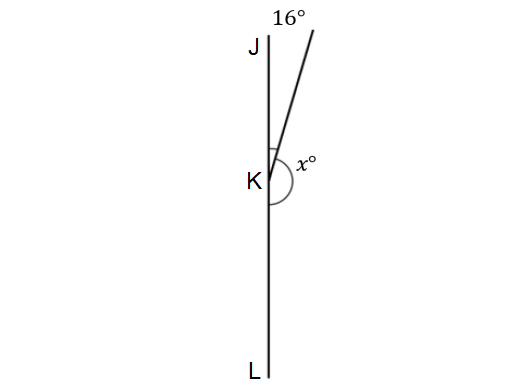
Solution :

In the figure above, x° and 16° are adjacent angles and they add up to m∠JKL.

x° + 16° = m∠JKL

Since ∠JKL is a straight angle, substitute m∠JKL = 180°.

x° + 16° = 180°

Subtract 16° from both sides.

(x° + 16°) - 16° = 180° - 16°

x° + 16° - 16° = 164°

x° + 0° = 164°

The value of x is 164.

Example 7 :

In the figure given below, if ∠ABC is a straight angle, find the value of x.

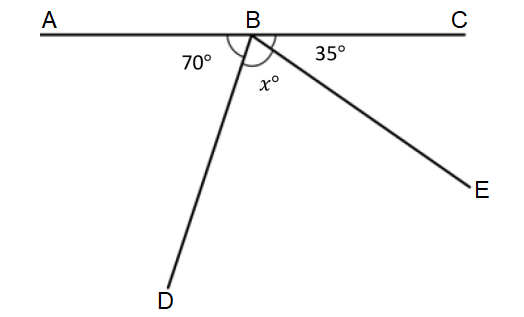
Solution :

In the figure above, ∠ABD and ∠DBC are adjacent angles and they add up to m∠ABC.

m∠ABD + m∠DBC = m∠ABC

Since ∠ABC is a straight angle, substitute m∠ABC = 180°.

m∠ABD + m∠DBC = 180°

m∠ABD + m∠DBE + m∠EBC = 180°

Substitute m∠ABD = 70°, m∠DBE = x° and m∠EBC = 35°.

70° + x° + 35° = 180°

x° + 105° = 180°

Subtract 105° from both sides.

(x° + 105°) - 105° = 180° - 105°

x° + 105° - 105° = 75°

x° + 0° = 75°

x° = 75°

The value of x is 75.

Example 8 :

In the figure given below, if ∠PQR is a straight angle, find the value of x.

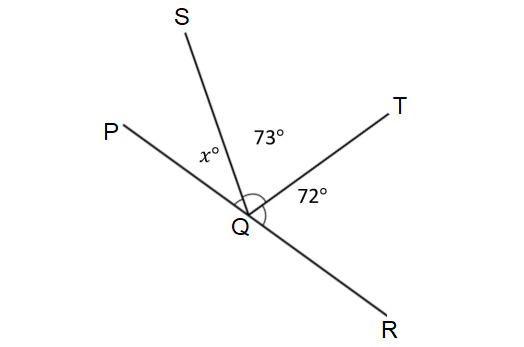
Solution :

In the figure above, ∠PQT and ∠TQR are adjacent angles and they add up to m∠PQR.

m∠PQT + m∠TQR = m∠PQR

Since ∠PQR is a straight angle, substitute m∠PQR = 180°.

m∠PQT + m∠TQR = 180°

m∠PQS + m∠SQT + m∠TQR = 180°

Substitute m∠PQS = x°, m∠SQT = 73° and m∠TQR = 72°.

x° + 73° + 72° = 180°

x° + 145° = 180°

Subtract 145° from both sides.

(x° + 145°) - 145° = 180° - 145°

x° + 145° - 145° = 35°

x° + 0° = 35°

x° = 35°

The value of x is 35.

Example 9 :

In the figure shown below, PQST is a rectangle. Without using a protractor, determine m∠SQT.

Solution :

In the figure above, ∠PQS and ∠SQT are adjacent angles and they add up to m∠PQT.

m∠PQS + m∠SQT = m∠PQT

Since PQST is a rectangle, each interior angle is a right angle. Then, m∠PQT = 90°.

m∠PQS + m∠SQT = 90°

Substitute m∠PQS = 74°.

74° + m∠SQT = 90°

Subtract 74° from both sides.

74° + m∠SQT - 74° = 90° - 74°

m∠SQT = 16°

Example 10 :

Two adjacent angles are complementary. If the second angle is 10° more than three times the first angle, find the two angles.  

Solution :

Let x° be the first angle.

Then, the second angle is  (3x° + 10°).

Since the two adjacent angles are complementary,

x° + (3x° + 10°) = 90°

x + 3x + 10 = 90

4x + 10 = 90

Subtract 10 from both sides.  

(4x + 10) - 10 = 90 - 10

4x + 10 - 10 = 80

4x + 0 = 80

4x = 80

Divide both sides by 4.

x = 20

3x + 10 = 3(20) + 10

= 60 + 10

= 70

Therefore, the required angles are 20° and 70°.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.