ANALYZING BOX PLOTS

Box plots show five key values to represent a set of data, the least and greatest values, the lower and upper quartile, and the median.

To create a box plot, arrange the data in order, and divide them into four equal-size parts or quarters.

Then draw the box and the whiskers. 

Example 1 :

The number of points a high school basketball player scored during the games he played this season are organized in the box plot shown.

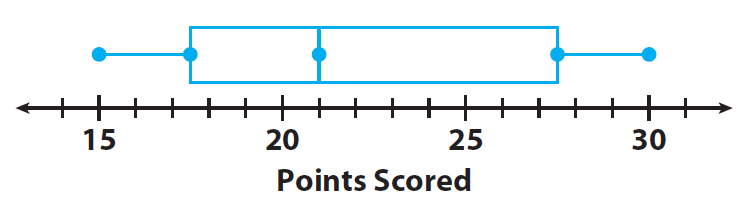
1.  Find the least and greatest values.

Least value : 15 points ;

Greatest value : 30 points

2.  Find the median and describe what it means for the data.

Median : 21 points; the median, or second quartile,

Q2, divides the data values into two halves, a lower half and an upper half.

3.  Find and describe the lower and upper quartiles.

The lower quartile, Q1, is the median of the lower half. The upper quartile, Q3, is the median of the upper half. Q1 : 17.5 and Q3 : 27.5

4.  The inter quartile range is the difference between the upper and lower quartiles, which is represented by the length of the box. Find the interquartile range.

Q3 - Q1  =  27.5 - 17.5

Q3 - Q1  =  10 points.

5.  Why is one-half of the box wider than the other half of the box ?

The quarter of data to the right of the median is more spread out than the quarter of data to the left of the median.

Example 2 :

Maths test scores of a student in several tests are organized in the box plot shown.

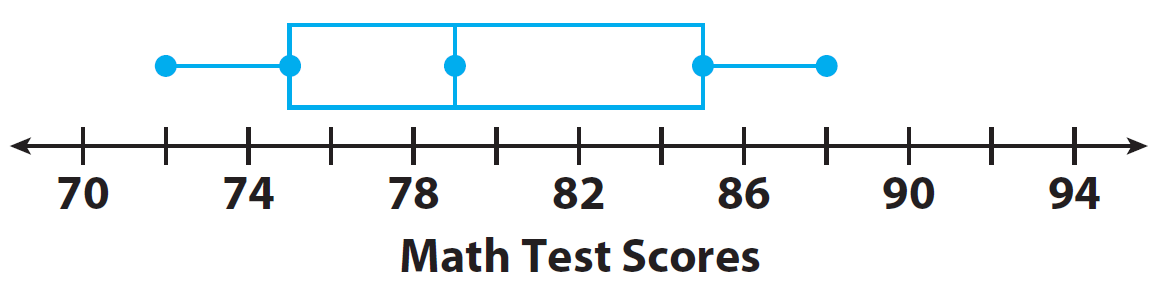
1.  Find the minimum and maximum scores.

Minimum score : 72 ;

Maximum score : 88

2.  Find the range.

Range  =  88 - 72

Range  =  16

3.  Find the median and describe what it means for the data.

Median : 79 ; the median, or second quartile,

Q2, divides the data values into two halves, a lower half and an upper half.

4.  Find and describe the lower and upper quartiles.

The lower quartile, Q1, is the median of the lower half. The upper quartile, Q3, is the median of the upper half. Q1 : 75 and Q3 : 85

5.  The inter quartile range is the difference between the upper and lower quartiles, which is represented by the length of the box. Find the interquartile range.

Q3 - Q1  =  85 - 75

Q3 - Q1  =  10

6.  Why is one-half of the box wider than the other half of the box ?

The quarter of data to the right of the median is more spread out than the quarter of data to the left of the median.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.