ANGLE OF ELEVATION AND DEPRESSION WORD PROBLEMS WORKSHEET

(1)  From the top of a tree of height 13 m the angle of elevation and depression of the top and bottom of another tree are 45° and 30° respectively. Find the height of the second tree. (√3 = 1.732)       Solution 

(2)  A man is standing on the deck of a ship, which is 40 m above water level. He observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of the hill as 30° . Calculate the distance of the hill from the ship and the height of the hill. (√3 = 1.732)

Solution 

(3)  If the angle of elevation of a cloud from a point ‘h’ meters above a lake is θ₁ and the angle of depression of its reflection in the lake is θ₂ . Prove that the height that the cloud is located from the ground is h(tan θ₁+ tan θ₂)/(tan θ₂ - tan θ₁)             Solution 

(4)  The angle of elevation of the top of a cell phone tower from the foot of a high apartment is 60° and the angle of depression of the foot of the tower from the top of the apartment is 30° . If the height of the apartment is 50 m, find the height of the cell phone tower. According to radiations control norms, the minimum height of a cell phone tower should be 120 m. State if the height of the above mentioned cell phone tower meets the radiation norms.            Solution

(5)  The angles of elevation and depression of the top and bottom of a lamp post from the top of a 66 m high apartment are 60° and 30° respectively. Find (i) The height of the lamp post. (ii) The difference between height of the lamp post and the apartment. (iii) The distance between the lamp post and the apartment. (√3 = 1.732)          Solution

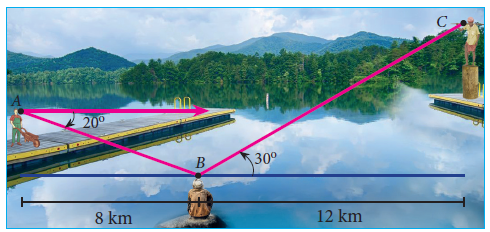
(6)  Three villagers A, B and C can see each other across a valley. The horizontal distance between A and B is 8 km and the horizontal distance between B and C is 12 km. The angle of depression of B from A is 20° and the angle of elevation of C from B is 30° . Calculate : (i) the vertical height between A and B. (ii) the vertical height between B and C. (tan 20° = 0.3640, √3 = 1.732)]

Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.