ANGLE OF ELEVATION PRACTICE PROBLEMS
The angle of elevation is an angle formed by the line of sight with the horizontal when the point being viewed is above the horizontal level. That is, the case when we raise our head to look at the object.
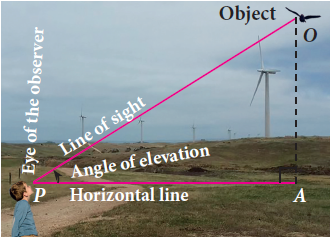
In order to solve word problems, first draw the picture to represent the given situation.
Now, decide what we have to find from the given picture.
Mark the sides as opposite, hypotenuse and adjacent based on theta.
Now we have to choose a trigonometric ratio sinθ, cos θ or tanθ based on the information that we have and the thing we have to find.
For example, if we have opposite side and we have to find the length of hypotenuse then we have to choose sin θ.
Problem 1 :
Find the angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of a tower of height 10√3 m.
Solution :
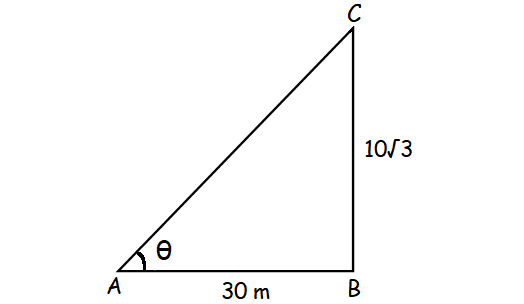
BC - height of the tower
∠B = 90°
AC = hypotenuse side, BC = opposite side, AB = Adjacent side
Here we have to find θ, known sides are opposite and adjacent. Based on this information, we have to use tan θ.
tan θ = Opposite side / Adjacent side
tan θ = BC/AB
tan θ = 10√3/30
tan θ = √3/3
tan θ = 1/√3
θ = tan-1(1/√3)
θ = tan-1(1/√3)
θ = 30° or π/6
Problem 2 :
A road is flanked on either side by continuous rows of houses of height 4√3 m with no space in between them. A pedestrian is standing on the median of the road facing a row house. The angle of elevation from the pedestrian to the top of the house is 30° . Find the width of the road.
Solution :
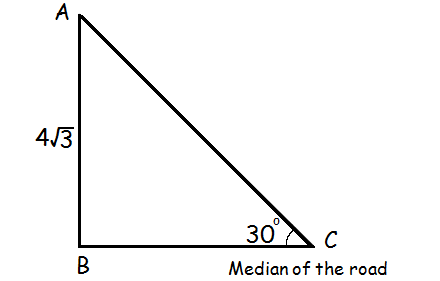
AB = opposite side, BC = Adjacent side, AC = hypotenuse side
tan θ = Opposite side / Adjacent side
tan 30 = AB/BC
1/√3 = 4√3/Distance from median of the road to house
BC = 4√3(√3) = 12
Width of the road :
= 2(BC)
= 2(12)
= 24 m
Problem 3 :
A tower stands vertically on the ground. From a point on the ground, which is 48 m away from the foot of the tower, the angle of elevation of the top of the tower is 30° . Find the height of the tower.
Solution :
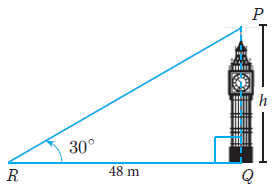
In the triangle PRQ,
Opposite side = h, hypotenuse = PR and adjacent side = PQ
tan θ = Opposite side / adjacent side
tan 30 = PQ / RQ
1/√3 = h/48
h = 48 / √3
Rationalizing the denominator, we get
h = 48√3/3
h = 16√3
Then, height of the tower is 16√3 m.
Problem 4 :
A kite is flying at a height of 75 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60° . Find the length of the string, assuming that there is no slack in the string.
Solution :
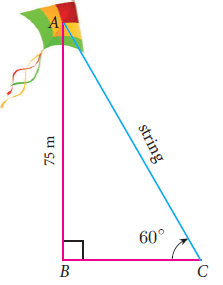
Length of string = hypotenuse
In triangle ABC, AB = opposite side
BC = Adjacent side
AC = Hypotenuse
sin θ = Opposite side / Hypotenuse
sin 60 = AB / AC
√3/2 = 75/AC
AC = 75(2) / √3
Rationalizing the denominator, we get
AC = 150 / √3
= 150√3/3
= 50√3
So, the length of the string is 50√3 m.
Problem 5 :
Two ships are sailing in the sea on either sides of a lighthouse. The angle of elevation of the top of the lighthouse as observed from the ships are 30° and 45° respectively. If the lighthouse is 200 m high, find the distance between the two ships. (√3 = 1.732 )
Solution :
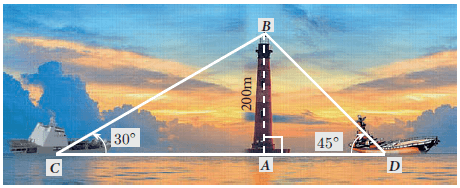
In triangles ABC and ABD.
tan θ = Opposite side / adjacent side
tan 30 = AB / AC
1/√3 = 200/AC
AC = 200√3 --(1)
tan 45 = AB / AD
1 = 200/AD
AD = 200 --(2)
Distance between two ships = AC + AD
= 200√3 + 200
= 200(√3 + 1)
= 200(1.732 + 1)
= 200(2.732)
= 546.4 m
So, the distance between two ships is 546.4 m.
Problem 6 :
From a point on the ground, the angles of elevation of the bottom and top of a tower fixed at the top of a 30 m high building are 45° and 60° respectively. Find the height of the tower. (√3 = 1.732)
Solution :
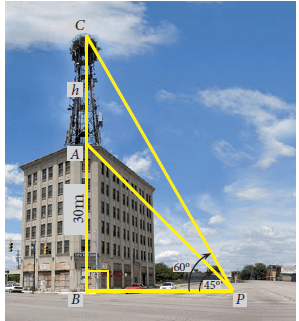
In triangle ABP,
tan θ = Opposite side / adjacent side
tan 45 = AB / BP
1 = 300 / BP
BP = 300 ----(1)
tan 60 = (AB + AC) / BP
√3 = (300 + h) / BP
BP = (300 + h)/√3 ----(2)
(1) = (2)
300 = (300 + h) / √3
300 √3 = 300 + h
h = 300√3 - 300
h = 300(√3 - 1)
= 300(1.732 - 1)
= 300 (0.732)
h = 219.6
Height of the tower = 300 + 219.6
= 519.6 m
So, the height of the tower is 519.6 m.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29) -
10 Hard SAT Math Questions (Part - 28)
Oct 14, 25 10:57 AM
10 Hard SAT Math Questions (Part - 28)
