ANGLE RELATIONSHIPS WORKSHEET
Problems 1-7 : Find the value of x in the diagram.
Problem 1 :
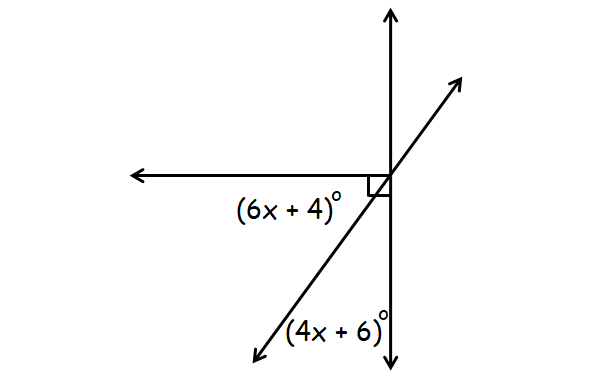
Problem 2 :
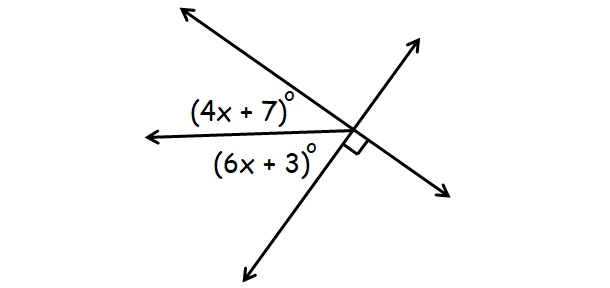
Problem 3 :
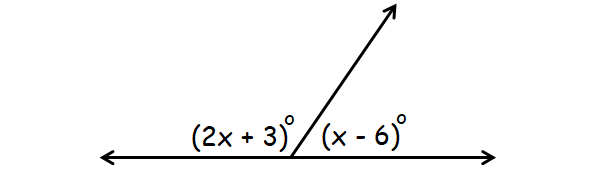
Problem 4 :
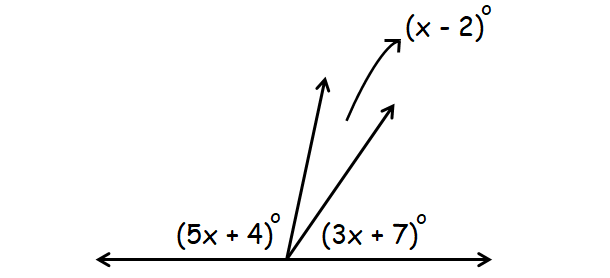
Problem 5 :
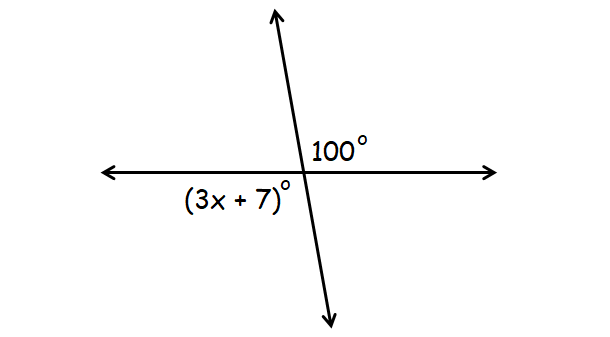
Problem 6 :
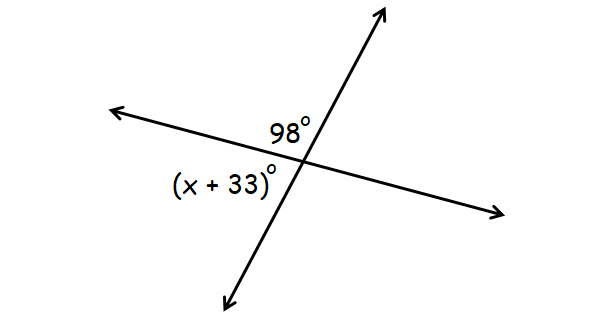
Problems 7-8 : In given the diagram, lines l1 and l2 are parallel and t is a transversal. Find the value of x.
Problem 7 :
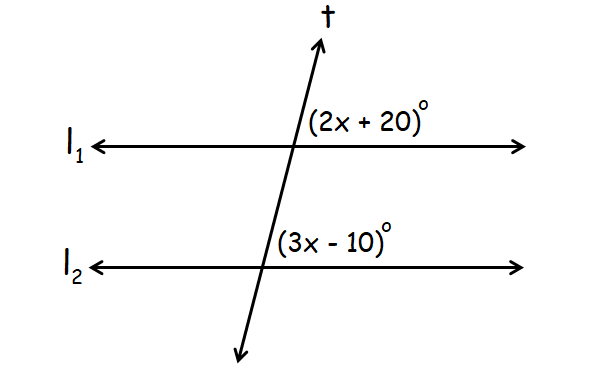
Problem 8 :
In the diagram given below, l1 lines l2 are parallel and t is a transversal. Find the value of x.
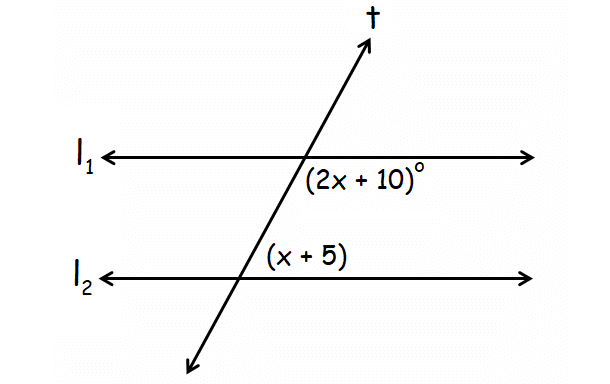
Problem 9 :
In the diagram given below, a lines b are parallel and t is a transversal. Find the value of x.
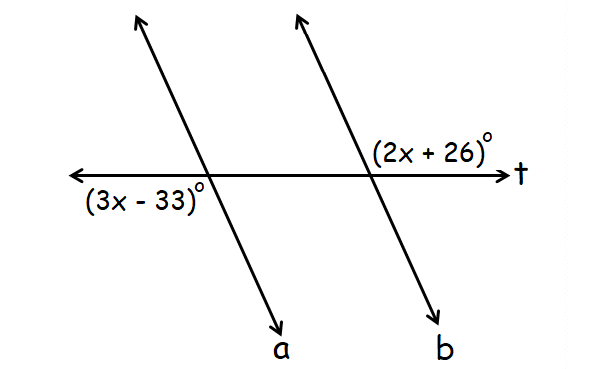
Problem 10 :
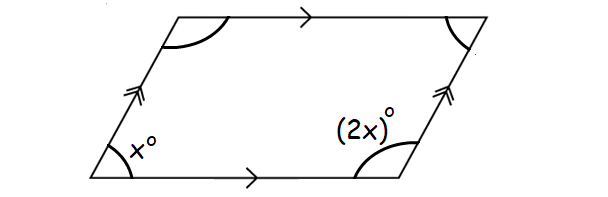
In the diagram given below, find the value of x.

Answers
1. Answer :
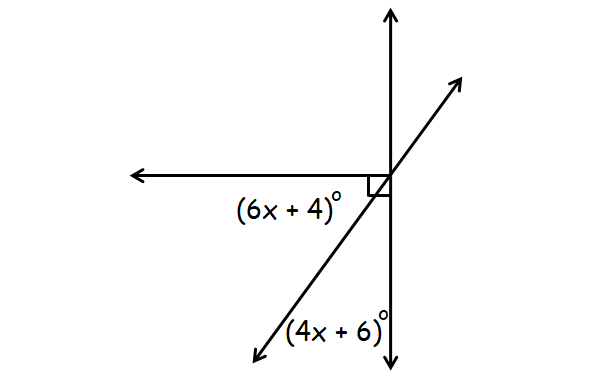
In the diagram above, (6x + 4)° and (4x + 6)° are complementary.
(6x + 4)° + (4x + 6)° = 90°
6x + 4 + 4x + 6 = 90
10x + 10 = 90
10x = 80
x = 8
2. Answer :
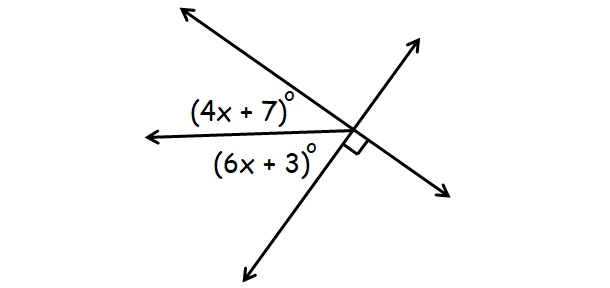
In the diagram above, (4x + 7)° and (6x + 3)° are complementary.
(4x + 7)° + (6x + 3)° = 90°
4x + 7 + 6x + 3 = 90
10x + 10 = 90
10x = 80
x = 8
3. Answer :
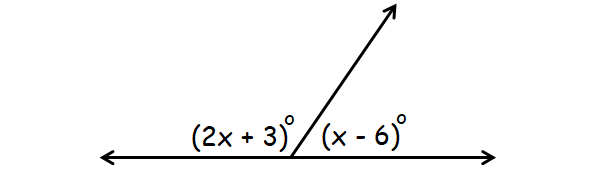
In the diagram above, (2x + 3)° and (x - 6)° are supplementary angles.
(2x + 3)° + (x - 6)° = 180°
2x + 3 + x - 6 = 180
3x - 3 = 180
3x = 183
x = 61
4. Answer :
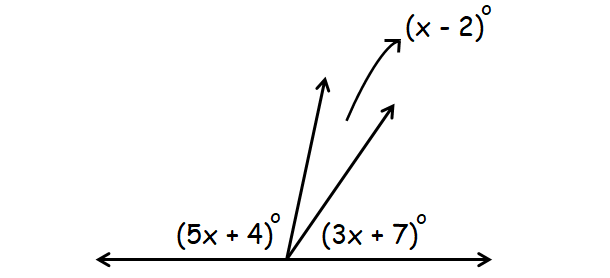
In the diagram above, (5x + 4)°, (x - 2)° and (3x + 7)° are supplementary angles.
(5x + 4)° + (x - 2)° + (3x + 7)° = 180°
5x + 4 + x -2 + 3x + 7 = 180
9x + 9 = 180
9x = 171
x = 19
5. Answer :
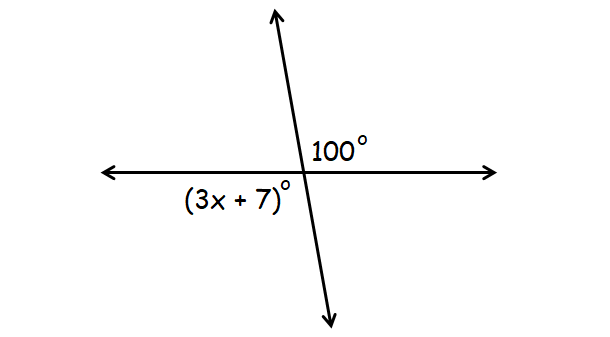
In the diagram above, (3x + 7)° and 100° are vertical angles.
(3x + 7)° = 100°
3x + 7 = 100
3x = 93
x = 31
6. Answer :

In the diagram above, (x + 33)° and 98° form a linear pair.
(x + 33)° + 98° = 180°
x + 33 + 98 = 180
x + 131 = 180
x = 49
7. Answer :
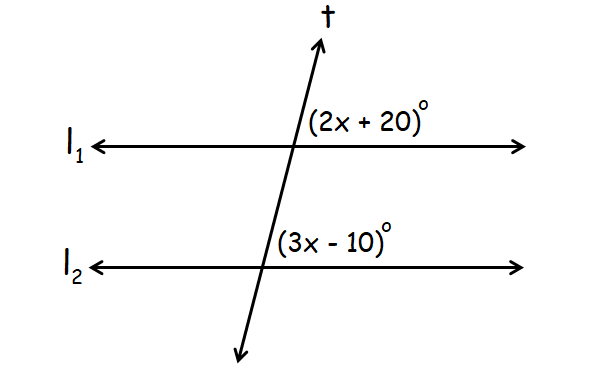
In the above diagram, (2x + 20)° and (3x - 10)° are corresponding angles.
When two parallel lines are cut by a transversal, corresponding angles are congruent.
(2x + 20)° = (3x - 10)°
2x + 20 = 3x - 10
30 = x
8. Answer :
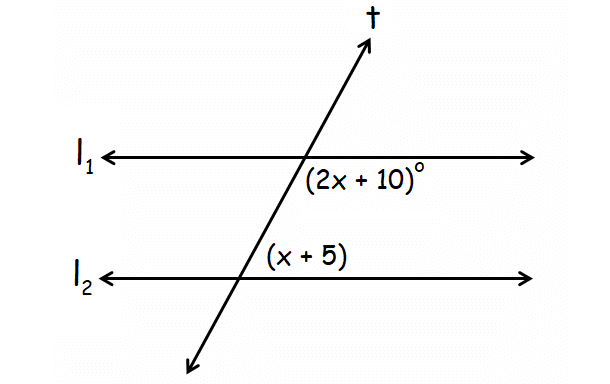
In the above diagram, (2x + 10)° and (x + 5)° are consecutive interior angles.
When two parallel lines are cut by a transversal, consecutive interior angles are supplementary.
(2x + 10)° + (x + 5)° = 180°
2x + 10 + x + 5 = 180
3x + 15 = 180
3x = 165
x = 55
9. Answer :
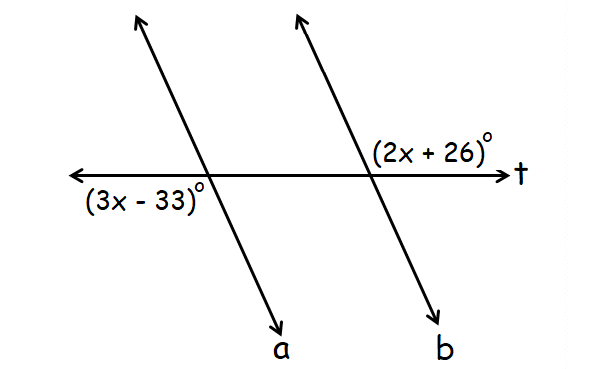
In the diagram diagram, (2x + 26)° and (3x - 33)° are alternate interior angles.
When two parallel lines are cut by a transversal, alternate interior angles are congruent.
(2x + 26)° = (3x - 33)°
2x + 26 = 3x - 33
59 = x
10. Answer :

In the diagram diagram, it is clear that AB||CD and AD||BC.
So ABCD is a parallelogram.
In a parallelogram, two consecutive angles are always supplementary.
x° + (2x)° = 180°
x + 2x = 180
3x = 180
x = 60
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
AP Calculus AB Problems with Solutions (Part - 7)
Jan 26, 25 06:56 AM
AP Calculus AB Problems with Solutions (Part - 7) -
Digital SAT Math Problems and Solutions (Part - 103)
Jan 25, 25 01:00 AM
Digital SAT Math Problems and Solutions (Part - 103) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Jan 25, 25 12:52 AM
SAT Math Resources (Videos, Concepts, Worksheets and More)