ANGLE THEOREMS FOR TRIANGLES

In this section, you will learn the following two important theorems. 

1.  Triangle sum theorem

2.  Exterior angle theorem

Triangle Angle Theorem

Step 1 : 

Draw a triangle and cut it out. Label the angles A, B, and C.

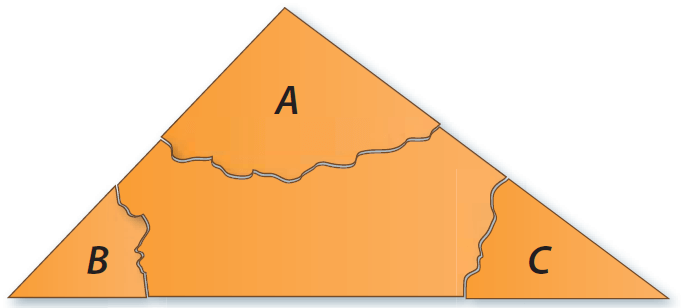
Step 2 : 

Tear off each “corner” of the triangle. Each corner includes the vertex of one angle of the triangle.

Step 3 : 

Arrange the vertices of the triangle around a point so that none of your corners overlap and there are no gaps between them.

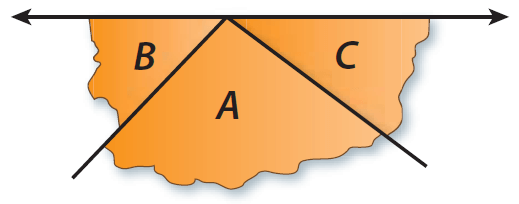
Step 4 : 

What do you notice about how the angles fit together around a point ?

The angles form a straight angle.

Step 5 : 

What do you notice about how the angles fit together around a point ?

180°

Step 6 : 

Describe the relationship among the measures of the angles of triangle ABC ?

The sum of the angle measures is 180°.

Step 7 : 

What does the triangle sum theorem state ? 

The triangle sum theorem states that for triangle ABC,  

m∠A + m∠B + m∠C =  180°

Exterior Angle Theorem

Step 1 :

Sketch a triangle and label the angles as m∠1, m∠2 and m∠3.

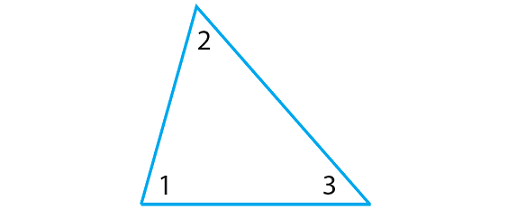
Step 2 :

According to Triangle Sum Theorem, we have

m∠1 + m∠2 + m∠3  =  180° ------ (1)

Step 3 :

Extend the base of the triangle and label the exterior angle as m∠4.

Step 4 :

m∠3 and m∠4 are the angles on a straight line. 

So, we have 

m∠3 + m∠4  =  180° ------ (2)

Step 5 :

Use the equations (1) and (2) to complete the following equation,  

m∠1 + m∠2 + m∠3  =  m∠3 + m∠4 ------ (3)

Step 6 :

Use properties of equality to simplify the equation (3). 

m∠1 + m∠2 + m∠3  =  m∠3 + m∠4

Subtract m∠3 from both sides.

aaaaaaaaaaa m∠1 + m∠2 + m∠3  =  m∠3 + m∠4 aaaaaaaaaaa aaaaaaaaaaaaaaaaaaaaa  - m∠3   - m∠3 aaaaaaaaaaaaaaaaa aaaaaaaaaaa ------------------------------------ aaaaaaaaaaa aaaaaaaaaaa m∠1 + m∠2             =            m∠4 aaaaaaaaaaa aaaaaaaaaaa ------------------------------------ aaaaaaaaaaa

Hence, the Exterior Angle Theorem states that the measure of an exterior angle is equal to the sum of its remote interior angles.

That is, 

m∠1 + m∠2  =  m∠4

Solved Problems

Problem 1 : 

Can 30°, 60° and 90° be the angles of a triangle ?

Solution :

Let us add all the three given angles and check whether the sum is equal to 180°.

 30° +  60° + 90°  =  180°

Since the sum of the angles is equal 180°, the given three angles can be the angles of a triangle. 

Problem 2 : 

Can 35°, 55° and 95° be the angles of a triangle ?

Solution :

Let us add all the three given angles and check whether the sum is equal to 180°.

 35° +  55° + 95°  =  185°

Since the sum of the angles is not equal 180°, the given three angles can not be the angles of a triangle. 

Problem 3 : 

In a triangle, if the second angle is 5° greater than the first angle and the third angle is 5° greater than second angle, find the three angles of the triangle. 

Solution :

Let "x" be the first angle.

The second angle  =  x + 5

The third angle  =  x + 5 + 5  =  x + 10

We know that,

the sum of the three angles of a triangle  =  180°

x + (x+5) + (x+10)  =  180°

3x + 15  =  180

3x  =  165

x  =  55

The first angle  =  55°

The second angle  =  55 + 5  =  60°

The third angle  =  60 + 5  =  65°

So, the three angles of a triangle are 55°, 60° and 65°. 

Problem 4 : 

Find m∠W and m∠X in the triangle given below.

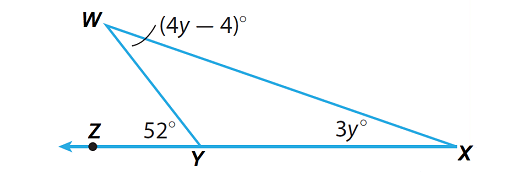
Solution : 

Step 1 : 

Write the Exterior Angle Theorem as it applies to this triangle.

m∠W + m∠X  =  m∠WYZ

Step 2 : 

Substitute the given angle measures.

(4y - 4)° + 3y°  =  52°

Step 3 : 

Solve the equation for y.

(4y - 4)° + 3y°  =  52°

4y - 4 + 3y  =  52

Combine the like terms. 

7y - 4  =  52

Add 4 to both sides.

7y - 4 + 4  =  52 + 4

Simplify.

7y  =  56

Divide both sides by 7. 

7y / 7  =  56 / 7

y  =  8

Step 4 : 

Use the value of y to find m∠W and m∠X.

So, m∠W  =  28° and m∠X  =  24°.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.