ANGLES AT THE CENTER AND CIRCUMFERENCE EXAMPLES

Example 1 :

Find the value of x :

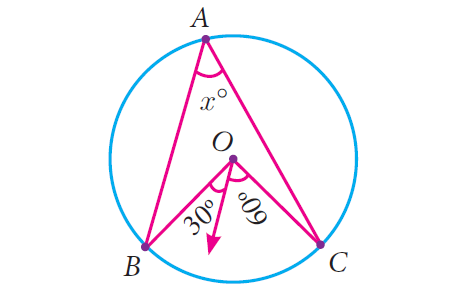
Solution :

∠BOC  =  2∠BAC

90°  =  2x°

90  =  2x

Divide each side by 2.

45  =  x

Example 2 :

Find the value of x :

Solution :

OP  =  OQ  (Radius)

Equal sides will form equal angles.

∠OQP  =  ∠QPO  =  x°

∠ORP  =  ∠OPR  =  30°

∠QPR  =  x° + 30°

2∠QPR  =  ∠QOR

2(x° + 30°)  =  80°

2(x + 30)  =  80

Divide each side by 2.

x + 30  =  40

Subtract 30 from each side. 

x  =  10

Example 3 :

Find the value of x :

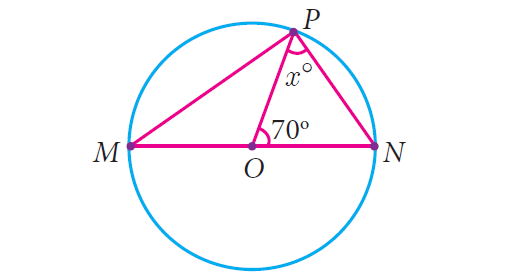
In triangle PON,

OP  =  ON  (Radius)  =  x

∠ONP + ∠NOP + ∠OPN  =  180

x° + 70° + x°  =  180°

x + 70 + x  =  180

2x + 70  =  180

Subtract 70 from each side.

2x   =  110

Divide each side by 2.

x  =  55

Example 4 :

Find the value of x :

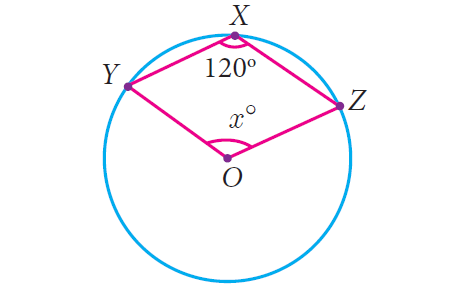
Solution :

x°  =  120°

x  =  120

Example 5 :

Find the value of x :

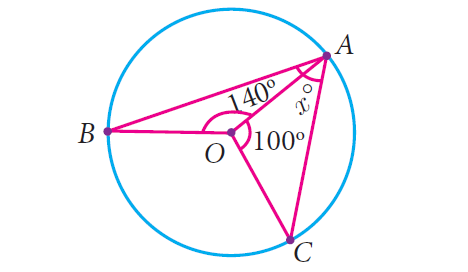
OA  =  OC  (Radius)

Equal sides will form equal angles.

∠OAC  =  ∠OCA  =  x°

In triangle OAC, 

x° + x° + 100°  =  180°

x + x + 100  =  180

2x + 100  =  180

Subtract 100 from each side. 

2x  =  80

Divide each side by 2. 

x  =  40

Question 2 :

In the given figure, ∠CAB = 25°, find ∠BDC, ∠DBA and ∠COB. 

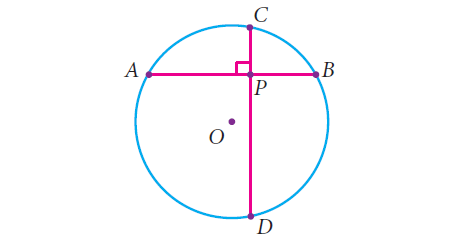
Solution :

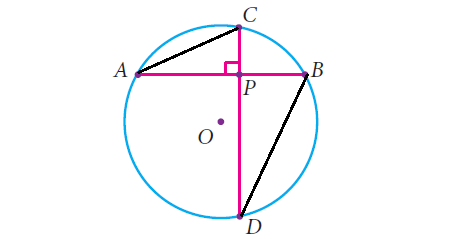
In triangle APC,

∠APC + ∠PCA + ∠CAP  =  180°

90° + ∠PCA + 25°  =  180°

∠PCA + 115°  =  180°

Subtract 115° from each side. 

∠PCA  =  65°

∠DBA  =  65° (Angle in same segment)

∠BDC  =  25° (angle in same segment)

∠COB  =  50°

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.