AP Calculus AB Problems with Solutions (Part - 9)

Problem 1 :

Given f(x) = 10 - 16/x, find all c in the interval [2, 8] that satisfies the Mean Value Theorem.

Solution :

Problem 2 :

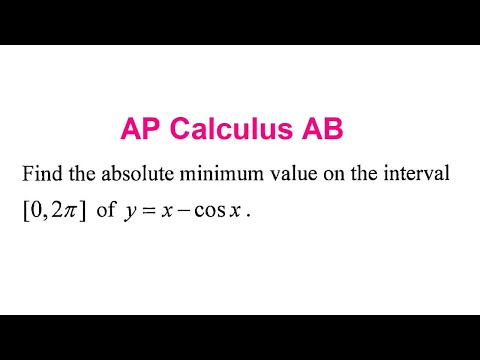
Find the absolute minimum value on the interval [0, 2π] of y = x - cos x.

Solution :

Problem 3 :

f(x) = -x³ + 18x² - 105x + 198

Which of the following statements is true of the function given above?

A) f is decreasing on the interval on (6, ∞)

B) f is decreasing on the interval on (5, 7)

C) f is increasing on the interval on (-∞, 5)

D) f is increasing on the interval on (5, 7)

E) None of these

Solution :

Problem 4 :

Find the values of x that give relative extrema for the function f(x) = 3x⁵ - 5x³ and determine the type.

Solution :

Problem 5 :

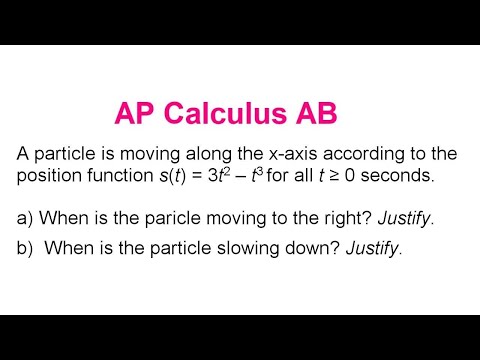
A particle is moving along the x-axis according to the position function s(t) = 3t² – t³for all t ≥ 0 seconds.

a) When is the paricle moving to the right? Justify.

b) When is the particle slowing down? Justify.

Solution :

You might like these

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.