AP Calculus AB Problems with Solutions (Part - 1)

Problem 1 :

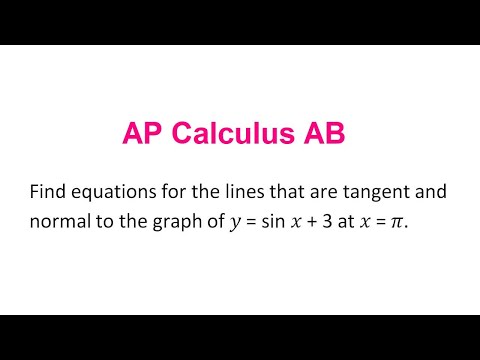
Find equations for the lines that are tangent and normal to the graph of y = sin x + 3 at x = 𝜋.

Solution :

Problem 2 :

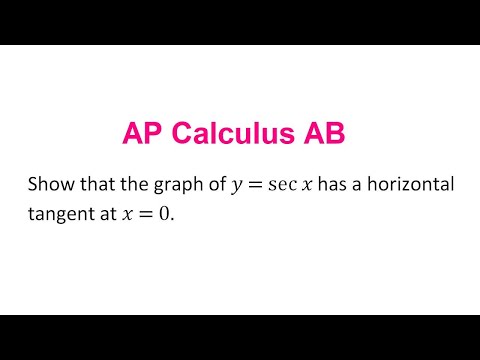
Show that the graph of y = sec x has a horizontal tangent at x = 0.

Solution :

Problem 3 :

Find equations for the lines that are tangent and normal to the curve y = √2 cos x at the point (𝜋/4, 1).

Solution :

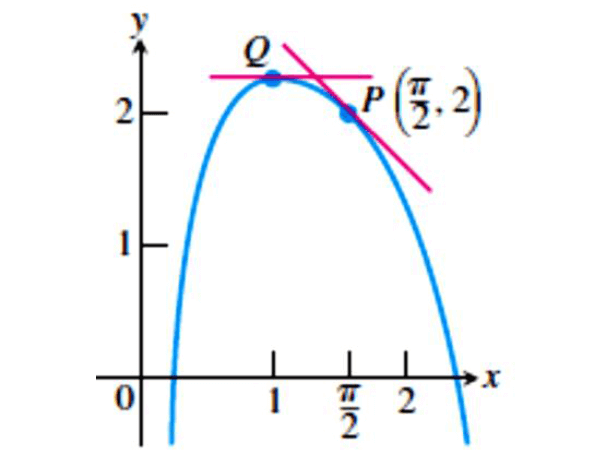
Problem 4 :

Find equations for the lines that are tangent and normal to the curve y = √2 cos x at the point (𝜋/4,1).

Solution :

Problem 5 :

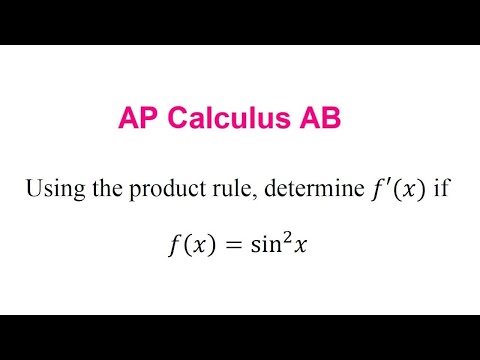
Using the product rule, determine 𝑓′'(x), if 𝑓(x)=sin²x.

Solution :

You might like these

AP Calculus AB Problems with Solutions (Part - 1)

AP Calculus AB Problems with Solutions (Part - 2)

AP Calculus AB Problems with Solutions (Part - 3)

AP Calculus AB Problems with Solutions (Part - 4)

AP Calculus AB Problems with Solutions (Part - 5)

AP Calculus AB Problems with Solutions (Part - 6)

AP Calculus AB Problems with Solutions (Part - 7)

AP Calculus AB Problems with Solutions (Part - 8)

AP Calculus AB Problems with Solutions (Part - 9)

AP Calculus AB Problems with Solutions (Part - 10)

AP Calculus AB Problems with Solutions (Part - 11)

AP Calculus AB Problems with Solutions (Part - 12)

AP Calculus AB Problems with Solutions (Part - 13)

AP Calculus AB Problems with Solutions (Part - 14)

AP Calculus AB Problems with Solutions (Part - 15)

AP Calculus AB Problems with Solutions (Part - 16)

AP Calculus AB Problems with Solutions (Part - 17)

AP Calculus AB Problems with Solutions (Part - 18)

AP Calculus AB Problems with Solutions (Part - 19)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.