APPLICATION PROBLEMS OF RATE OF CHANGE IN CALCULUS

Problem 1 :

Newton's law of cooling is given by θ = θ₀° e^⁻kt, where the excess of temperature at zero time is θ₀° C and at time t seconds is θ° C. Determine the rate of change of temperature after 40 s given that θ₀ = 16° C and k = -0.03.(e^1.2  =  3.3201)

Solution :

Newton's law of cooling θ  =  θ₀° e^⁻kt

θ₀°  =  16° C

k  =  -0.03

rate of change of temperature with respect to time

dθ/dt = - k θ₀° e^(⁻kt)

t  =  40

dθ/dt  =  - (-0.03) (16)e^(⁻0.03) (40)

  =  0.48  e^⁻1.2

  =  0.48 (3.3201)

  =  1.5936° C/s

Problem 2 :

The altitude of a triangle is increasing at a rate of 1 cm/min while the area of the triangle is increasing at a rate of 2 cm²/min. At what rate is the base of the triangle changing when the altitude is 10 cm and the area is 100 cm².

Solution :

Let "b" and "h" be the base and height of the triangle ABC.

Area of triangle ABC

A  =  (1/2) b h

h  =  10      area  =  100

100  =  (1/2) x b x 10

b  =  (100 x 2)/10

b  =  20

dA/dt  =  (1/2) [b (dh/dt) + h (db/dt)]

h (db/dt)  =  2 (dA/dt) - b (dh/dt)

db/dt  =  (2/h) (dA/dt) - (b/h) (dh/dt)

db/dt  =  (2/10) (2) - (20/10) (1)

db/dt  =  (4/10) - (20/10)

db/dt  =  -16/10

db/dt  =  -1.6 cm/min

Problem 3 :

At noon, ship A is 100 km west of ship B. Ship A is sailing east at 35 km/hr and ship B us sailing north at 25 km/hr. How fast is the distance between the ship changing at 4.00 p.m

Solution :

Let P and Q are the starting position of the ships A and B.

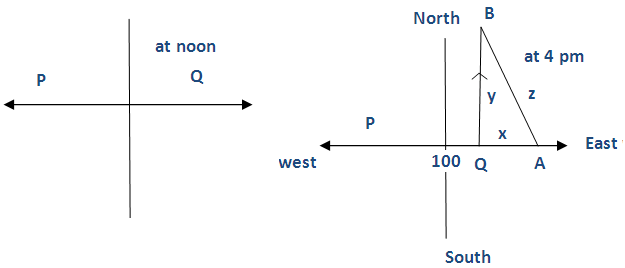
Let x, y and z be the distance between QA and QB and AB respectively.

z²  =  x² + y²

Here x, y and z are changing with respect to time.

2z (dz/dt)  =  2x (dx/dt) + 2y (dy/dt)

Dividing each side by 2.

z (dz/dt)  =  x (dx/dt) + y (dy/dt)

dx/dt  =  speed of ship A  =  35 km/hr

dy/dt  =  speed of B = 25 km/hr

x  =  40,  y  =  100

z  =  √(40)² + (100)²

z  =  √1600 + 10000

z  =  √2600

z  =  20√29

20√29 (dz/dt)  =  40 (35) + (100) (25)

20√29 (dz/dt)  =  1400 + 2500

20√29 (dz/dt)  =  3900

dz/dt  =  3900 /(20√29)

dz/dt  =  195/√29

There the rate of change of distance between two ships is 195/√29 km/hr.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.