AREA AND PERIMETER WORKSHEET
Problem 1 :
Find the area of the square shown below.
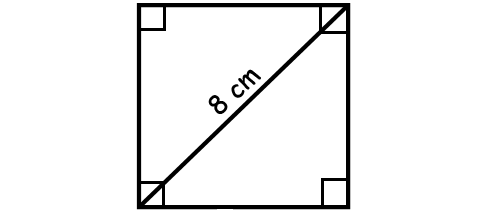
Problem 2 :
Find the perimeter of the rectangle shown below.
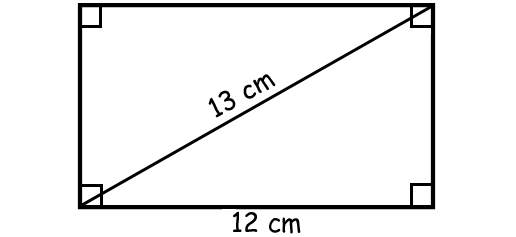
Problem 3 :
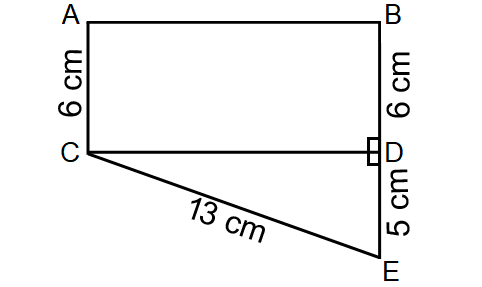
Find the area of the quadrilateral shown below.
Problem 4 :
Find the area of the parallelogram shown below.
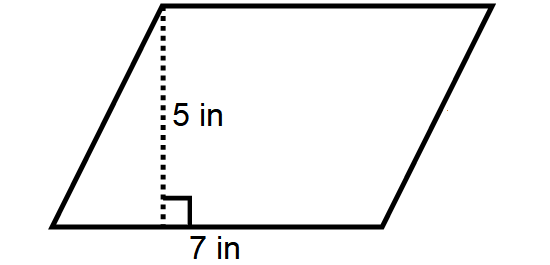
Problem 5 :
The length of each side of the square inscribed into the circle shown below is 4 cm. Find the perimeter of the circle.
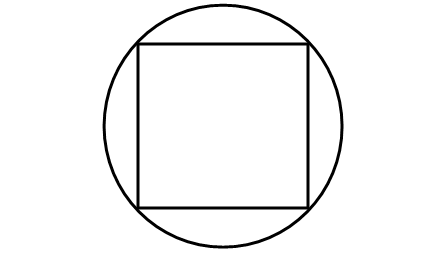
Problem 6 :
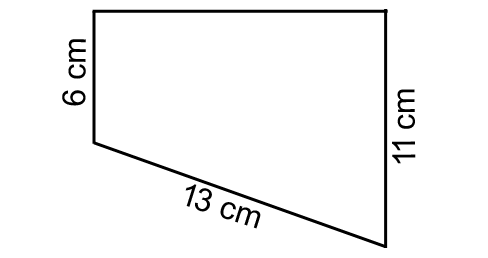
Find the area of the figure shown below.
Problem 7 :
A regular pentagon is inscribed in a circle with radius 1 unit. Find the area of the pentagon.
Problem 8 :
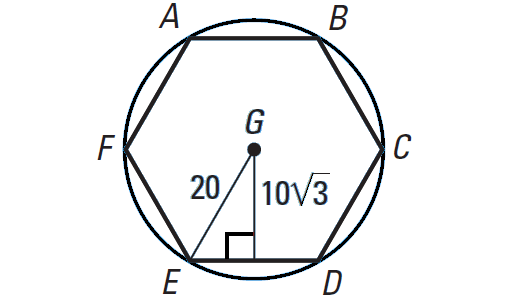
Find the area of the regular hexagon shown below.
Detailed Answer Key
Problem 1 :
Find the area of the square shown below.
Solution :
In the square shown above, the length of the diagonal is 8 cm.
When the length of a diagonal is given, formula for area of a square :
= 1/2 ⋅ d2
Substitute 8 for d.
= 1/2 ⋅ 82
Simplify.
= 1/2 ⋅ 64
= 32
So, the area of the square shown above is is 32 square cm.
Problem 2 :
Find the perimeter of the rectangle shown below.
Solution :
In the above rectangle, width is 12 cm and the diagonal measures 13 cm.
To find the perimeter of a rectangle, we need to know the length and width.
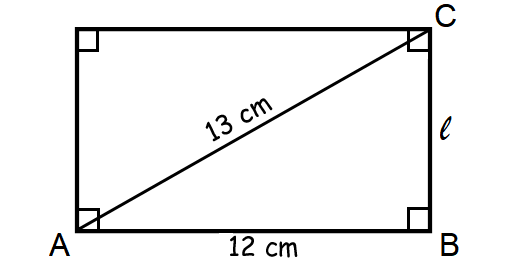
In the figure shown above, consider the right triangle ABC.
By Pythagorean Theorem, we have
AB2 + BC2 = AC2
Substitute.
122 + l2 = 132
Simplify and solve for l.
144 + l2 = 169
Subtract 144 from each side.
l2 = 25
Find positive square root on both sides.
√l2 = √25
l = 5
Therefore, the length of the rectangle is 5 cm.
Formula for perimeter of a rectangle :
= 2(l + w)
Substitute 5 for l and 12 for w.
= 2(5 + 12)
= 2(17)
= 34
So, the perimeter of the rectangle shown above is 34 cm.
Problem 3 :
Find the area of the quadrilateral shown below.
Solution :
Formula for area of a quadrilateral :
= 1/2 ⋅ d ⋅ (h1 + h2)
Substitute 3 for h1 and 6 for h2 and 10 for d.
= 1/2 ⋅ 10 ⋅ (3 + 6)
= 1/2 ⋅ 10 ⋅ 9
= 45
So, the area of the quadrilateral shown above is 45 square cm.
Problem 4 :
Find the area of the parallelogram shown below.
Solution :
Formula for area of a parallelogram :
= b ⋅ h
Substitute 7 for h and 5 for b.
= 7 ⋅ 5
= 35
So, the area of the quadrilateral shown above is 35 square inches.
Problem 5 :
The length of each side of the square inscribed into the circle shown below is 4 cm. Find the perimeter of the circle.
Solution :
To find the perimeter of the circle, we need to know its radius.
Let us find the radius of the circle.
In the figure shown above, consider the right triangle OBC.
By Pythagorean Theorem, we have
OB2 = OC2 + CB2
Substitute.
OB2 = 22 + 22
Simplify and solve for OB.
OB2 = 4 + 4
OB2 = 8
Find positive square root on both sides.
√OB2 = √8
OB = 2√2
Therefore, the radius of the circle is 2√2 cm.
Formula for perimeter of a circle is
= 2πr
Substitute 2√2 for r.
= 2π(2√2)
= 4√2π cm
So, the perimeter of the circle shown above is 4√2π cm.
Problem 6 :
Find the area of the figure shown below.
Solution :
We can find the area of the figure shown above by dividing the figure into rectangle and triangle as shown below.
In the figure shown above, consider the right triangle CDE.
By Pythagorean Theorem, we have
CD2 + DE2 = CE2
Substitute.
CD2 + 52 = 132
Simplify and solve for CD.
CD2 + 25 = 169
Subtract 25 from each side.
CD2 = 144
Find positive square root on both sides.
√CD2 = √144
CD = 12
Therefore, the width of the rectangle is 12 cm.
And also, the base of the triangle is 12 cm.
Area of the rectangle ABCD :
Area = l ⋅ w
Substitute 6 for l and 12 for w.
Area = 6 ⋅ 12
Area = 72 cm2
Area of the triangle CDE :
Area = 1/2 ⋅ b ⋅ h
Substitute 12 for b and 5 for h.
Area = 1/2 ⋅ 12 ⋅ 5
Area = 30 cm2
Area of the complete figure :
= Area of the rectangle ABCD + Area of the triangle CDE
= 72 + 30
= 102 cm2
So, the area of the figure shown above is 102 square cm.
Problem 7 :
A regular pentagon is inscribed in a circle with radius 1 unit. Find the area of the pentagon.
Solution :
Draw a sketch.
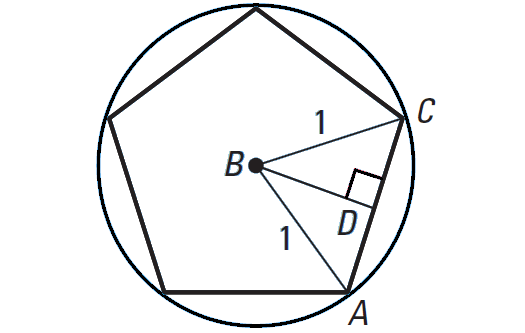
To apply formula for the area of a regular pentagon, we must find its apothem and perimeter.
The measure of central ∠ABC is
= 1/5 ⋅ 360°
= 72°
Let us consider the isosceles triangle ΔABC in the above regular pentagon.
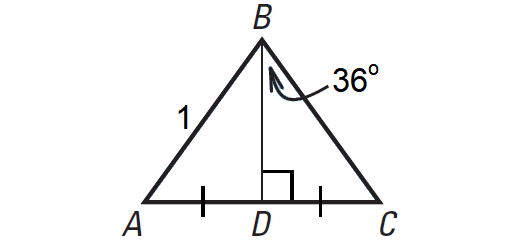
The altitude to base AC also bisects ∠ABC and side AC. The measure of ∠DBC, then, is 36°.
In right ΔDBC, we can use trigonometric ratios to find the lengths of the legs.
|
cos 36° = BD/BC cos 36° = BD/1 cos 36° = BD |
sin 36° = DC/BC sin 36° = DC/1 sin 36° = DC |
So, the pentagon has an apothem of
a = BD = cos 36°
and a perimeter of
P = 5(AC)
P = 5(2 ⋅ DC)
P = 10(DC)
P = 10 ⋅ sin 36°
The area of the pentagon is
A = 1/2 ⋅ apothem ⋅ perimeter of polygon
Substitute.
A = 1/2 ⋅ cos 36° ⋅ 10 ⋅ sin 36°
Simplify.
A = 5 ⋅ cos 36° ⋅ sin 36°
A ≈ 2.38 square units
Problem 8 :
Find the area of the regular hexagon shown below.
Solution :
To apply formula for the area of a regular hexagon, we must be knowing the apothem and perimeter.
Apothem is already given and we have to find the side length (ED) of the polygon to find its perimeter.
Let us consider the isosceles triangle ΔGED in the above regular pentagon.
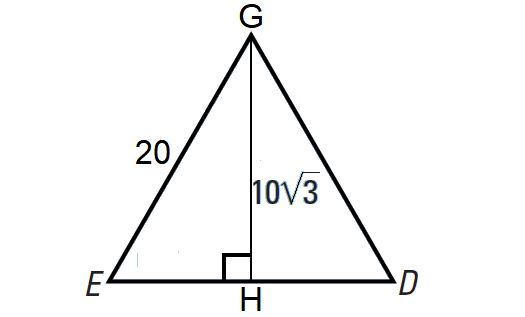
Because ΔGED is isosceles, the altitude GH to base ED bisects the side ED.
In right ΔEGH, we can use Pythagorean Theorem to find the length of EH.
EH2 + GH2 = EG2
Substitute.
EH2 + (10√3)2 = 202
EH2 + 300 = 400
Subtract 300 from each side.
EH2 = 100
Take square root on each side.
EH = 10
So, the hexagon has the perimeter of
P = 6(ED)
P = 6(2 ⋅ EH)
P = 6(2 ⋅ 10)
P = 6(20)
P = 120
The area of the hexagon is
A = 1/2 ⋅ apothem ⋅ perimeter of polygon
Substitute.
A = 1/2 ⋅ 10√3 ⋅ 120
Simplify.
A = 600√3 square units
Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.
If you have any feedback about our math content, please mail us :
v4formath@gmail.com
We always appreciate your feedback.
You can also visit the following web pages on different stuff in math.
WORD PROBLEMS
Word problems on simple equations
Word problems on linear equations
Word problems on quadratic equations
Area and perimeter word problems
Word problems on direct variation and inverse variation
Word problems on comparing rates
Converting customary units word problems
Converting metric units word problems
Word problems on simple interest
Word problems on compound interest
Word problems on types of angles
Complementary and supplementary angles word problems
Trigonometry word problems
Markup and markdown word problems
Word problems on mixed fractrions
One step equation word problems
Linear inequalities word problems
Ratio and proportion word problems
Word problems on sets and venn diagrams
Pythagorean theorem word problems
Percent of a number word problems
Word problems on constant speed
Word problems on average speed
Word problems on sum of the angles of a triangle is 180 degree
OTHER TOPICS
Time, speed and distance shortcuts
Ratio and proportion shortcuts
Domain and range of rational functions
Domain and range of rational functions with holes
Graphing rational functions with holes
Converting repeating decimals in to fractions
Decimal representation of rational numbers
Finding square root using long division
L.C.M method to solve time and work problems
Translating the word problems in to algebraic expressions
Remainder when 2 power 256 is divided by 17
Remainder when 17 power 23 is divided by 16
Sum of all three digit numbers divisible by 6
Sum of all three digit numbers divisible by 7
Sum of all three digit numbers divisible by 8
Sum of all three digit numbers formed using 1, 3, 4
Sum of all three four digit numbers formed with non zero digits
Recent Articles
-
How to Find Transpose of a Matrix
Oct 21, 20 02:53 AM
How to Find Transpose of a Matrix
-
How to Find the Number of Elements in a Matrix
Oct 21, 20 02:43 AM
How to Find the Number of Elements in a Matrix
-
Practice Test in Subtracting Decimals
Oct 21, 20 01:35 AM
Practice Test in Subtracting Decimals
