AREA OF PARALLELOGRAM
A parallelogram is a quadrilateral in which opposite sides are parallel and equal in length.
In other words opposite sides of a quadrilateral are equal in length, then the quadrilateral is called a parallelogram.
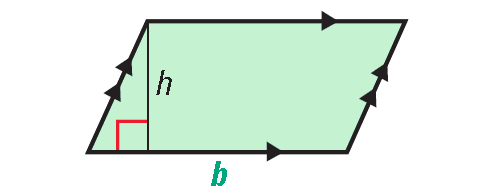
The area of a parallelogram is the product of a base and its corresponding height.
Then, the formula to find area of a parallelogram is given by
A = b ⋅ h square units
We can justify the area for parallelogram as follows.

The area of a parallelogram is the area of a rectangle with the same base and height.
Examples
Example 1 :
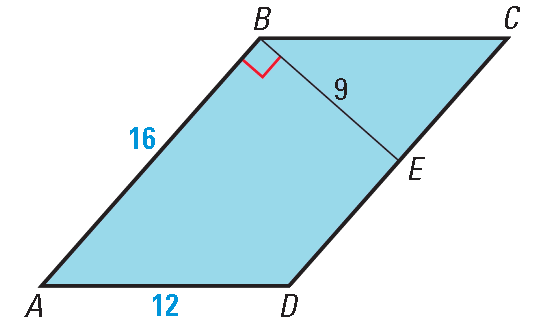
Find the area of the parallelogram ABCD shown below.
Solution :
Method 1 :
Use AB as the base.
So, b = 16 and h = 9.
Formula for area of a parallelogram is
= b ⋅ h
Substitute the given measures.
= 16 ⋅ 9
= 144 square units
Method 2 :
Use AD as the base.
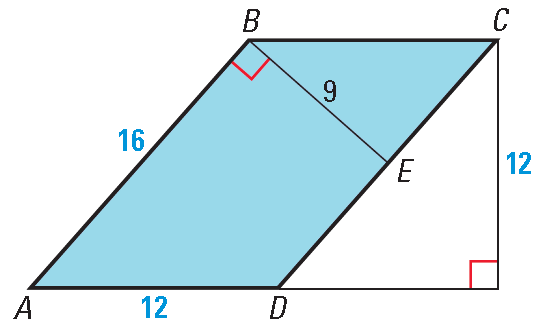
So, b = 12 and h = 12.
Formula for area of a parallelogram is
= b ⋅ h
Substitute the given measures.
= 12 ⋅ 12
= 144 square units
Example 2 :
Find the area of the parallelogram ABCD shown below.
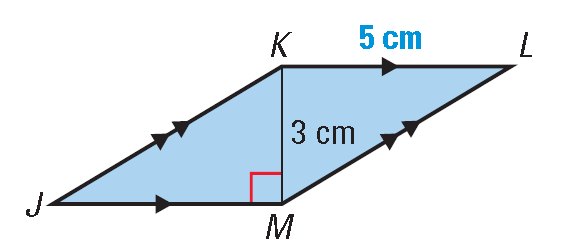
Formula for area of a parallelogram is
= b ⋅ h
Substitute b = 5 and h = 3.
= 5 ⋅ 3
= 15 square units
Example 3 :
A mirror is made of two congruent parallelograms as shown in the diagram. The parallelograms have a combined area of 9 1/3 square yards. The height of each parallelogram is 1 1/3 yards. How long is the base of each parallelogram ?
Solution :
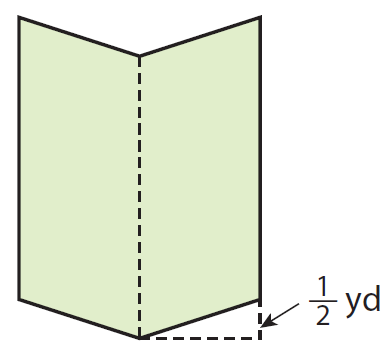
Because the given parallelograms are congruent area of two parallelogram will be equal.
Combined area of parallelograms = 9 1/3 square yards
Combined area of parallelograms = 28/3 square yards
Area of one parallelogram = (28/3) ÷ 2
Area of one parallelogram = 14/3
b ⋅ h = 14/3
b ⋅ 1 1/3 = 14/3
b ⋅ 4/3 = 14/3
Multiply each side by 3/4.
b = 14/3 ⋅ 3/4
b = 14/4
b = 7/2
b = 3 1/2
So, the base of the parallelogram is 3 1/2 yards.
Example 4 :
Find the base of a parallelogram if its area is 40 square cm and its altitude is 15 cm.
Solution :
Area of a parallelogram = 40 cm2
b ⋅ h = 40
Here, altitude (or) height (h) = 15 cm.
b ⋅ 15 = 40
Divide each side by 15.
b = 2.67
So, the base of the parallelogram is 2.67 inches.
Example 5 :
Find the area of the shape shown below.
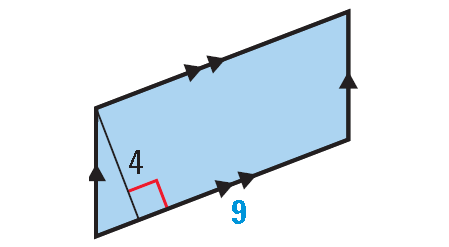
The above shape has four sides. So, it is a quadrilateral. Because the opposite sides are parallel, the above quadrilateral is a parallelogram.
Formula for area of a parallelogram is
= b ⋅ h
Substitute b = 9 and h = 4.
= 9 ⋅ 4
= 36 square units
Example 6 :
If the area of the shape shown below is 60 square inches, then find the value of x.
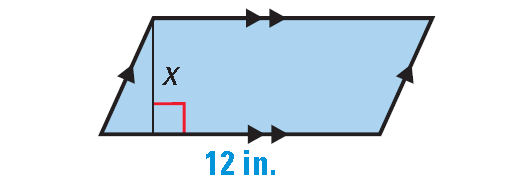
Solution :
Given : Area of the above shape is 60 square inches.
The above shape has four sides. So, it is a quadrilateral. Because the opposite sides are parallel, the above quadrilateral is a parallelogram.
Area = 60 in2
b ⋅ h = 60
Substitute b = 12 and h = x.
12 ⋅ x = 60
Divide each side by 12.
x = 5
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Solving Equations with the Given Roots
Jan 23, 25 04:57 AM
Solving Equations with the Given Roots -
Given Composite Function : How to Find the Inside or Outside Function
Jan 22, 25 02:43 AM
How to Find the Inside or Outside Function From the Given Composite Function -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Jan 20, 25 09:31 PM
SAT Math Resources (Videos, Concepts, Worksheets and More)