AREA OF A SECTOR WORKSHEET
Problem 1 :
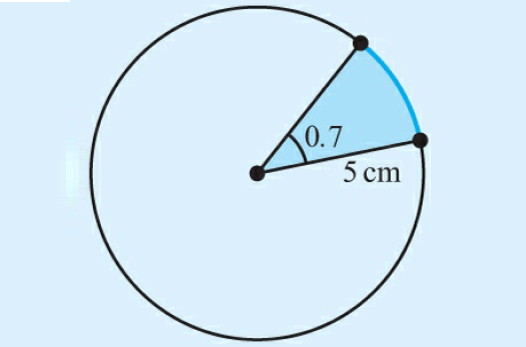
Find the area of the sector shaded in blue color. Angle given at the center is in radians.
Problem 2 :
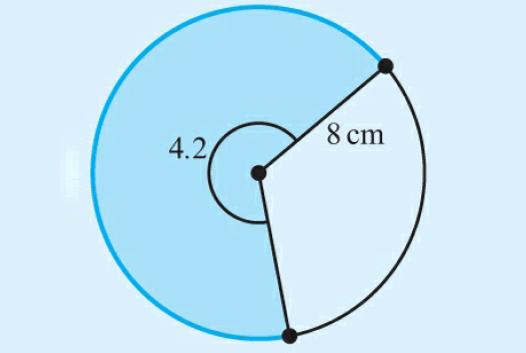
Find the area of the sector shaded in blue color. Angle given at the center is in radians.
Problem 3 :
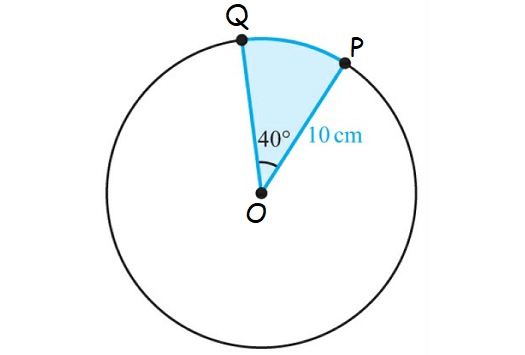
Find the area of the sector POQ.
Problem 4 :
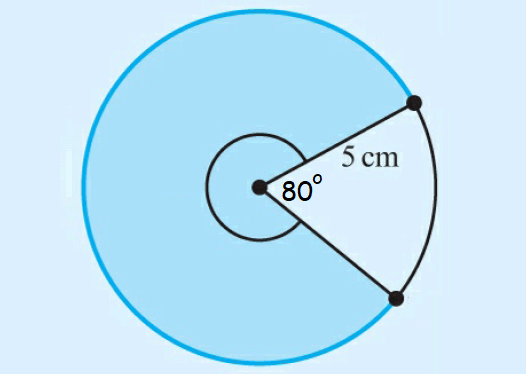
Find the area of the sector shaded in blue color.
Problem 5 :
A circle has radius 7.5 cm Find the area of the sector in the circle whose central angle measures π/4 radians.
Problem 6 :
A circle has radius 6 cm Find the area of the sector in the circle whose central angle measures 45°.
Problem 7 :
The area of a sector is 24π cm2. If the radius of the circle is 8 cm, find the central angle of the sector in radians.
Problem 8 :
The area of a sector is 25π cm2. If the radius is 10 cm, find the central angle of the sector in degrees.
Problem 9 :
The area of a sector is 18.75π cm2. If the central angle of the sector is π/6 radians, find the radius of the circle.
Problem 10 :
The area of a sector is 50 cm2. If the central angle of the sector is π/4 radians, find the area of the circle.

1. Solution :
Area of the sector :
= (1/2)r2θ
Substitute r = 5 and θ = 0.7.
= (1/2)(5)2(0.7)
= 8.75 cm2
2. Solution :
Area of the sector :
= (1/2)r2θ
Substitute r = 8 and θ = 4.2.
= (1/2)(8)2(4.2)
= 134.4 cm2
3. Solution :
Convert 40° to radians.
40° = 40° x (π/180°)
= 2π/9
Area of the sector :
= (1/2)r2θ
Substitute r = 10 and θ = 0.7.
= (1/2)(10)2(2π/9)
= 34.9 cm2
4. Solution :
Central angle of the shaded region :
= 360° - 80°
= 280°
Convert 280° to radians.
280° = 280° x (π/180°)
= 14π/9
Area of the sector :
= (1/2)r2θ
Substitute r = 5 and θ = 14π/9.
= (1/2)(5)2(14π/9)
= 61.09 cm2
5. Solution :
Area of the sector :
= (1/2)r2θ
Substitute r = 7.5 cm and θ = π/4.
= (1/2)(7.5)2(π/4)
= 22.09 cm2
6. Solution :
Convert 45° to radians.
45° = 45° x (π/180°)
= π/4
Area of the sector :
= (1/2)r2θ
Substitute r = 6 and θ = π/4.
= (1/2)(6)2(π/4)
= 14.14 cm2
7. Solution :
Area of the sector = 24π cm2
(1/2)r2θ = 24π
Substitute r = 8.
(1/2)(8)2θ = 24π
(1/2)(64)θ = 24π
32θ = 24π
Divide both sides by 32.
θ = 24π/32
θ = 3π/4
The central angle of the sector is 3π/4 radians.
8. Solution :
Area of the sector = 25π cm2
(1/2)r2θ = 25π
Substitute r = 10.
(1/2)(10)2θ = 25π
(1/2)(100)θ = 25π
50θ = 25π
Divide both sides by 50.
θ = π/2
Convert π/2 to degrees.
π/2 = (π/2) x (180°/π)
= 90°
The central angle of the sector is 90°.
9. Solution :
Area of the sector = 18.75π cm2
(1/2)r2θ = 18.75π
Substitute θ = π/6.
(1/2)r2(π/6) = 18.75π
πr2/12 = 18.75π
Multiply both sides by 12.
πr2 = 225π
Divide both sides by π.
r2 = 225
Take square root on both sides.
r = 15
The radius of the circle is 15 cm.
10. Solution :
Area of the sector = 50 cm2
(1/2)r2θ = 50
Substitute θ = π/4.
(1/2)r2(π/4) = 50
πr2/8 = 50
Multiply both sides by 8.
πr2 = 400
Area of the circle = 400 cm2
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 146)
Apr 18, 25 06:52 AM
Digital SAT Math Problems and Solutions (Part - 146) -
Logarithmic Derivative Problems and Solutions
Apr 16, 25 09:25 PM
Logarithmic Derivative Problems and Solutions -
Digital SAT Math Problems and Solutions (Part - 145)
Apr 16, 25 12:35 PM
Digital SAT Math Problems and Solutions (Part - 145)

