AREA OF QUADRILATERAL

A plane figure bounded by four sides is known as a quadrilateral. The straight line joining the opposite corners is called its diagonal. The diagonal divides the quadrilateral in to two triangles.

Area of Quadrilateral General Formula

Let us derive the area of quadrilateral general formula. Consider the quadrilateral ABCD shown below :

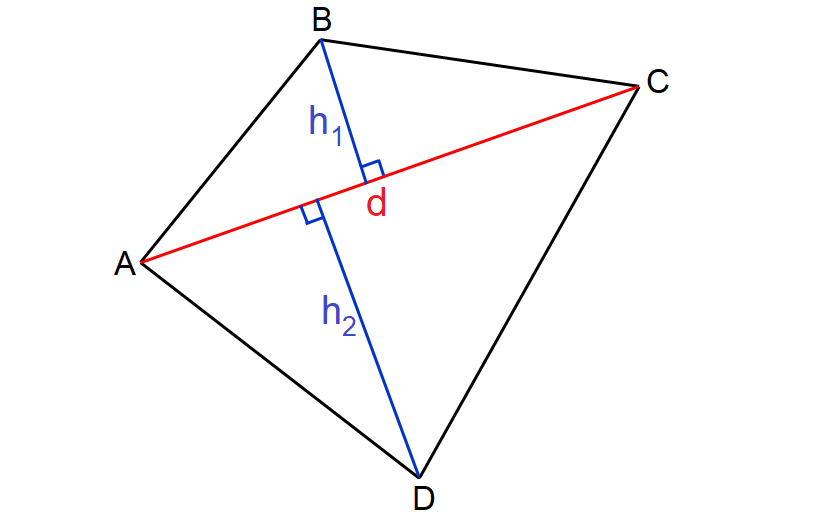
We can observe the quadrilateral above as a combination of two triangles, considering the diagonal AC (or d) as common base. h₁ and h₂ are the heights of the two triangles.  

Area of quadrilateral ABCD above can be calculated by adding the areas of two two triangles ABC and ADC.

The area of quadrilateral ABCD is

= Area of Δ ABC + Area of Δ ADC

= 1/2 ⋅ d ⋅ h₁ + 1/2 ⋅ d ⋅ h₂

= = 1/2 ⋅ d ⋅ (h₁ + h₂)

 Area of quadrilateral general formula : 

Here is the list of the formulas to find the area of quadrilaterals such as square, rectangle, parallelogram, trapezoid, kite and rhombus. 

Square

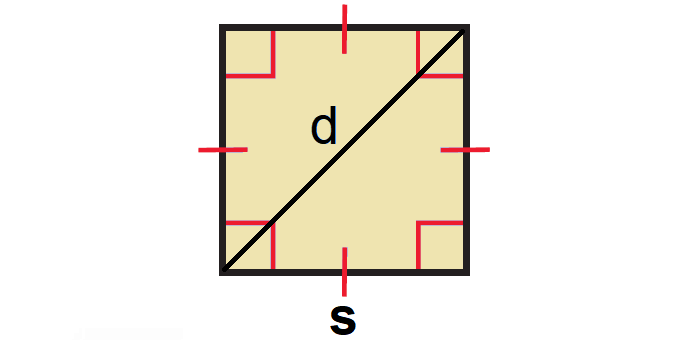
The area of a square is the product of the lengths of its two sides or square of the length of its diagonal. 

Area  =  s ⋅ s = s²

Area = 1/2 ⋅ d²

Rectangle

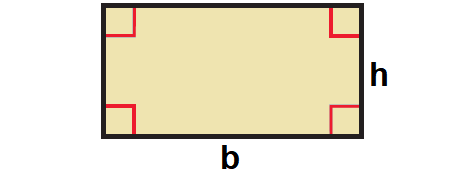
The area of a rectangle is the product of its base and height. 

Area  =  b ⋅ h

Parallelogram

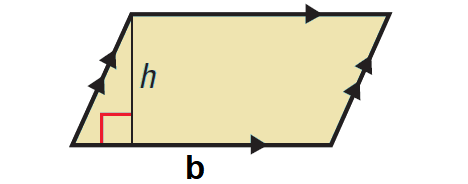
The area of a parallelogram is the product of a base and its corresponding height. 

Area  =  b ⋅ h

Trapezoid (Trapezium)

The area of a trapezoid is one half the product of the height and sum of the bases. 

Area  =  1/2 ⋅ h ⋅ (b₁ + b₂)

Kite

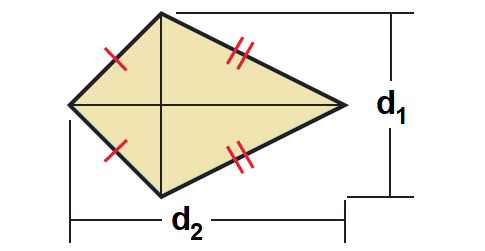
The area of a kite is one half the product of the lengths of its diagonals. 

Area  =  1/2 ⋅ d₁ ⋅ d₂

Rhombus

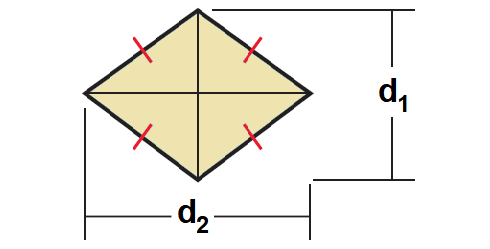
The area of a rhombus is one half the product of the lengths of its diagonals. 

Area  =  1/2 ⋅ d₁ ⋅ d₂

Problem 1 :

Find the area of parallelogram 

Solution :

Base = 16 ft and height = 18 ft

Area of parallelogram = base x height

= 16 x 18

= 288 square feet

Problem 2 :

Find the area of trapezium

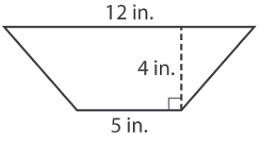
Solution :

Let the lengths of bases be a and b. Height be h.

a = 12 in, b = 5 in and h = 4 in

Area of trapezium = (1/2) x h (a + b)

= (1/2) x 4 x (12 + 5)

= 2(17)

= 34 square inches.

Problem 3 :

Find the area of rhombus.

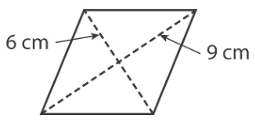
Solution :

Let d₁ and d₂ be the diagonals.

d₁ = 9 cm and d₂ = 6 cm

Area of rhombus = (1/2) x d₁ x d₂

= (1/2) x (9 x 6)

= 9 x 3

= 27 square cm.

Problem 4 :

A desktop in the shape of a parallelogram has a base of 30 inches and a height of 40 inches. What is the area of the desktop?

Solution :

Base = 30 in and height = 40 in

Area of parallelogram = base x height

= 30 x 40

= 1200 square inches

Problem 5 :

A rhombus has one diagonal that is 14 centimeters long and one diagonal that is 12 centimeters long. What is the area of the rhombus?

Solution :

Let d₁ and d₂ be the diagonals.

d₁ = 14 cm and d₂ = 12 cm

Area of rhombus = (1/2) x d₁ x d₂

= (1/2) x (14 x 12)

= 7 x 12

= 84 square cm.

Problem 6 :

The bases of a trapezoid are 24 feet and 16 feet. The height of the trapezoid is 12 feet. What is the area of the trapezoid?

Solution :

Let the lengths of bases be a and b. Height be h.

a = 24 feet, b = 16 feet in and h = 12 feet

Area of trapezium = (1/2) x h (a + b)

= (1/2) x 12 x (24 + 16)

= 6 x 40

= 240 square feet

Problem 7 :

A rectangular shaped swimming pool with dimensions 30 m × 20 m has 5 m wide cemented path along its length and 8 m wide path along its width (as shown in figure). Find the cost of cementing the path at the rate of $200 per m².

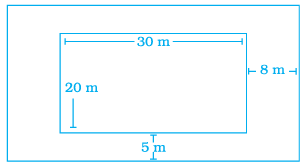
Solution :

Area of cementing path = Area of large rectangle - area of small rectangle

Length of large rectangle = 30 + 8 + 8

= 46 m

Width of the rectangle = 20 + 5 + 5

= 30 m

Area of large rectangle = 46 x 30

= 1380 m²

Area of small rectangle = 30 x 20

= 600 m²

Area of cementing path = 1380 - 600

= 780 m²

Amount to be spent = 780 x 200

= $156, 000

Problem 8 :

Find the area of the shaded portion.

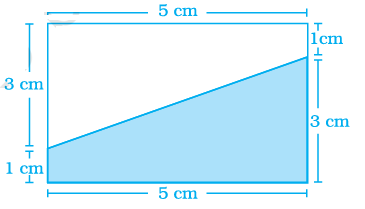
Solution :

Area of trapezium = (1/2) x height x (a + b)

height = 5 cm, a = 1 cm and b = 3 cm

= (1/2) x 5 x (1 + 3)

= (1/2) x 5 x 4

= 2 x 5

= 10 cm²

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.