AREA OF SCALENE TRIANGLE

Scalene triangle is a triangle with all sides of different lengths.

All angles are different, too.

So, no sides are equal and no angles are equal.

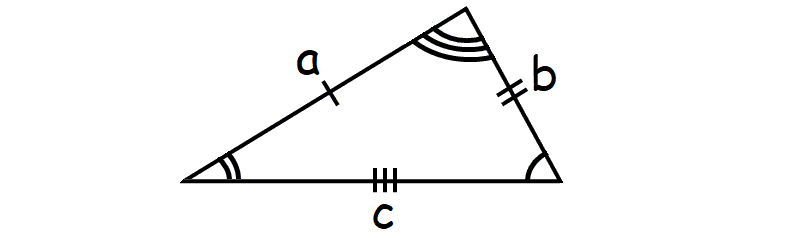
Formula for Area of Scalene Triangle : 

=  √[s(s - a)(s - b)(s - c)]

where

S  =  (a + b + c) / 2

Here a, b and c are side lengths of the triangle. 

Practice Problems

Problem 1 :

Find the area of the scalene triangle whose length of sides are 12 cm, 18 cm and 20 cm.

Solution :

Because the lengths of the three sides are different, the triangle is scalene triangle. 

S  =  (a + b + c) / 2

Substitute 12 for a, 18 for b and 20 for c. 

S  =  (12 + 18 + 20) / 2

S =  50/2

S  =  25

Formula for area of scalene triangle : 

=  √[s(s - a)(s - b)(s - c)]

Substitute. 

=  √[25 x (25 - 12) x (25 - 18) x (25 - 20)]

=  √(25 x 13 x 7 x 5)

=  5√455

So, area of the given scalene triangle is 5 √455 square cm.

Problem 2 :

The sides of a scalene triangle are 12 cm, 16 cm and 20 cm. Find the altitude to the longest side.

Solution :

In order to find the altitude to the longest side of a triangle, first we have to find the area of the triangle.

S  =  (a + b + c) / 2

Substitute 12 for a, 16 for b and 20 for c. 

S  =  (12 + 16 + 20) / 2

S =  48/2

S  =  24

Formula for area of scalene triangle : 

=  √[s(s - a)(s - b)(s - c)]

Substitute. 

=  √[24 x (24 - 12) x (24 - 16) x (24 - 20)]

=  √(24 x 12 x 8 x 4)

=  96 cm²

Because we want to find the altitude to the longest side, the longest side will be the base of the triangle as shown below.

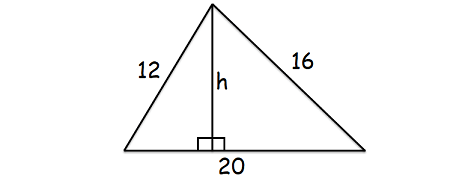
Here, the longest side is 20 cm.

Area of the above triangle  =  96 cm²

(1/2) x 20 x h  =  96

10h  =  96

Divide each side by 10.

h  =  9.6 cm

So, the altitude to the longest side is 9.6 cm.

Problem 3 :

The sides of a scalene triangle are in the ratio (1/2) : (1/3) : (1/4). If the perimeter is 52 cm, then find the length of the smallest side.

Solution :

From the given information, the sides the triangle are

x/2, x/3 and x/4

Perimeter of the triangle  =  52 cm

(x/2) + (x/3) + (x/4)  =  52

(6x + 4x + 3x) / 12  =  52

13x / 12  =  52  

13x  =  624

x  =  48

Then, 

x/2  =  24

x/3  =  16

x/4  =  12

So, the length of smallest side is 12 cm.

Problem 4 :

The area of the scalene triangle is 216 cm² and the sides are in the ratio 3 : 4 : 5. Find the perimeter of the triangle.

Solution :

From the given information, the sides the triangle are

3x, 4x and 5x

S  =  (3x + 4x + 5x) / 2

S  =  6x

Area of the triangle  =  216 cm²

√[s(s - a)(s - b)(s - c)]  =  216

√[6x(6x - 3x)(6x - 4x)(6x - 5x)]  =  216

√[6x(3x)(2x(x]  =  216

√(36x⁴)  =  216

6x²  =  216

x²  =  36

x  =  6

Then, 

3x  =  18

4x  =  24

5x  =  30

Perimeter of the given scalene triangle is 

=  18 + 24 + 30

=  72 cm

Problem 5 :

One side of a right angle scalene triangle is twice the other,and the hypotenuse is 10 cm. Find the area of the triangle.

Solution :

Let 'x' be the length of one of the legs of the triangle.

Then, the length of the other leg is 2x. 

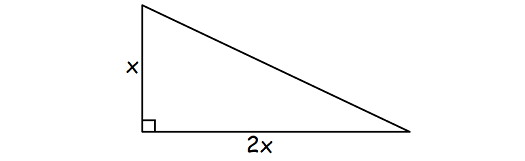
Using Pythagorean theorem, 

x² + (2x)²  =  10²

x² + 4x²  =  100

5x²  =  100

x²  =  20

√x²  =  √20

x  =  √(4 x 5)

x  =  2√5

Then, 

2x  =  2(2√5)

2x  =  4√5

Area of the given right angle scalene triangle is 

=  (1/2)(x) (2x)

=  (1/2)(2√5)(4√5)

=  20 cm²

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here. 

If you have any feedback about our math content, please mail us : 

v4formath@gmail.com

We always appreciate your feedback. 

You can also visit the following web pages on different stuff in math. 

WORD PROBLEMS

HCF and LCM  word problems

Word problems on simple equations 

Word problems on linear equations 

Word problems on quadratic equations

Algebra word problems

Word problems on trains

Area and perimeter word problems

Word problems on direct variation and inverse variation 

Word problems on unit price

Word problems on unit rate 

Word problems on comparing rates

Converting customary units word problems 

Converting metric units word problems

Word problems on simple interest

Word problems on compound interest

Word problems on types of angles 

Complementary and supplementary angles word problems

Double facts word problems

Trigonometry word problems

Percentage word problems 

Profit and loss word problems 

Markup and markdown word problems 

Decimal word problems

Word problems on fractions

Word problems on mixed fractrions

One step equation word problems

Linear inequalities word problems

Ratio and proportion word problems

Time and work word problems

Word problems on sets and venn diagrams

Word problems on ages

Pythagorean theorem word problems

Percent of a number word problems

Word problems on constant speed

Word problems on average speed 

Word problems on sum of the angles of a triangle is 180 degree

OTHER TOPICS 

Profit and loss shortcuts

Percentage shortcuts

Times table shortcuts

Time, speed and distance shortcuts

Ratio and proportion shortcuts

Domain and range of rational functions

Domain and range of rational functions with holes

Graphing rational functions

Graphing rational functions with holes

Converting repeating decimals in to fractions

Decimal representation of rational numbers

Finding square root using long division

L.C.M method to solve time and work problems

Translating the word problems in to algebraic expressions

Remainder when 2 power 256 is divided by 17

Remainder when 17 power 23 is divided by 16

Sum of all three digit numbers divisible by 6

Sum of all three digit numbers divisible by 7

Sum of all three digit numbers divisible by 8

Sum of all three digit numbers formed using 1, 3, 4

Sum of all three four digit numbers formed with non zero digits

Sum of all three four digit numbers formed using 0, 1, 2, 3

Sum of all three four digit numbers formed using 1, 2, 5, 6