AREA OF SQUARE
A square is a four-sided closed figure where the lengths of all the four sides will be equal and each vertex angle will be right angle or 90o as shown below.
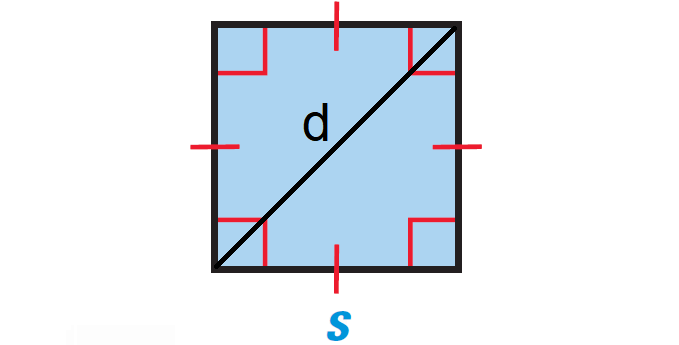
Formula for Area of a Square
Let s be the length of each side of a square.
Then, the formula for area of a square :
Area = s2
Let d be the length of each diagonal of a square.
Then, the formula for area of a square :
Area = 1/2 ⋅ d2
Examples
Example 1:
Find the area of the square having side length 24 cm.
Solution:
When the length of a side is given, formula for area of a square :
= s2
Substitute 24 for s.
= 242
= 576
So, area of the square is 576 square cm.
Example 2:
If the area of a square is 64 square inches, then find the length of each side.
Solution:
Area of the square = 64 in2
S2 = 64
Find positive square root on both sides.
√s2 = √(8 ⋅ 8)
S = 8
So, the length of each side of the square is 8 inches.
Example 3:
The square has side length 250 cm. Find its area in square meter.
Solution:
When the length of a side is given, formula for area of a square :
= s2
Substitute 250 for s.
= 2502
= 62500 cm2 -----(1)
We know
100 cm = 1 m
Square both sides.
(100 cm)2 = (1 m)2
1002 cm2 = 12 m2
10000 cm2 = 1 m2
Therefore, to convert centimeter square into meter square, we have to divide by 10000.
(1)-----> Area of the square = 62500 cm2
Divide the right side by 10000 to convert cm2 into m2.
Area of the square = (62500 / 10000) m2
= 6.25 m2
So, the area of the square is 6.25 square meter.
Example 4:
If the length of each diagonal is 2√2 cm, then find its area.
Solution:
When the length of a diagonal is given, formula for area of a square :
= 1/2 ⋅ d2
Substitute 2√2 for d.
= 1/2 ⋅ (2√2)2
Simplify.
= 1/2 ⋅ (4 ⋅ 2)
= 1/2 ⋅ (8)
= 4
So, the area of the square is 4 square cm.
Example 5:
If the lengths of the diagonals of two squares are in the ratio 2 : 5. then find the ratio of their areas.
Solution:
From the ratio 2 : 5, let the diagonals of two squares be 2x and 5x respectively.
When the length of a diagonal is given, formula for area of a square :
= 1/2 ⋅ d2
|
Area of 1st square = 1/2 ⋅ (2x)2 = 1/2 ⋅ (4x2) = 4x2 / 2 |
Area of 2nd square = 1/2 ⋅ (5x)2 = 1/2 ⋅ (25x2) = 25x2 / 2 |
Ratio of the areas :
= (4x2 / 2) : (25x2 / 2)
Multiply each term of the ratio by 2.
= 4x2 : 25x2
Divide each term by x2.
= 4 : 25
So, the ratio of the areas of two squares is 4 : 25.
Apart from the stuff given in this section, if you need any other stuff in math, please use our google custom search here.
If you have any feedback about our math content, please mail us :
v4formath@gmail.com
We always appreciate your feedback.
You can also visit the following web pages on different stuff in math.
WORD PROBLEMS
Word problems on simple equations
Word problems on linear equations
Word problems on quadratic equations
Area and perimeter word problems
Word problems on direct variation and inverse variation
Word problems on comparing rates
Converting customary units word problems
Converting metric units word problems
Word problems on simple interest
Word problems on compound interest
Word problems on types of angles
Complementary and supplementary angles word problems
Trigonometry word problems
Markup and markdown word problems
Word problems on mixed fractrions
One step equation word problems
Linear inequalities word problems
Ratio and proportion word problems
Word problems on sets and venn diagrams
Pythagorean theorem word problems
Percent of a number word problems
Word problems on constant speed
Word problems on average speed
Word problems on sum of the angles of a triangle is 180 degree
OTHER TOPICS
Time, speed and distance shortcuts
Ratio and proportion shortcuts
Domain and range of rational functions
Domain and range of rational functions with holes
Graphing rational functions with holes
Converting repeating decimals in to fractions
Decimal representation of rational numbers
Finding square root using long division
L.C.M method to solve time and work problems
Translating the word problems in to algebraic expressions
Remainder when 2 power 256 is divided by 17
Remainder when 17 power 23 is divided by 16
Sum of all three digit numbers divisible by 6
Sum of all three digit numbers divisible by 7
Sum of all three digit numbers divisible by 8
Sum of all three digit numbers formed using 1, 3, 4
Sum of all three four digit numbers formed with non zero digits
Recent Articles
-
Difference of Two Squares Worksheet
Oct 29, 20 12:21 AM
Difference of Two Squares Worksheet
-
Difference of Two Squares
Oct 28, 20 11:59 PM
Difference of Two Squares - Concept - Examples
-
Special Products of Binomials Worksheet
Oct 28, 20 11:53 PM
Special Products of Binomials Worksheet
