AREA BOUNDED BY THE GIVEN LINE X AXIS AND ORDINATES

Example 1 :

Find the area of the region bounded by the line

x-y = 1 

x - axis x = 2 and x = 4 

Solution :

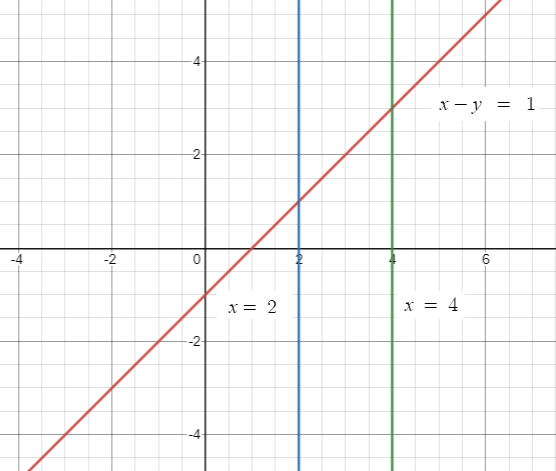
Within the limit x  =  2 and x  =  4, we find area under the given curve x - y  =  1.

The area lies above the x-axis, so the required area is

=  integral a to b y dx

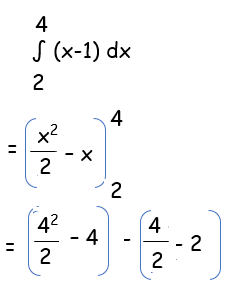
=  4 square units.

Example 2 :

Find the area of the region bounded by the line

x - y = 1 

x - axis, x = - 2 and x = 0

Solution :

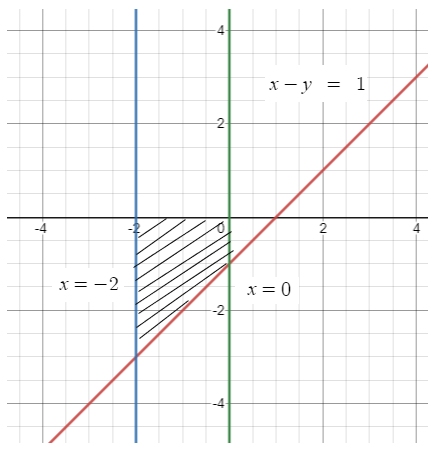
Required area = integral a to b ∫-y dx

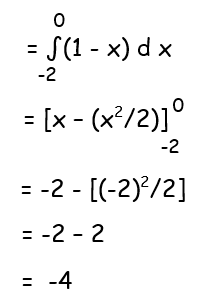
So, the required area is 4 square units.

Example 3 :

Find the area of the region by the line

x - 2y - 12 = 0

and y-axis, y = 2 and y = 5

Solution :

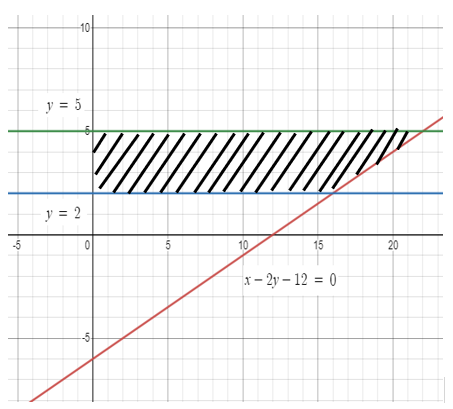
The area lies on the right side of y-axis. So,

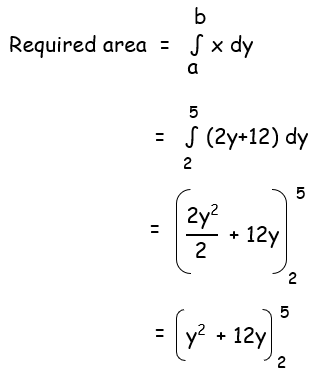
= (25 + 60) - (4 + 24)

= 85 - 28

= 57

So, the required area is 57 square units.

Example 4 :

Find the area of the region bounded by the line

y = x - 5

and the x - axis between the ordinates x = 3 and x = 7.

Solution :

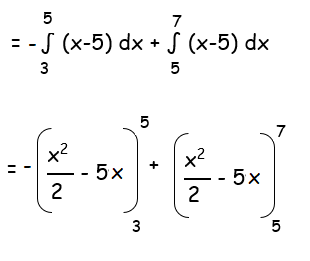
=  [-(25/2) + 25] + [(9/2)-15] + [(49/2)-35] - [(25/2)-25]

=  -12.5 + 25 + 4.5 - 15 + 24.5 - 35 - 12.5 + 25

=  -12.5 - 15 - 35 - 12.5 + 25 + 4.5 + 24.5 + 25

=  -75 + 54

=  21 square units.

Example 5 :

Find the area of the region bounded by

x² = 36y 

y - axis , y = 2 and y = 4

Solution :

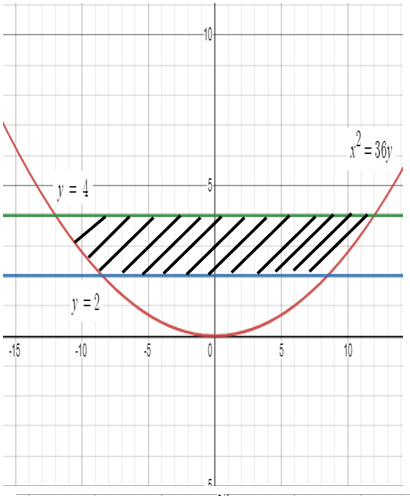
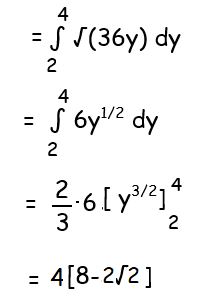
So, area of the shaded region is 8(4-√2) square units.

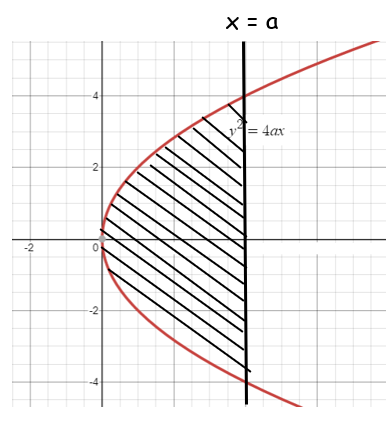
Example 6 :

Find the area included between the parabola

y² = 4ax

and its latus rectum.

Solution :

Example 7 :

Find the area of the region bounded by the ellipse

(x²/9) + (y²/5)  =  1

between the two latus rectum.

Solution :

a² = 9, b² = 5

e = √[1-(b²/a²)]

e = √[1-(5/9)]

= √[(9-5)/9]

= √(4/9)

e = 2/3

Equation of latus rectum x = ± ae

a = 3, e  =  2/3

ae = 3 (2/3)

ae = 2

Equation of latus rectum x = ± 2

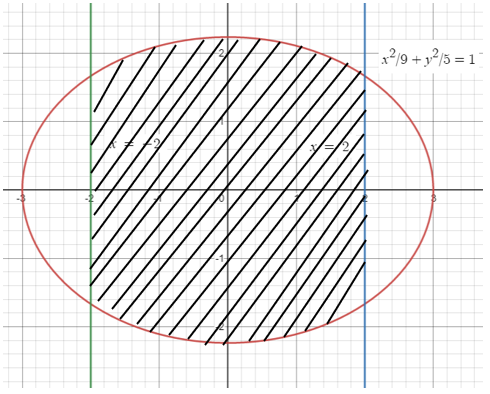
Required area  =  integral a to b ∫ y dx

(x²/9) + (y²/5)  =  1

(y²/5)  =  1 - (x²/9)

y²/5  =  (9 - x²)/9

y²  =  (5/9) (9-x²)

y  =  √(5/9) (9-x²)

y  =  √5/3 √(9-x²)

By using the limits x = 0 and x = 2,  we can find area above the x-axis.

To find the total shaded area we have to multiply the above area by 4.

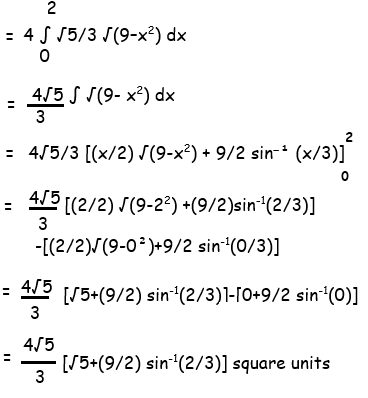
Example 8 :

Find the area of the region bounded by the parabola

y² = 4x

and the line

2x - y = 4

Solution : 

To find the point of intersection we have to solve both equations.

x  =  y²/4   ------ (1)

x  =  (4+y)/2 ------ (2)

(1)  =  (2)

y²/4 = (4 + y)/2

2y² = 4(4 + y)

2 y² = 16 + 4 y

2y² - 4 y - 16 = 0

now we are going to divide the whole equation by 2,

y² - 2y - 8 = 0

(y - 4) (y + 2) = 0

y - 4 = 0   y + 2 = 0

y = 4 and y  =  -2

Point of intersection of two curves are (0, 4) (0, -2).

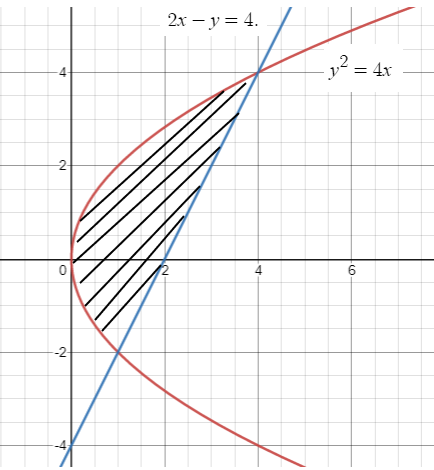
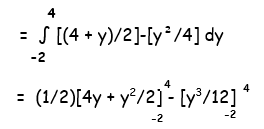
=  [(32+16)/4 - (64/12) ] - [ (-16 + 4)/4 - (-8/12) ]

=  [(48/4) - (16/3)] - [(-12/4) - (-8/12)]

=  [12-(16/3)] - [-3 + (2/3)]

=  [(36-16)/3)] - [(-9+2)/3]

=  [20/3] - [-7/3]

=  (20/3) + (7/3)

=  (20+7)/3

=  27/3

=  9 square units

Example 9 :

Find the area in the first quadrant bounded by 

f(x) = 4x - x²

and the x-axis.

Solution : 

f(x) = 4x - x²

Finding x-intercepts, we get

4x - x² = 0

x(4 - x) = 0

x = 0 and x = 4

= 2(4)² - 4³/3 - 0

= 2(16) - (64/3)

= 32 - (64/3)

= (96 - 64)/3

= 32/3

So, the required area is 32/3 square units.

Example 10 :

Find the area bounded by the following curves

f(x) = x² - 4, y = 0 and x = 4.

Solution : 

f(x) = x² - 4

y = x² - 4 ------(1)

y = 0 ------(2)

Finding points of intersecting of (1) and (2), we get

x² - 4 = 0

x² = 4

x = √4

x = ±2

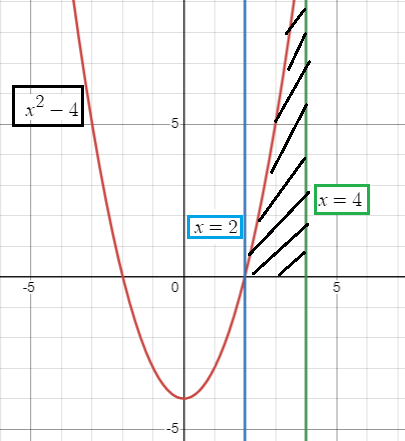
= (4³/3 - 4(4)) - (2³/3 - 4(2))

= (64/3) - 16 - 8/3 + 8

= (64/3) - (8/3) - 8

= (64 - 8)/3 - 8

= (56/3) - 8

= (56 - 24) / 3

= 32/3

So, the required area is 32/3 square units.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.