ARITHMETIC SERIES WORD PROBLEMS WITH ANSWERS

Question 1 :

A man repays a loan of 65,000 by paying 400 in the first month and then increasing the payment by 300 every month. How long will it take for him to clear the loan?

Solution :

For the first month he is paying  =  400

payment of second month  =  400 + 300  =  700

payment of 3rd month  =  700 + 300  =  1000

400 + 700 + 1000, ................

loan amount  =  65000

(n/2)[2a + (n - 1)d]  =  65000

(n/2)[2(400) + (n - 1)300]  =  65000

  (n/2)[800 + 300n - 300]  =  65000

 (n/2)[500 + 300n]  =  65000

(n/2)[5 + 3n]  =  650

n[5 + 3n]  =  1300

5n + 3n²  =  1300

3n² + 5n - 1300  =  0

3n² - 60n  + 65n - 1300  =  0

3n(n - 20) + 65(n - 20)  =  0

(n - 20) (3n + 65)  =  0

n = 20 or n = -65/3 (not acceptable)

So, he will clear the loan amount in 20 months.

Question 2 :

A brick staircase has a total of 30 steps. The bottom step requires 100 bricks. Each successive step requires two bricks less than the previous step.

(i) How many bricks are required for the top most step?

(ii) How many bricks are required to build the stair case?

Solution :

Let "l" be the number of bricks in the last step

Number of bricks in the 1st step (a)  =  100

Number of bricks in the 2nd step  =  100 - 2  =  98

d  =  98 - 100  =  -2

number of steps (n)  =  30

(i)

t₃₀  =  a + 29d

  =  100 + 29(-2)

  =  100 - 58

  =  42

So, we will have 42 bricks in the top most step.

(ii) How many bricks are required to build the stair case?

S_n  =  (n/2)[a + l]

  =  (30/2)[100 + 42]

  =  15(142) 

  =  2130

Question 3 :

If S_1, S₂, S₃,....Sm are the sums of n terms of m A.P.’s whose first terms are 1,2, 3,...m and whose common differences are 1, 3, 5,..., (2m -1) respectively, then show that S₁ + S₂ + S₃ +............S_m  =  (mn/2)(1 + mn)

Solution :

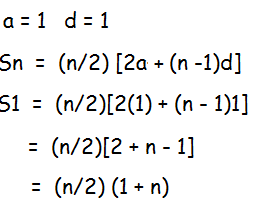
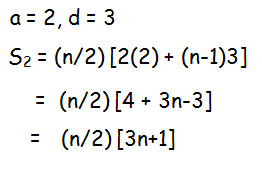
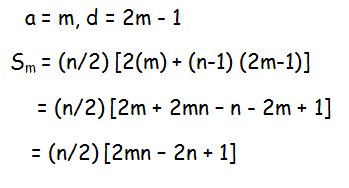
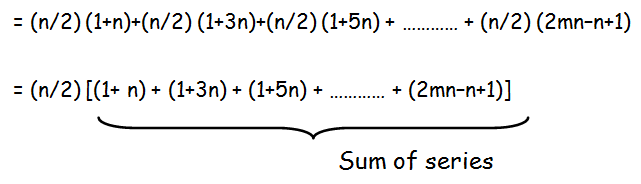
n = [(l-a)/d] + 1

 n  =  [((2mn - n + 1) - (1 + n))/2n] + 1

 n  =  [(2mn - n + 1 - 1 - n)/2n] + 1

n = [(2mn - 2n)/2n] + 1

n = (m - 1) + 1

n = m

 S₁ + S₂ + S₃ +............S_m  

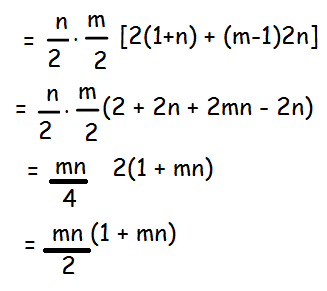
Hence proved.

Question 4 :

Find the sum

Solution :

a = (a-b)/(a+b)

d = (3a-2b)/(a+b) - (a-b)/(a+b)

  d = [3a - 2b -(a - b)]/(a + b) 

  d = [3a - 2b -a + b]/(a + b) 

  d  =  (2a - b) / (a + b)

Sn  =  (n/2)[2a + (n - 1)d]  

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.

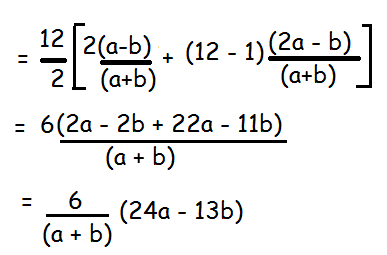
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.