AUXILIARY LINE
Auxiliary line (Helping line) is an extra line needed to complete a proof or problem in plane geometry.
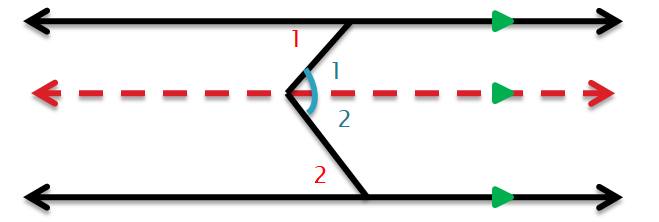
In the figure above, dotted line is an auxiliary line. Through any point there is exactly one line that will be parallel to an already existing line.
Proving the Triangle Sum Theorem Using an Auxiliary Line
Given : ΔABC
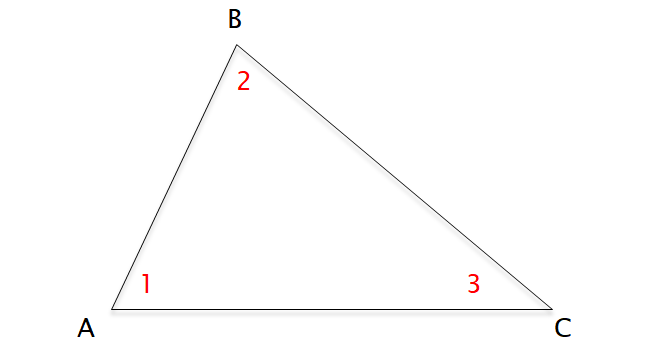
Prove : m∠1 + m∠2 + m∠3 = 180°
Recall : The sum of the interior angles of a triangle 180°.
Plane for the proof :
Draw an auxiliary line through B that is parallel to AC, name the new angles on this line m∠4 and m∠5.
Demonstrate that m∠4 + m∠2 + m∠5 = 180°,
m∠1 ≅ m∠4 and m∠3 ≅ m∠5
Use substitution to get
m∠1 + m∠2 + m∠3 = 180°
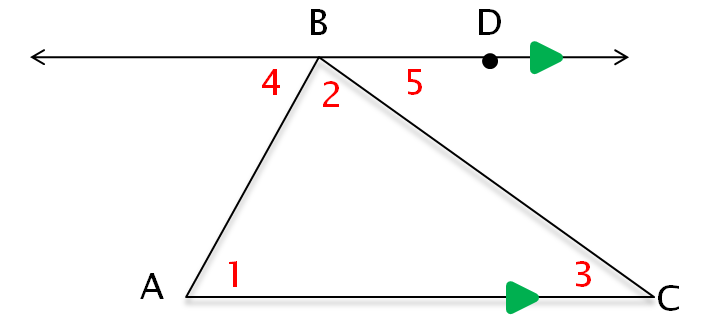
|
Statement : ΔABC Draw BD parallel to AC m∠4 + m∠2 + m∠5 = 180° |
Reason : Given Parallel postulate Angle Addition Postulate and Definition of a straight line. |
|
m∠1 ≅ m∠4, m∠3 ≅ m∠5 m∠1 = m∠4, m∠3 = m∠5 m∠1 + m∠2 + m∠3 = 180° |
AIA Theorem Definition of Congruence Substitution Property of Equality. |
Solved Problems
Problem 1 :
Using a 3rd parallel Line – Auxiliary Line, find the value of x.
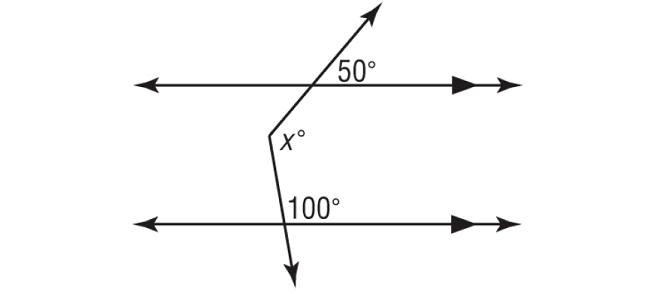
Solution :
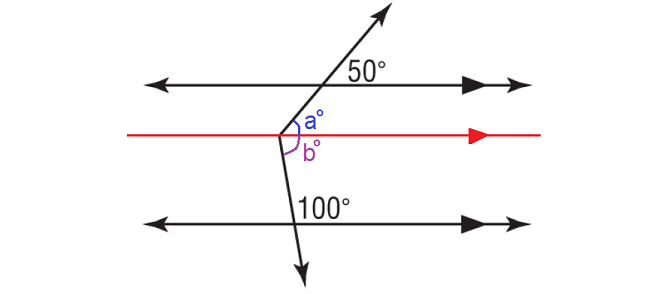
In the figure above, a° and 50° are corresponding angles and they are equal.
a° = 50°
b° and 105° are interior angles on the same side of the transversal and they are supplementary.
b° + 100° = 180°
Subtract 100° from each side.
b° = 80°
In the above figure,
x = a + b
= 50 + 80
= 130
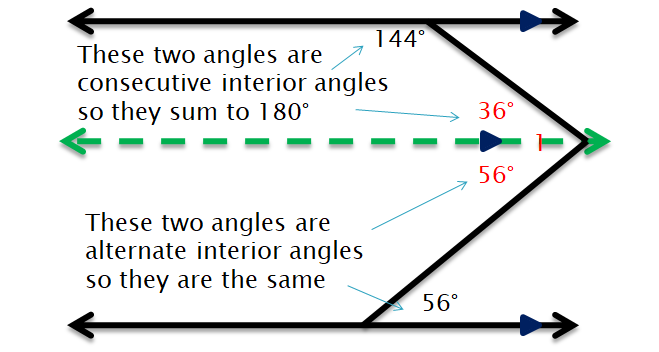
Problem 2 :
Using a 3rd parallel Line – Auxiliary Line, find the value of x.

Solution :
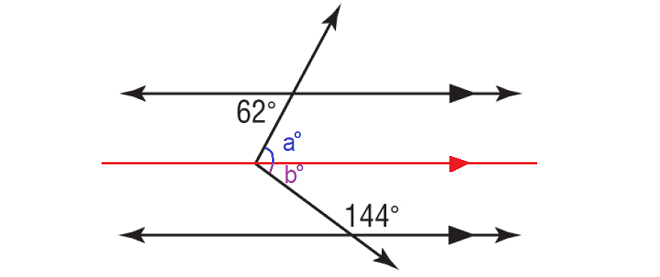
In the figure above, a° and 60° are alternate interior angles and they are equal.
a° = 62°
b° and 144° are interior angles on the same side of the transversal and they are supplementary.
b° + 144° = 180°
Subtract 144° from each side.
b° = 36°
In the above figure,
x = a + b
= 62 + 36
= 98
How do we use auxiliary lines to find missing angles? Compare and contrast diagrams a and b versus diagram c.
Problem 3 :
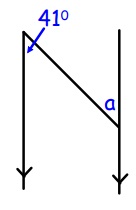
Solution :
Since the lines are parallel, alternate interior angles are equal.
a = 41
Problem 4 :
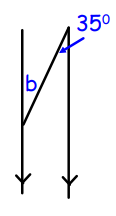
Solution :
Since the lines are parallel, alternate interior angles are equal.
b = 35
Problem 5 :
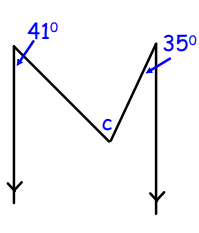
Solution :
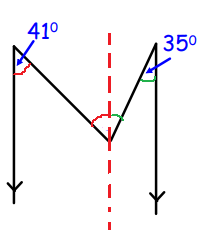
c = 41 + 35
= 76
So, the angle c is 76.
Problem 6 :
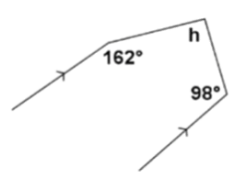
Solution :
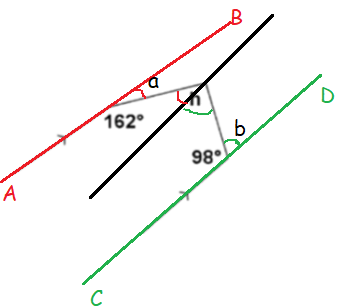
From the picture given, the lines AB and CD are parallel.
|
162 + a = 180 a = 180 - 162 a = 18 |
98 + b = 180 b = 180 - 98 b = 82 |
h = a + b
h = 18 + 82
h = 100
So, the value of h is 100 degree.
Problem 7 :
Find the value of the variable(s) in each figure. Explain your reasoning.

Solution :
By observing the picture above, the lines are parallel.
Sum of co-interior angles is equal to 180 degree.
x + 106 = 180
x = 180 - 106
x = 74 degree
Sum of angles of linear pair is 180 degree.
106 + 2y = 180
2y = 180 - 106
2y = 74
y = 74/2
y = 37
Corresponding angles are equal. Then,
4z + 6 = 2y
4z + 6 = 74
4z = 74 - 6
4z = 68
z = 68/4
= 17
So, the values of x, y and z are 74, 34 and 17 respectively.
Problem 8 :
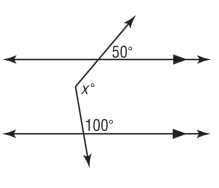
Solution :
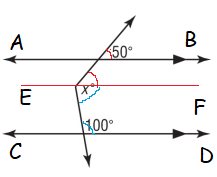
By observing the lines AB and CD are parallel.
EF is the auxiliary line.
- The angles marked using red color is co-interior angles. The sum of co-interior angles is equal to 180 degree.
So, the missing angle marked using red color is 130 (180 - 50) degree.
- The angles marked using blue color is co-interior angles. The sum of co-interior angles is equal to 180 degree.
So, the missing angle marked using blue color is 80 (180 - 100) degree.
x = 130 + 80
= 210
Problem 9 :
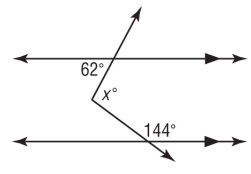
Solution :

By observing the lines AB and CD are parallel.
EF is the auxiliary line.
- The angles marked using red color is alternate interior angles. So, the missing angle using red color is 62 degree.
- The angles marked using green color is co-interior angles. The sum of co-interior angles is equal to 180 degree.
= 180 - 144
= 36
x = 62 + 36
x = 98
So, the value of x is 98 degree.
Problem 10 :
A diagonal brace strengthens the wire fence and prevents it from sagging. The brace makes a 50° angle with the wire as shown. Find the value of the variable
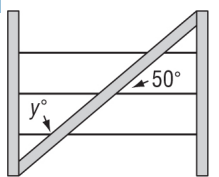
Solution :
So, missing angle is 50 degree
Problem 11 :
An auxillarv line is a new parallel line that can help us find unknown angles
Steps
1. Draw an auxiliary line through the unknown angle.
2. Use the properties of parallel lines to find the missing angles.
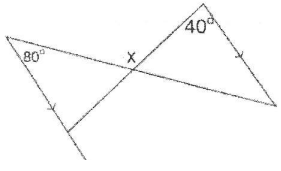
Solution :
Through x, we should draw the line.

Angles marked with red color = 40
Angle marked with green = 80
x = 40 + 80
= 120 degree.
So, the angle measure x is 120 degree.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 134)
Apr 02, 25 12:40 AM
Digital SAT Math Problems and Solutions (Part - 134) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Apr 02, 25 12:35 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part 135)
Apr 02, 25 12:32 AM
Digital SAT Math Problems and Solutions (Part 135)

