CARTESIAN PRODUCT OF TWO SETS

If A and B are two non-empty sets, then the set of all ordered pairs (a, b) such that "a" belongs to A and "b" belongs to B, is called the Cartesian product of A and B, to be denoted by A x B.

Thus,

A x B  =  {(a, b) : a ∈ A and b ∈ B}

And the Cartesian product of B and A, to be denoted by

B x A

Thus, 

B x A  =  {(b, a) : a ∈ A and b ∈ B}

And also,

A x B  ≠  B x A 

But, 

n(A x B)  =  n(B x A)

If A is null set or B is null set, we define that A x B is null set.  

The figure given below clearly illustrates the Cartesian product of two sets.

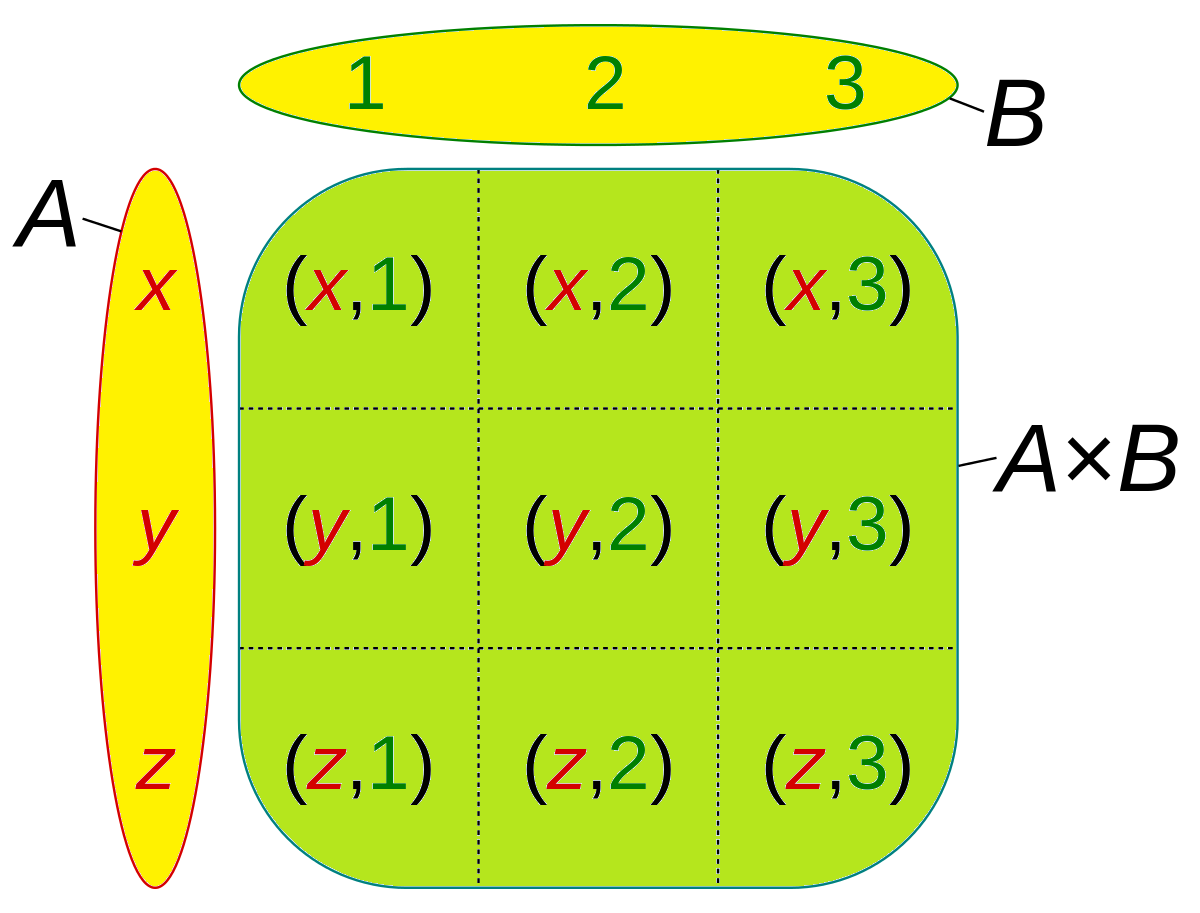
Solved Examples

Example 1 :

Let A  =  {1, 2, 3}, B  =  {4, 5}. Find A x B and B x A.

Solution : 

A x B  =  {(1, 4), (1, 5), (2, 4), (2, 5), (3, 4), (3, 5)}

B x A  =  {(4, 1), (4, 2), (4, 3), (5, 1), (5, 2), (5, 3)}

Example 2 :

If A x B  =  {(3, 2), (3, 4), (5, 2), (5, 4)}, find the sets A and B.

Solution : 

Clear, A is the set of all first co-ordinates of A x B, while B is the set of all second co-ordinates of elements of A x B. 

Therefore A  =  {3, 5} and B  =  {2, 4}

Example 3 :

Let P  =  {1, 3, 6}, Q  =  {3, 5}. Prove the following : 

P x Q  ≠  Q x P

n(P x Q)  =  n(Q x P)

Solution :

Product sets : 

P x Q  =  {(1, 3), (1, 5), (3, 3), (3, 5), (6, 3), (6, 5)}

Q x P  =  {(3, 1), (3, 3), (3, 6), (5, 1), (5, 3), (5, 6)}

Notice that

n(P)  =  3, n(Q)  =  2, n(P x Q)  =  6 and n(Q x P)  =  6

And also,

n(P x Q)  =  n(P) x n(Q)  

n(Q x P)  =  n(P) x n(Q)  

In Cartesian product, the ordered pairs (3, 5) and (5, 3) are not equal.

So,

P x Q  ≠  Q x P 

But,

n(P x Q)  =  n(Q x P)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.