CENTRAL ANGLES AND ARC MEASURES

1. A central angle is an angle with its vertex at the center of the circle and its two sides are radii. 

2. For example : m∠POQ is a central angle in circle P shown below. 

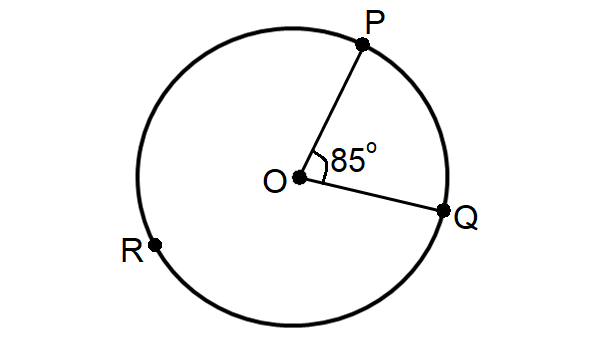
3. The sum of all central angle is 360°.

4. The measure of the arc formed by the endpoints of a central angle is equal to the degree of the central angle.

In the above diagram, 

m∠arc PQ = 85°

m∠arc PRQ = 360° - 85° = 275°

5. The measure of the arc formed by the endpoints of the diameter is equal to 180°.

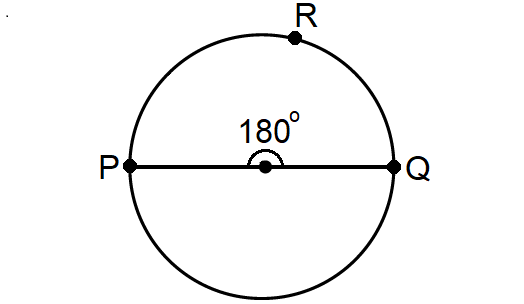
In the above diagram, 

m∠arc PRQ = 180°

Example 1 : 

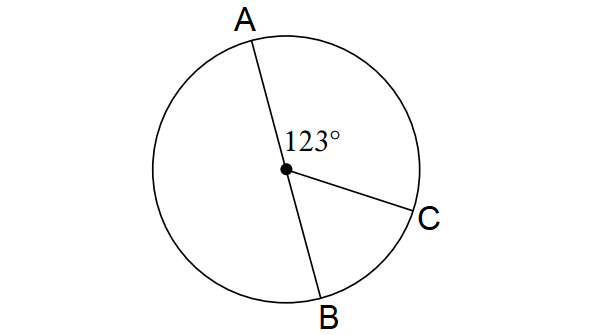
From the diagram shown above, find the following arc measures. 

(i) m∠arc BC

(ii) m∠arc ABC

Solution :

(i) m∠arc BC :

AB is the diameter of the above circle. 

m∠arc AB = 180°

m∠arc BC + m∠arc CA = 180°

m∠arc BC + 123° = 180°

m∠arc BC = 57°

(ii) m∠arc ABC :

m∠arc ABC = m∠arc AB + m∠arc BC

= 180° + 57°

= 237°

Example 2 :

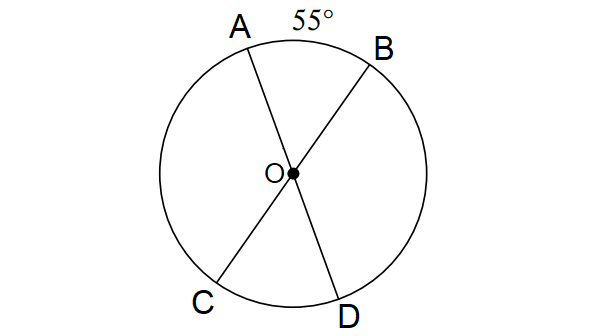
From the diagram shown above, find the following measures. 

(i) m∠arc CD

(ii) m∠AOC

(iii) m∠arc BD

(iv) m∠arc ABC

(v) m∠arc CBD

Solution :

(i) m∠arc CD :

m∠AOB and m∠COD are vertical angles. 

m∠COD = m∠AOB

m∠arc CD = m∠arc AB

m∠arc CD = 55°

(ii) m∠AOC :

BC is the diameter of the above circle. 

m∠arc BAC = 180°

m∠arc BA + m∠arc AC = 180°.

55° + m∠arc AC = 180°.

m∠arc AC = 125°.

m∠AOC = 125°.

(iii) m∠arc BD : 

m∠BOD and m∠AOC are vertical angles. 

m∠BOD = m∠AOC

m∠BOD = 125°

m∠arc BD = 125°

(iv) m∠arc ABC : 

m∠arc ABC = m∠arc ABD + m∠arc DC

= 180° + 55°

= 235°

(v) m∠arc CBD : 

m∠arc CBD = m∠arc CAB + m∠arc BD

= 180° + 125°

= 305°

Example 3 :

Find the value of x in the diagram shown below. 

From the diagram shown above, find the  m∠arc QTR.

Solution :

Find m∠arc QP :

PS is the diameter of the above circle.

m∠arc PTS = 180°

m∠arc PT + m∠arc TS = 180°

135° + m∠arc TS = 180°

m∠arc TS = 45°

Find m∠arc QTR :

m∠QTR = m∠arc QT + m∠arc TS + m∠arc SR

= 180° + 45° + 81°

= 306°

Example 4 :

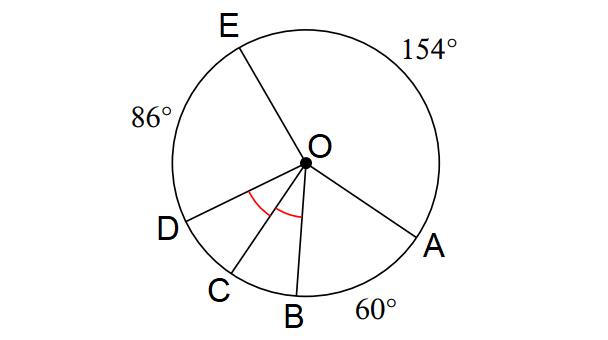
From the diagram shown above, find the following measures. 

m∠BOD, m∠BOE and m∠BOC

Solution :

Find m∠BOD :

In the circle above,

m∠arc AB + m∠arc BCD + m∠arc DE + m∠arc EA = 360°

60° + m∠arc BCD + 86° + 154° = 360°

m∠arc BCD + 300° = 360°

m∠arc BCD = 60°

m∠BOD = 60°

Find m∠BOE :

m∠BOE = m∠arc BCD + m∠arc DE

= 60° + 86°

= 146°

Find m∠BOC :

In the above diagram, m∠BOC = m∠COD.

m∠BOC + m∠COD = m∠BOD

m∠BOC + m∠BOC = m∠BOD

2m∠BOC = 60°

m∠BOC = 30°

Example 5 :

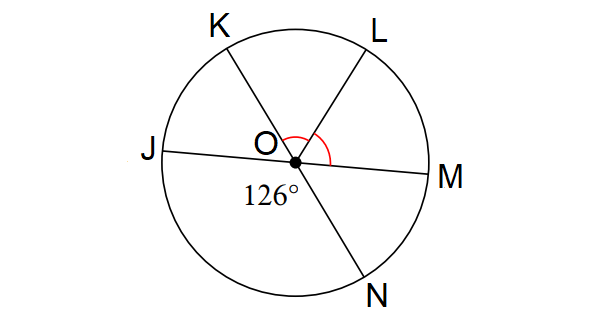
From the diagram shown above, find the following measures. 

m∠KOL and m∠arc MNK

Solution :

In the diagram above, m∠JON and ∠KOM are vertical angles.

m∠KOM = m∠KOM

m∠KOM = 126°

m∠KOL + m∠LOM = 126°

In the above diagram, m∠KOL = m∠LOM.

m∠KOL + m∠KOL = 126°

2m∠KOL = 126°

m∠KOL = 63°

Find m∠arc MNK :

m∠arc MNK = 360° - m∠arc KLM

m∠arc MNK = 360° - m∠KOM

m∠arc MNK = 360° - 126°

m∠arc MNK = 234°

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.