CIRCLES WORD PROBLEMS

Problem 1 :

The diameter of a cart wheel is 2.1 m. Find the distance traveled when it completes 100 revolutions.

Solution :

In order to find the distance covered in one revolution, we have to find the circumference of the circle.

Radius of wheel is 

=  2.1 / 2

=  1.05 m

Distance traveled when 100 revolutions are completed : 

=  100 x circumference of the wheel

=  100 x 2 x (22/7) x (1.05)

=  100 x 2 x 22 x 0.15

=  660 m

Problem 2 :

The diameter of a circular park is 98 m. Find the cost of fencing it at $4 per meter.

Solution :

Diameter of the circular park is 98 m.

Radius : 

=  98/2

=  49 m

Cost of fencing is $4 per meter. 

Length covered by fencing is equal circumference of the circular park.

Circumference of the park is 

=  2πr

=  2 (22/7) x 49

=  2 x 22 x 7

=  308 m

Cost for fencing is

=  308 x 4

=  $1232

Problem 3 : 

A wheel makes 20 revolutions to cover a distance of 66 m. Find the diameter of the wheel.

Solution :

Given :

A wheel makes 20 revolutions to cover a distance of 66 m. 

Then, the distance covered in one revolution is

=  66/20

=  33/10 m

The distance covered in one revolution is equal to circumference of the circle. 

Then,

2πr  =  33/10

2 x (22/7) x  r  =  33/10

(44/7) x r  =  33/10

Multiply each side by 44/7.

r  =  (33/10) x (7/44)

r  =  0.525

Diameter : 

=  2 x radius

=  2 x 0.525

=  1.05 m

Problem 4 :

The radius of a cycle wheel is 35 cm. How many revolutions does it make to cover a distance of 81.40 m ?

Solution :

Radius is given in centimeters and the distance is given in meters.

To have all the measures in meters, we can convert the given radius measure to meters.

Radius :

=  35 cm

=  35/100 m

=  0.35 m

Let 'n' be the number of revolutions required to cover the distance of 81.40 m. 

n x one revolution of cycle wheel  =  81.40

n x 2πr  =  81.40

n x 2 x (22/7) x 0.35  =  81.40

n x 2.2  =  81.40

Divide each side by 2.2

n  =  81.40/2.2

n  =  37

So, the cycle wheel has to revolve 37 times to cover the distance.

Problem 5 :

The radius of a circular park is 63 m. Find the cost of fencing it at $12 per metre.

Solution :

Radius of the circular park is 63 m.

Length of fencing is equal to circumference of the circular park.  

Circumference of the cicular park is 

=  2πr

=  2 x (22/7) x 63

=  2 x 22 x 9

=  396 m

Cost of fencing is $12 per meter. 

Total cost for fencing the park is

=  396 x 12

=  $4752

Problem 6 :

A goat is tethered by a rope 3.5 m long. Find the maximum area that the goat can graze.

Solution :

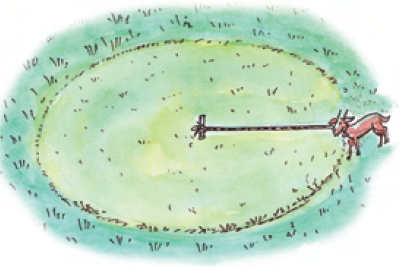
Radius of the circle is equal to length of the rope. 

Then, the radius is 

=  3.5 m

=  7/2 m

Area grazed by the goat is equal to the area of the circle with radius 7/2 m. 

Maximum area grazed by the goat is 

=  πr²

=  (22/7) x (7/2) x (7/2)

=  77/2

  =  38.5 sq. m

Example 7 :

The circumference of a circular park is 176 m. Find the area of the park.

Solution :

Circumference of the circular park is 176 m (given). 

Then, 

2πr  =  176

2 x (22/7) x r  =  176

(44/7) x  r  =  176

Multiply each side by 7/44.

r  =  176 x (7/44)

r  =  28 m

Area of the circular park is 

=  πr²

=  (22/7) x 28 x 28

= 22 x 4 x 28

= 2464 sq. m.

Problem 8 :

A silver wire when bent in the form of a square encloses an area of 121 sq. cm. If the same wire is bent in the form of a circle. Find the area of the circle.

Solution :

Area of the square is 121 sq. cm. (given)

Let a be the length of each side of the square.

Then, 

a²  =  121

a²  =  11²

a  =  11

Then, perimeter of the square is 

=  4a

= 4 x 11

= 44 cm

Length of the wire is equal to perimeter of the square. 

So, length of the wire is 44 cm.

If thee wire is bent in the form of a circle, then the circumference of the circle is equal to length of the wire

Circumference of a circle  =  44 cm

2πr  =  44

2 x (22/7) x r  =  44

(44/7) x r  =  44

Multiply each side by 7/44.

r  =  44 x (7/44)

r  =  7 cm

Area of the circle is

=  πr²

=  (22/7) x 7 x 7

=  154 sq. cm

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.