CIRCUMFERENCE AND ARC LENGTH

The circumference of a circle is the distance around the circle. 

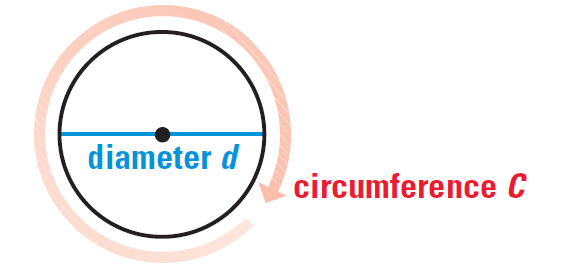
For all circles, the ratio of the circumference to the diameter is the same. This ratio is known as π, or pi.

An arc length is a portion of the circumference of a circle. We can use the measure of the arc (in degrees) to find its length (in linear units).

Circumference of a Circle

The circumference C of a circle is 

C  =  πd

or

C  =  2πr

where d is the diameter of the circle and r is the radius of the circle. 

Arc Length

In a circle, the ratio of the length of a given arc to the circumference is equal to the ratio of the measure of the arc to 360°. 

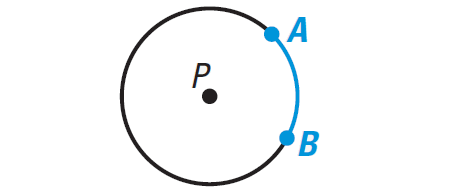
In the diagram shown above, we have

(Arc length of AB)/2πr  =  m∠arc AB/360°

or

Arc length of AB  =  [m∠arc AB/360°] ⋅ 2πr

Length of a Semicircle and 90° Arc

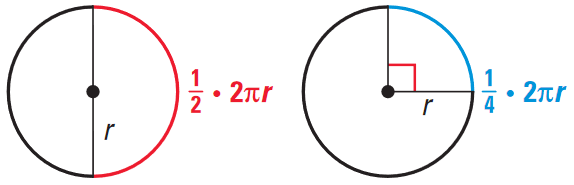
The length of a semicircle is one half the circumference, and the length of a 90° arc is one quarter of the circumference.

Finding Circumference

Example 1 :

Find the circumference of a circle with radius 6 centimeters. Round decimal answers to two decimal places.

Solution :

The formula for circumference of a circle is given by 

C  =  2πr

Plug r  =  6.

C  =  2π(6)

C  =  12π

Use calculator to get the value of π.

C  ≈  37.70

Hence, the circumference is about 37.70 centimeters.

Using Circumference

Example 2 :

Find the radius of a circle with circumference 31 meters. Round decimal answers to two decimal places.

Solution :

The formula for circumference of a circle is given by 

C  =  2πr

Plug C  =  31.

31  =  2πr

Divide each side by 2π.

31/2π  =  r

Use calculator to get the value of π.

4.93  ≈  r

Hence, the radius is about 4.93 meters. 

Finding Arc Lengths

Example 3 :

Find the length of the arc AB in the diagram shown below. 

Solution :

Formula for length of the arc :  

Arc length of AB  =  [m∠arc AB/360°] ⋅ 2πr

Substitute. 

Arc length of AB  =  [50°/360°] ⋅ 2π(5)

Simplify. 

Arc length of AB  ≈  4.36 centimeters

Example 4 :

The track shown below has six lanes. Each lane is 1.25 meters wide. There is a 180° arc at each end of the track. The radii for the arcs in the first two lanes are given.

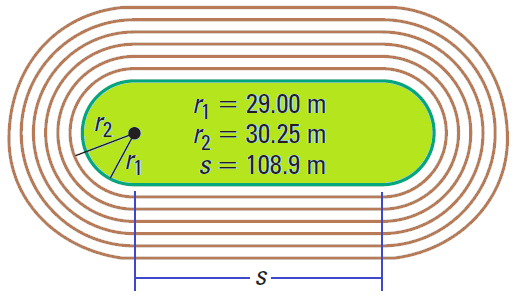
a.  Find the distance around Lane 1.

b.  Find the distance around Lane 2.

Solution :

The track is made up of two semicircles and two straight sections with length s.To find the total distance around each lane, find the sum of the lengths of each part.Round decimal answers to one decimal place.

Using Arc Lengths

Example 5 :

Find the circumference of the circle shown below. 

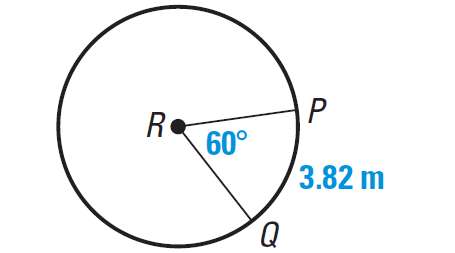
Solution :

(Arc length of PQ)/2πr  =  m∠arc PQ/360°

3.82/2πr  =  60°/360°

3.82/2πr  =  1/6

Take reciprocal on each side. 

2πr/3.82  =  6/1

2πr/3.82  =  6

Multiply each side by 3.82

2πr  =  6(3.82)

2πr  =  6(3.82)

2πr  =  22.92

Hence, the circumference of the circle is about 22.92 meters. 

Example 6 :

Find the m∠arc XY. 

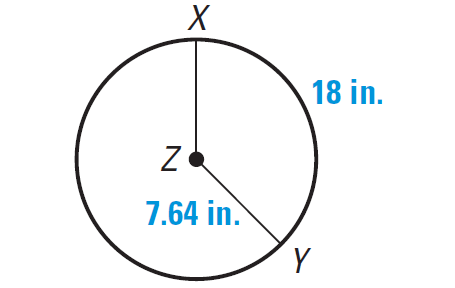
Solution :

(Arc length of XY)/2πr  =  m∠arc XY/360°

Substitute. 

18/2π(7.64)  =  m∠arc XY/360°

18/15.28π  =  m∠arc XY/360°

Multiply each side by 360°.

360° ⋅ 18/2π(7.64)  =  m∠arc XY

135°  ≈  m∠arc XY

Hence, m∠arc XY is about 135°.

Comparing Circumferences

Example 7 :

Tires from two different automobiles are shown below. How many revolutions does each tire make while traveling 100 feet?Round decimal answers to one decimal place.

Solution :

Tire A has a diameter of 14 + 2(5.1), or 24.2 inches. Its circumference is π(24.2), or about 76.03 inches.

Tire B has a diameter of 15 + 2(5.25), or 25.5 inches. Its circumference isπ(25.5), or about 80.11 inches.

Divide the distance traveled by the tire circumference to find the number of revolutions made.

First convert 100 feet to 1200 inches.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.