CLASS 9 MATH WORKSHEET WITH SOLUTION

Problem 1 :

Solve the given equation below x + 2 log₂₇ 9  =  0

(A)  -4/3     (B)  1/2     (C)  2/5

Solution :

x + 2log₂₇ 9  =  0

x  =  -2log₂₇ 9

x  =  log₂₇ 9^-2

We know that,

If x  =  log_a b then a^x  =  b

27^x  =  9^-2

(3³)^x  =  (3²)^-2

3^3x  =   3^-4 

If b^x  = b^y then x  =  y

3x  =  -4

x  =  -4/3

So, the answer is -4/3.

Problem 2 :

In the given, ∠A = 64° , ∠ABC = 58°. If BO and CO are the bisectors of ∠ABC and ∠ACB respectively of ΔABC, find x° and y°

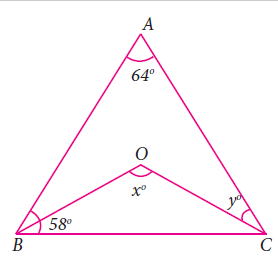
Solution :

Since BO and CO are bisectors of ABC and ACB.

<OBC  =  58/2  =  29

In triangle ABC,

<ABC + <BAC + <BCA  =  180

58 + 64 + <BCA  =  180

<BCA  =  180 - 122

<BCA  =  58

<y  =  58

In triangle OBC,

<OBC + <BOC + <BCO  =  180

29 + <BOC + 29  =  180

<BOC  =  180 - 58

x  =  <BOC  =  122

Problem 3 :

Find the number of subsets for the set A  =  {1, 2, 3, 4, 5}

(A)  11   (B)  22   (C)  32

Solution :

Given, A  =  {1, 2, 3, 4, 5}

Here, A contains 5 elements.

So, n  =  5

The number of subsets for A  =  2ⁿ

=  2⁵

=  32

So, the answer is 32.

Problem 4 :

{The number of engineering colleges in Singapore}

The above set is a _--------

(A)  singleton set   (B)  infinite set   (C)  finite set 

Solution :

So, the answer is finite set.

Problem 5 :

The cardinal number of the set P {0}

(A)  3   (B)  4   (C)  1 

Solution :

We know that,

The cardinal number of the set P {0} is n(P)

Here, P contains 1 element.

So, the n(P) is 1.

Problem 6 :

If A  =  {1, 2, 3} and B  =  {2, 3, 4}, find A n B.

(A)  {1, 2, 3}   (B)  {2, 3}   (C)  {1, 2, 3, 4} 

Solution :

Given, A  =  {1, 2, 3} and B  =  {2, 3, 4}

Find A n B.

The common elements in sets A and B is A n B  =  {2, 3}

So, the answer is {2, 3}

Problem 7 :

The equation x + 3y  =  12, 3x + 9y  =  24 has _------ solution.

(A)  unique   (B)  infinite   (C) no 

Solution :

By writing the given equations a₁x + b₁y + c₁  =  0 and a₂x + b₂y + c₂  =  0 in the form, we get

x + 3y - 12  =  0

3x + 9y - 24  =  0

From the equations, let us find the values of a₁, a₂, b₁, b₂, c₁, and c₂

Here a₁  =  1, b₁  =  3 and c₁  =  -12

a₂  =  3, b₂  =  9 and c₂  =  -24

a₁/a₂  =  1/3 -----(1)

b₁/b₂  =  3/9  =  1/3 -----(2)

c₁/c₂  =  12/24  =  1/2 -----(3)

 (1) = (2) ≠ (3)

Now, a₁/a₂ = b₁/b₂ ≠ c₁/c₂

So, it has no solution.

Problem 8 :

Solve 5x + 3y  =  11, 3x + 5y  =  -3 

(A)  (1, 2)   (B)  (0, 3)   (C) (4, -3) 

Solution :

5x + 3y  =  11 -----(1)

3x + 5y  =  -3 -----(2)

Using elimination method :

Subtract 3(1) - 5(2), we get

15x + 9y - 15x - 25y  =  33 + 15

9y - 25y  =  48

-16y  =  48

y  =  -3

By applying y  =  -3 in equation (1), we get

5x + 3(-3)  =  11

5x - 9  =  11

5x  =  20

x  =  4

So, the solution is (4, -3)

Problem 9 :

Find the sum of 2x⁴ - 3x² + 5x + 3 and 4x + 6x³ - 6x² - 1

(A)  2x⁴ + 6x³ - 9x² + 9x + 2

(B)   4x⁴ + 6x³ - 9x² - 9x 

(C)  -2x⁴ + 6x³ + 9x² - 9x + 2

Solution :

By adding, we get

=  2x⁴ - 3x² + 5x + 3 + 4x + 6x³ - 6x² - 1

=  2x⁴ + 6x³ - 9x² + 9x + 2

So, the answer is 2x⁴ + 6x³ - 9x² + 9x + 2

Problem 10 :

If a/b  =  5 then the value of (a - b)/(a + b) is

(A)  1/8   (B)  2/3   (C)  3/4 

Solution :

Given, a/b  =  5/1

Here a  =  5 and b  =  1

Then,

(a - b)/(a + b)  =  (5 - 1)/(5 + 1)

=  4/6

(a - b)/(a + b)  =  2/3

So, the answer is 2/3.

Apart from the stuff given above,  if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.