CLASSIFYING NUMBERS RATIONAL AND IRRATIONAL

Even though we can classify real numbers in many ways, it can be classified into two major categories. 

They are

(i) Rational numbers

(ii) Irrational numbers

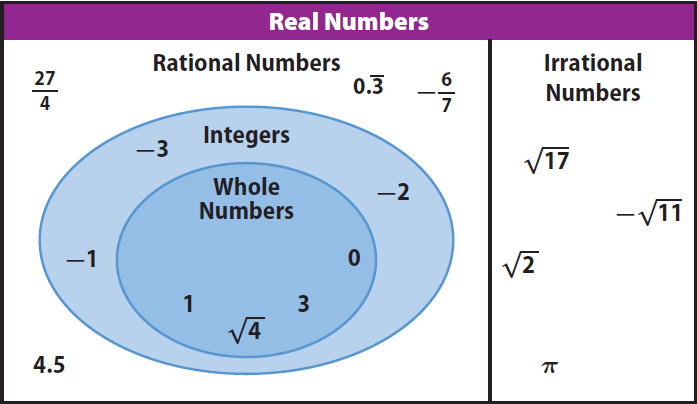
We already know that the set of rational numbers consists of whole numbers, integers, and fractions. 

So, the set of real numbers consists of the set of rational numbers and the set of irrational numbers.

It has been illustrated in the picture given below.

Solved Questions

Questions 1 : 

Write the name that apply to the number given below. 

√5

Answer :

5 is in square root. It is a whole number, but it is not a perfect square. 

So, √5 is irrational. 

Question 2 : 

Write the name that apply to the number given below. 

-16.28

Answer :

–16.28 is a terminating decimal.

So, -16.28 is rational.

Question 3 : 

Write the name that apply to the number given below. 

√81/9

Answer :

Let us do the possible simplification in the given number.

√81/9  =  9/9 

√81/9  =  1

So, √81 / 9 is whole, positive integer, integer, rational.

Question 4 : 

Write the name that apply to the number given below. 

-9

Answer :

-9 is negative integer, integer, rational.

Question 5 : 

Write the name that apply to the number given below. 

9

Answer :

9 is whole, positive integer, integer, rational.

Question 6 : 

Write the name that apply to the number given below. 

2√3

Answer :

We have 3 in square root. 3 is a whole number, but it is not a perfect square. 

So, √3 is irrational. 

We already know the fact, if an irrational number is multiplied by a rational number, the product is irrational. 

So, 2√3 is irrational.

Question 7 : 

Write the name that apply to the number given below. 

√25

Answer :

25 is in square root. 25 is a whole number and also it is a perfect square. 

So, we have

√25  =  √(5x5)  =  5

So, √25 is whole, positive integer, integer, rational.

Question 8 : 

Write the name that apply to the number given below. 

√250

Answer :

250 is in square root. We are not sure whether 250 is a perfect square or not. 

So, let us simplify the given number. 

√250  =  √(5x5x5x2)

√250  =  5√(5x2)

√250  =  5√10

We have 10 in square root. 10 is a whole number, but it is not a perfect square. 

So, √10 is irrational. 

We already know the fact, if an irrational number is multiplied by a rational number, the product is irrational. 

Therefore, √250 is irrational.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.