COMBINED FIGURES WORKSHEET
Question 1 :
Find the perimeter and area of the shape shown below. (Use π ≈ 3.14).
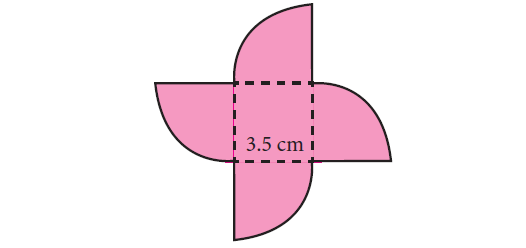
Question 2 :
Jennifer has a key-chain which is in the form of an equilateral triangle and a semicircle attached to a square of side 5 cm as shown below. Find its area. (Use π ≈ 3.14 and √3 = 1.732).

Question 3 :
A 3-fold invitation card is given with measures as shown below. Find its area.
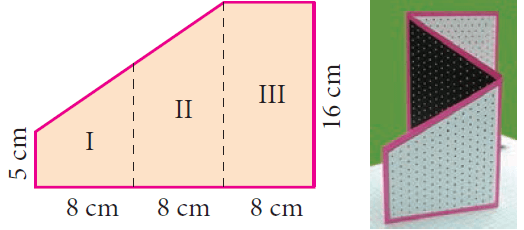
Question 4 :
A 3-fold invitation card is given with measures as shown below. Find its area.

Question 5 :
Find the area of the shaded region in the square of side 10 cm as shown below (Use π ≈ 3.14).

Question 6 :
Find the area of an irregular polygon field whose measures are as shown below.


1. Answer :

Radius of a circular quadrant, r = 3.5 cm and side of a square, a = 3.5 cm.
The given figure is formed by the joining of 4 quadrants of a circle with each side of a square. The boundary of the given figure consists of 4 arcs and 4 radii.
Perimeter of the given combined shape :
|
= 4 x length of the arcs of the quadrant of a circle |
+ 4 x radius |
= (4 x 1/4 x 2πr) + 4r
= (1 x 2 x 3.14 x 3.5) + 4(3.5)
= 21.98 + 14
= 35.98 cm (approximately)
Area of the given combined shape :
|
= area of the square |
+ 4 x area of the quadrants of the circle |
= a2 + (4 x 1/4 x πr2)
= (3.5 x 3.5) + (1 x 3.14 x 3.5 x 3.5)
= 12.25 + 38.465
= 50.715
= 50.72 cm2 (approximately)
2. Answer :

Side of the square = 5 cm
Diameter of the semi-circle = 5 cm ⇒ Radius = 2.5 cm
Side of the equilateral triangle = 5 cm
Area of the key-chain :
= area of the semi circle
+ area of the square
+ area of the equilateral triangle
= (1/2)πr2 + a2 + (√3/4)a2
= (0.5 x 3.14 x 2.5 x 2.5) + (5 x 5) + [(1.732/4) x 5 x 5]
= 9.8125 + 25 + 10.825
= 45.64 cm2 (approximately)
3. Answer :

Figures I and II are trapeziums separately as well as combinedly.
The parallel sides of the combined trapezium (I and II) are 5 cm and 16 cm and its height, h = 8 + 8 = 16 cm, length of the rectangle (III) = 16 cm and its breadth = 8 cm.
Area of the combined invitation card :
= area of the combined trapezium + area of the rectangle
= (1/2) x h x (a + b) + l x w
= 0.5 x 16 x (5 + 16) + 16 x 8
= 8 x 21 + 128
= 168 + 128
= 296 cm2
4. Answer :

The mat given in the figure can be split into two rectangles as follows :

Area of the entire mat :
= area of the I rectangle + area of the II rectangle
= l1 x w1 + l2 x w2
= 5 x 2 + 9 x 2
= 10 + 18
= 28 ft2
Cost per square foot = $20.
The total cost of the entire mat = 28 x $20 = $560.
5. Answer :
Mark the unshaded parts of the given figure as I, II, III and IV
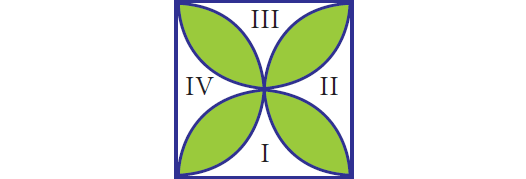
Area of the I and III parts :
= Area of the square – Area of 2 semicircles
= a2 - 2 x (1/2) x πr2
= 10 x 10 - 2 x 0.5 x 3.14 x 5 x 5
= 100 - 78.5
= 21.5 cm2
Similarly, the area of the II and IV parts = 21.5 cm2.
Area of the unshaded parts (I, II, III and IV) :
= 2 x 21.5
= 43 cm2 (approximately)
Area of the shaded part :
= area of the square – area of the unshaded parts
= 100 – 43
= 57 cm2 (approximately)
6. Answer :

The given field has four triangles (I, III, IV and V) and a trapezium.
Area of triangle (I) :
= (1/2) x b x h
= 0.5 x 5 x 6
= 15 m2
Area of trapezoid (II) :
= (1/2) x h x (a + b)
= 0.5 x 13 x (6 + 4)
= 0.5 x 13 x 10
= 65 m2
Area of triangle (III) :
= (1/2) x b x h
= 0.5 x 8 x 4
= 16 m2
Area of triangle (IV) :
= (1/2) x b x h
= 0.5 x 13 x 10
= 65 m2
Area of triangle (V) :
= (1/2) x b h
= 0.5 x 13 x 10
= 65 m2
The total area of the field :
= 15 + 65 + 16 + 65 + 65
= 226 m2
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Time and Work Problems
Oct 20, 25 07:13 AM
Time and Work Problems - Concept - Solved Problems -
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29)
