COMBINING LIKE TERMS WORKSHEET

Problem 1 :

Add (3x² + 7x - 2) and  (x² -  4x + 5).

Problem 2 :

Add (7y³ - 5y² + 1) and (2y² - y - 7).

Problem 3 :

Add (2p - q), (p + 3q) and (p - q).

Problem 4 :

Subtract 3ab from 7ab.

Problem 5 :

Subtract (2x + 3y²) from (5x - 2y²).

Problem 6 :

Add (a³ + 3a² - 5a - 2) and (a³ - 6a² + 9a - 8).

Problem 7 :

Add (2x³ + 5x² - 2x + 7) and (x³ + 4x² - x + 6).

Problem 8 :

Subtract (3x³ - 5y² + 1) from (4x³ - 2y² + 1).

Problem 9 :

Simplify :

(3x³ - 2x² - x + 4) + (2x³ + 7x² - 3x - 3)

Problem 10 :

Simplify :

(y³ + 2y² - 3y + 1) + 3(2y⁵ - 7y⁴ + 2y - 5)

Problem 11 :

Simplify :

-2(p⁵ + 2p⁴ - p² + 5) + 3(4p⁶ - 4p⁴ - 1)

Problem 12 :

Simplify :

5(5x⁶ + 2x³ - 6x² - 2) + 6(-3x⁶ + 2x⁵ + 2x + 1).

Problem 13 :

Simplify : 

-2(2x⁴ - 2x³ - x² + 5) + 3(2x⁴ - 2x² - 3).

Problem 14 :

Simplify :

5(x⁴ - x³ + 5) + 2(x⁴ - 5x² - 7).

Problem 15 :

Simplify :

3(6x⁴ - 2x³ - 3) + 2(2x⁴ - x² - 8).

Problem 16 :

Simplify :

(6x⁷ - 2x⁶ - 3x³ + 2x²) + 2(2x⁴ + 5x⁷ + 3x⁶ + x³ + x²)

Problem 17 :

Simplify :

(x⁷ - 3x⁶ - 2x³ + x²) + 5(3x⁴ + 15x⁷ + 4x⁶ + 2x³ + 6x²)

Problem 18 :

Subtract (2x³ + 5x² - 2x - 11) from (3x³ - 2x² - 5x - 6).

Problem 19 :

Subtract (x³ + 4x² - 12x - 5)  from (5x³ + 3x² + 2x - 10).

Problem 20 :

Subtract (2x³ - 5x² + 7x - 3) from 3(5x³ - 2x + 8).

Problem 21 :

Subtract 2(y² + 3y - 1) from 5(2y³ - 2y² - 7y - 4).

Problem 22 :

Simplify :

(x² + y²) - (x + y)(x - y)

Problem 23 :

Simplify :

(p - q)³ - (p + q)³

Answers

1. Answer :

= (3x² + 7x - 2) + (x² -  4x + 5)

= 3x² + 7x - 2 + x² -  4x + 5

Group the like terms together.

= (3x² + x²) + (7x - 4x)  + (-2 + 5)

Combine like terms.

= 4x² + 3x + 3

2. Answer :

= (7y³ - 5y² + 1) + (2y² - y - 7)

= 7y³ - 5y² + 1 + 2y² - y - 7

Group the like terms together.

= 7y³ + (-5y² + 2y²) - y + (1 - 7)

Combine the like terms.

= 7y³ + (-3y²) - y + (-6)

= 7y³ - 3y² - y - 6

3. Answer :

= (2p - q) + (p + 3q) + (p - q)

= 2p - q + p + 3q + p - q

Group the like terms together.

= (2p + p + p) + (-q + 3q - q)

Combine the like terms.

= 4p + q

4. Answer :

= 7ab - 3ab

Both the terms in the above expression are like terms. So, we can combine them.

= 4ab

5. Answer :

= (5x - 2y²) - (2x + 3y²)

Distribute the negative sign.

= 5x - 2y² - 2x - 3y²

Group the like terms together.

= (5x - 2x) + (-2y² - 3y²)

Combine the like terms.

= 3x + (-5y²)

= 3x - 5y²

6. Answer :

= (a³ + 3a² - 5a - 2) + (a³ - 6a² + 9a - 8)

= a³ + 3a² - 5a - 2 + a³ - 6a² + 9a - 8

Group the like terms together.

= (a³ + a³) + (3a² - 6a²) + (-5a + 9a) + (-2 - 8)

Combine the like terms.

= 2a³ + (-3a²) + 4a + (-10)

= 2a³ - 3a² + 4a - 10

7. Answer :

= (2x³ + 5x² - 2x + 7) + (x³ + 4x² - x + 6)

= 2x³ + 5x² - 2x + 7 + x³ + 4x² - x + 6

Group like terms together.

= (2x³ + x³) + (5x² + 4x²) + (-2x - x) + (7 + 6)

Combine like terms.

= 3x³ + 9x² - 3x + 13

8. Answer :

= (4x³ - 2y² + 1) - (3x³ - 5y² + 1)

Distribute the negative sign.

= 4x³ - 2y² + 1 - 3x³ + 5y² - 1

Group the like terms together.

= (4x³ - 3x³) + (-2y² + 5y²) + (1 - 1)

Combine the like terms.

= x³ + 3y² + 0

= x³ + 3y²

9. Answer :

= (3x³ - 2x² - x + 4) + (2x³ + 7x² - 3x - 3)

= 3x³ - 2x² - x + 4 + 2x³ + 7x² - 3 x - 3

Group like terms together.

= (3x³ + 2x³) + (-2x² + 7x²) + (-x - 3x) + (4 - 3)

Combine like terms.

= 5x³ + 5x² - 4x + 1

10. Answer :

= (y³ + 2y² - 3y + 1) + 3(2y⁵ - 7y⁴ + 2y - 5)

Using Distributive Property,

= (y³ + 2y² - 3y + 1) + 3(2y⁵ - 7y⁴ + 2y - 5)

= y³ + 2y² - 3y + 1 + 3(2y⁵) + 3(-7y^4)^ + 3(2y) + 3(-5)

= y³ + 2y² - 3y + 1 + 6y⁵ - 21y^4 + 6y - 15

= 6y⁵ - 21y⁴ + y³ + 2y² + (-3y + 6y) + (1 - 15)

= 6y⁵ - 21y⁴ + y³ + 2y² + 3y + (-14)

= 6y⁵ - 21y⁴ + y³ + 2y² + 3y - 14

11. Answer :

= -2(p⁵ + 2p⁴ - p² + 5) + 3(4p⁶ - 4p⁴ - 1)

= -2(p⁵) - 2(2p⁴) - 2(-p²) - 2(+5) + 3(4p⁶) + 3(-4p⁴) + 3(-1)

= -2p⁵ - 4p⁴ + 2p² - 10 + 12p⁶ - 12p⁴ - 3

= 12p⁶ - 2p⁵ + (-4p⁴ - 12p⁴) + 2p² + (-10 - 3)

= 12p⁶ - 2p⁵ + (-16p⁴) + 2p² + (-13)

= 12p⁶ - 2p⁵ - 16p⁴ + 2p² - 13

12. Answer :

= 5(5x⁶ + 2x³ - 6x² - 2) + 6(-3x⁶ + 2x⁵ + 2x + 1)

Distributive Property.

= 25x⁶ + 10x³ - 30x² - 10 - 18x⁶ + 12x⁵ + 12x + 6

Group like terms together.

= (25x⁶ - 18x⁶) + 12x⁵ + 10x³ - 30x² + 12x + (-10 + 6)

Combine like terms.

= 7x⁶ + 12x⁵ + 10x³ - 30x² + 12x - 4

13. Answer :

= -2(2x⁴ - 2x³ - x² + 5) +  3(2x⁴ - 2x² - 3)

Distributive Property.

= -4x⁴ + 4x³ + 2x² -10 +  6x⁴ - 6x² - 9

Group like terms together.

= (-4x⁴ + 6x⁴) + 4x³ + (2x² - 6x²) + (-10 - 9)

Combine like terms.

= 2x⁴ + 4x³ - 4x² - 19

14. Answer :

= 5(x⁴ - x³ + 5) +  2(x⁴ - 5x² - 7)

Distributive Property.

= 5x⁴ - 5x³ + 25 + 2x⁴ - 10x² - 14

Group like terms together.

= (5x⁴ + 2x⁴) - 5x³ - 10x² + (25 - 14)

Combine like terms.

= 7x⁴ - 5x³ - 10x² + 11

15. Answer :

= 3(6x⁴ - 2x³ - 3) + 2(2x⁴ - x² - 8)

Distributive Property.

= 18x⁴ - 6x³ - 9 + 4x⁴ - 2x² - 16

Group like terms together.

= (18x⁴ + 4x⁴) - 6x³ - 2x² + (-9 - 16)

Combine like terms.

= 22x⁴ - 6x³ - 2x² - 25

16. Answer :

= (6x⁷ - 2x⁶ - 3x³ + 2x²) + 2(2x⁴ + 5x⁷ + 3x⁶ + x³ + x²)

Distributive Property.

= 6x⁷ - 2x⁶ - 3x³ + 2x² + 4x⁴ + 10x⁷ + 6x⁶ + 2x³ + 2x²

Group like terms together.

= (6x⁷ + 10x⁷) + (-2x⁶ + 6x⁶) + 4x⁴ + (-3x³ + 2x³) + (2x² + 2x²)

Combine like terms.

= 16x⁷ + 4x⁶ + 4x⁴ - x³ + 4x²

17. Answer :

= (x⁷ - 3x⁶ - 2x³ + x²) + 5(3x⁴ + 15x⁷ + 4x⁶ + 2x³ + 6x²)

Distributive Property.

= x⁷ - 3x⁶ - 2x³ + x² + 15x⁴ + 75x⁷ + 20x⁶ + 10x³ + 30x²

Group like terms together.

= (x⁷ + 75x⁷) + (-3x⁶ + 20x⁶) + (-2x³ + 10x³) + 15x⁴ + (x² + 30x²)

Combine like terms.

= 76x⁷ + 17x⁶ + 8x³ + 15x⁴ + 31x²

18. Answer :

= (3x³ - 2x² - 5x - 6 ) - (2x³ + 5x² - 2x - 11)

Distributive Property.

= 3x³ - 2x² - 5x - 6 -2x³ - 5x² + 2x + 11

Group like terms together.

= (3x³ - 2x³) + (-2x² - 5x²) + (-5x + 2x) + (-6 + 11)

Combine like terms.

= x³ - 7x² - 3x + 5

19. Answer :

= (5x³ + 3x² + 2x - 10) - (x³ + 4x² - 12x - 5)

Distributive Property.

= 5x³ + 3x² + 2x - 10 - x³ - 4x² + 12x + 5

Group like terms together.

= (5x³ - x³) + (3x² - 4x²) + (2x + 12x) + (-10 + 5)

Combine like terms.

= 4x³ - x² + 14x - 5

20. Answer :

= 3(5x³ - 2x + 8) - (2x³ - 5x² + 7x - 3)

Using Distributive Property,

= 3(5x³) + 3(-2x) + 3(+8) - 2x³ + 5x² - 7x + 3

= 15x³ - 6x + 24 - 2x³ + 5x² - 7x + 3

= (15x³ - 2x³) + 5x² + (-6x - 7x) + (24 + 3)

= 13x³ + 5x² + (-13x) + 27

= 13x³ + 5x² - 13x + 27

21. Answer :

= 5(2y³ - 2y² - 7y - 4) - 2(y² + 3y - 1)

Using Distributive Property,

= 5(2y³ - 2y² - 7y - 4) - 2(y² + 3y - 1)

= 5(2y³) + 5(-2y²) + 5(-7y) + 5(-4) - 2(y²) - 2(+3y) - 2(-1)

= 10y³ - 10y² - 35y - 20 - 2y² - 6y + 2

= 10y³ + (-10y² - 2y²) + (-35y - 6y) + (-20 + 2)

= 10y³ + (-12y²) + (-41y) + (-18)

= 10y³ - 12y² - 41y - 18

22. Answer :

= (x² + y²) - (x + y)(x - y)

= x² + y² - [(x + y)(x - y)]

= x² + y² - [x² - xy  + xy - y²]

= x² + y² - [x² - y²]

= x² + y² - x² + y²

= 2y²

23. Answer :

The following algebraic identities can be used to simplify the given expression.

(a - b)³ = a³ - 3a²b + 3ab² - b³

(a + b)³ = a³ + 3a²b + 3ab² + b³

(p - q)³ - (p + q)³ :

= (p³ - 3p²q + 3pq² - q³) - (p³ + 3p²q + 3pq² + q³)

= p³ - 3p²q + 3pq² - q³ - p³ - 3p²q - 3pq² - q³

= -3p²q - q³ - 3p²q - q³

= -6p²q - 2q³

Practice Questions

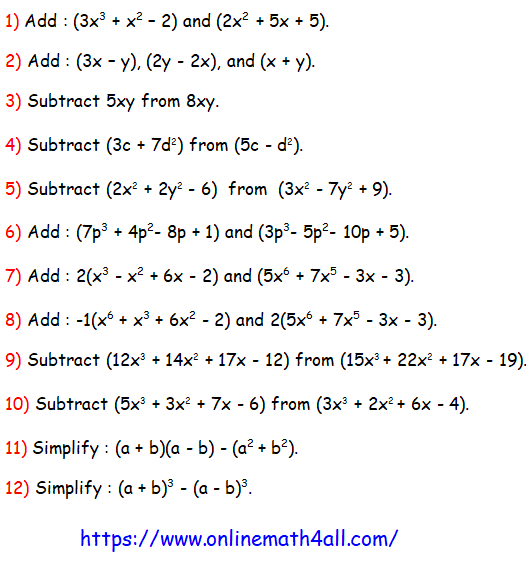
Click here to get detailed answers for the above questions.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.