COMBINING TRANSFORMATIONS

Combining transformations is nothing but doing more than one transformation on a geometric shape. That is, one transformation followed by another transformation. The resulting transformation can frequently be described by an equivalent single transformation.

Example

A triangle has the vertices (3, 4), (5, 4) and (5, 2).  Apply the indicated series of transformations to the triangle. Each transformation is applied to the image of the previous transformation, not the original figure. Label each image with the steps of the transformation applied.

(i)  Reflect across the x-axis.

(ii)  Translate 3 units to the left.

(iii)  Reflect across the y-axis.

(iv)  Translate 4 units up.

(v)  Rotate of 90 ° clockwise  about the origin.

Compare the size and shape of the final image to that of the original figure.

Solution :

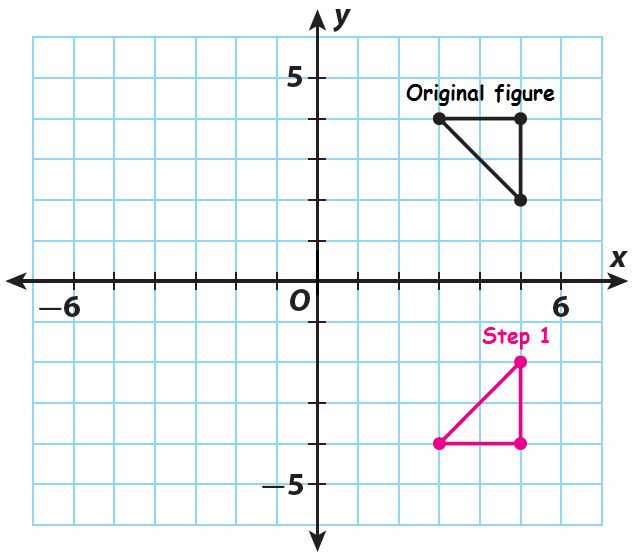
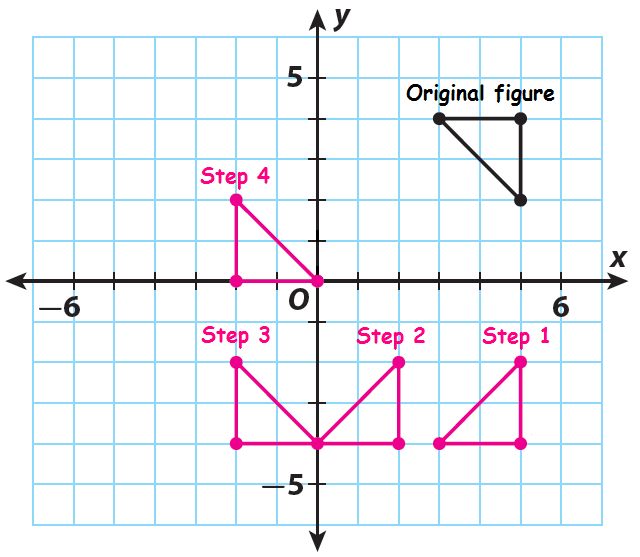
Step 1 :

(i) Reflect across the x-axis.

Since there is a reflection across the x-axis, we have to multiply each y-coordinate by -1. That is,

(x, y) ----> (x, -y)

So, we have

(3, 4) ----> (3, -4)

(5, 4) ----> (5, -4)

(5, 2) ----> (5, -2)

Graph the image.

Step 2 :

(ii) Translate 3 units to the left.

Since there is a translation of 3 units to the left, we have to subtract 3 from each x-coordinate. That is,

(x, y) ----> (x-3, y)

So, we have

(3, -4) ----> (0, -4)

(5, -4) ----> (2, -4)

(5, -2) ----> (2, -2)

Graph the image.

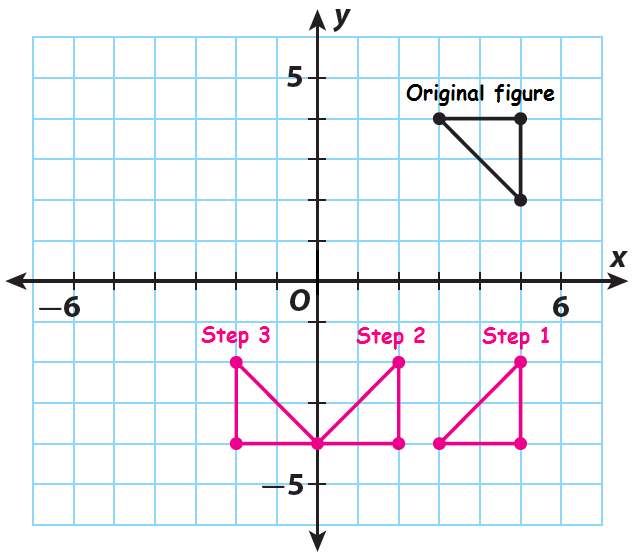
Step 3 :

(iii) Reflect across the y-axis.

Since there is a reflection across the y-axis, we have to multiply each x-coordinate by -1. That is,

(x, y) ----> (-x, y)

So, we have

(0, -4) ----> (0, -4)

(2, -4) ----> (-2, -4)

(2, -2) ----> (-2, -2)

Graph the image.

Step 4 :

(iv) Translate 4 units up.

Since there is a translation of 4 units up, we have to add 4 to each y-coordinate. That is,

(x, y) ----> (x, y + 4)

So, we have

(0, -4) ----> (0, 0)

(-2, -4) ----> (-2, 0)

(-2, -2) ----> (-2, 2)

Graph the image.

Step 5 :

(v) Rotate of 90 ° clockwise  about the origin.

Since there is a rotation of 90° clockwise about the origin, we have multiply each x-coordinate by -1 and interchange x and y coordinates. That is,

(x, y) ----> (y, -x)

So, we have

(0, 0) ----> (0, 0)

(-2, 0) ----> (0, 2)

(-2, 2) ----> (2, 2)

Graph the image.

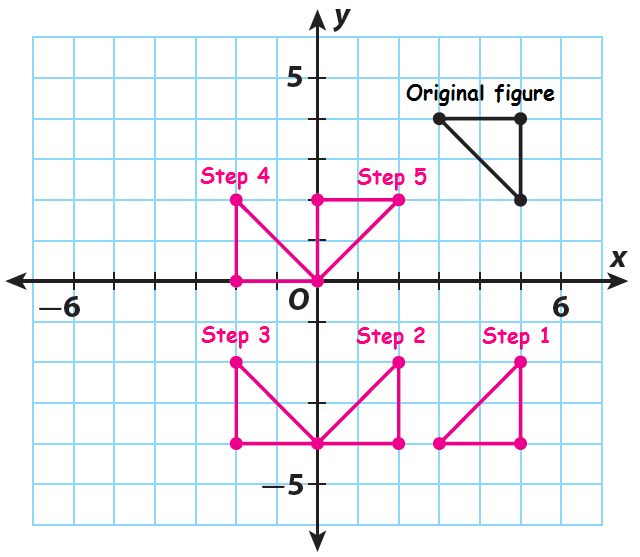
Compare the size and shape of the final image to that of the original figure.

They have the same size and shape, just a different orientation.

Reflect 

1. Which transformations change the orientation of figures?

Reflections and rotations

2.  Which transformations do not change the orientation of figures?

Translations

3.  Two figures have the same size and shape. What does this indicate about the figures?

One figure is the image of the other, and there is a sequence of transformations that will transform one figure into the other.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.