COMPARING COEFFICIENTS OF LINEAR EQUATIONS IN TWO VARIABLES AND SOLVING

To compare the coefficients of linear equations in two variables, the equations must be in the form.

a₁x + b₁y + c₁  =  0

a₂x + b₂y + c₂  =  0

The following three cases are possible for any given system of linear equations.

(i)  a₁/a₂  ≠  b₁/b_2, we get a unique solution

(ii)  a₁/a₂  =  a₁/a₂  = c₁/c₂, there are infinitely many solutions.

(iii)  a₁/a₂  =  a₁/a₂  ≠  c₁/c₂, there is no solution

Problems :

Which of the following pairs of linear equations are consistent/inconsistent? if consistent, obtain the solution graphically. 

(i)  x + y  =  5

   2 x + 2 y  =  10

Solution :

    x + y - 5  =  0

   2 x + 2 y - 10  =  0 

From the given equations, let us find the values of a₁, a₂, b₁, b₂, c₁ and c₂

a₁  =  1, b₁  =  1, c₁  =  -5

a₂  =  2, b₂  =  2, c₂  =  -10

a_1/a₂  =  1/2  -------(1)

b₁/b₂  = 1/2  -------(2)

c₁/c₂  =  -5/(-10)  =  1/2  -------(3)

This exactly matches the condition,

a₁/a₂  =  b₁/b₂  =  c₁/c₂

So, the system of equations will have infinitely many solution.

To draw the graph, let us find x and y intercepts.

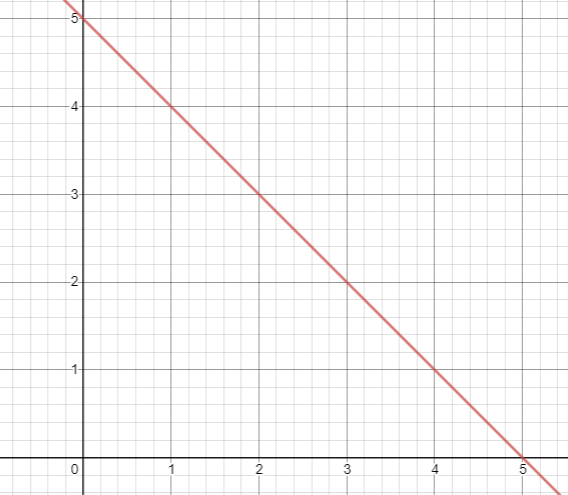
x + y - 5  =  0

Both equations are representing the same line.

(ii) x - y  =  8

   3 x - 3 y  =  16

Solution :

      x -  y – 8  =  0

     3 x - 3 y -16  =  0

From the given equations, let us find the values of a₁, a₂, b₁, b₂, c₁ and c₂

a₁  =  1, b₁  =  -1, c₁  =  -8

a₂  =  3, b₂  =  -3, c₂  =  -16

a_1/a₂  =  1/3  -------(1)

b₁/b₂  = (-1)/(-3)  =  1/3  -------(2)

c₁/c₂  =  -8/(-16)  =  1/2  -------(3)

This exactly matches the condition

 a_1/a₂  =  b₁/b₂  ≠  c₁/c₂

So, there is no solution. 

(iii)  2 x + y - 6  =  0

       4 x - 2 y - 4  =  0

Solution :

From the given equations, let us find the values of a₁, a₂, b₁, b₂, c₁ and c₂

a₁  =  2, b₁  =  1, c₁  =  -6

a₂  =  4, b₂  =  -2, c₂  =  -4

a_1/a₂  =  2/4  =  1/2  -------(1)

b₁/b₂  = 1/(-2)  =  -1/2  -------(2)

c₁/c₂  =  -6/(-4)  =  3/2  -------(3)

This exactly matches the condition a₁/a₂  ≠  b₁/b₂

So, it has unique solution.

Graphing 1^st equation,

2 x + y - 6  =  0

y  =  -2x + 6

Graphing 2^nd equation,

4 x - 2 y - 4 = 0

2y  =  4x - 4

y  =  2x - 2

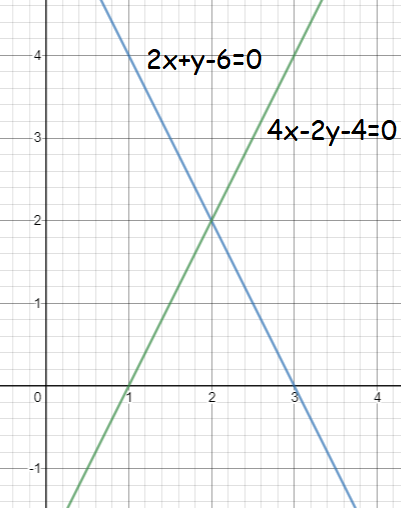
The above lines are intersecting at the point (2, 2). So, the solution is x  =  2 and y  =  2.

(iv)  2 x - 2 y - 2  = 0

       4 x - 4 y  - 5 = 0

Solution :

From the given equations, let us find the values of a₁, a₂, b₁, b₂, c₁ and c₂

a₁  =  2, b₁  =  -2, c₁  =  -2

a₂  =  4, b₂  =  -4, c₂  =  -5

a_1/a₂  =  2/4  =  1/2  -------(1)

b₁/b₂  = -2/(-4)  =  1/2  -------(2)

c₁/c₂  =  -2/(-5)  =  2/5  -------(3)

This exactly matches the condition a₁/a₂  =  b₁/b₂ ≠ c₁/c₂

This exactly matches the condition

 a_1/a₂  =  b₁/b₂  ≠  c₁/c₂

So, there is no solution. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.