COMPLETING THE SQUARE PRACTICE WORKSHEET

Solve the following quadratic equations by completing the square

(i) x²+6x–7  =  0

(ii)  x²+3x+1  =  0

(iii)  2x²+5x-3  =  0

(iv)  4x²+4bx–(a²-b²)  =  0

(v)  (5x+7)/(x–1)  =  3x+2

Solution

Question 1 :

x²+6x–7  =  0

Solution :

x²+6x–7  =  0

Step 1 :

Notice that the coefficient of x² is 1, so don't have to divide the entire equation by any numerical value.

Step 2 :

Write the coefficient of x as multiple of 2.

x²+2⋅x⋅3 –7  =  0

Step 3 :

Here the first and second term is in the form a² + 2ab, in order to complete this formula, we need b².

Here b  =  3

x²+2⋅x⋅3+3²-3² –7  =  0

(x+3)²–9–7  =  0

(x+3)²–16  =  0

(x+3)²  =  16

(x+3)  =  √16

x+3  =  ±4

Question 2 :

x²+3x+1  =  0

Solution :

Step 1 :

Notice that the coefficient of x² is 1, so don't have to divide the entire equation by any numerical value.

Step 2 :

Write the coefficient of x as multiple of 2.

x²+2⋅x⋅(3/2)+1  =  0

Step 3 :

Here the first and second term is in the form a² + 2ab, in order to complete this formula, we need b².

Here b  =  3

 x²2⋅x⋅(3/2)+(3/2)²-(3/2)²+1  =  0

[x + (3/2)]² – (9/4) + 1 = 0

[x+(3/2)]²  =  (9/4) – 1

[x+(3/2)]²  =  5/4

x+(3/2)  =  √(5/4)

[x+(3/2)]  =  ±√5/2

So, the solution is {(√5-3)/2, (-√5-3)/2}.

Question 3 :

2x²+5x-3  =  0

Solution :

Step 1 :

Notice that the coefficient of x² is 2, so divide the entire equation by 2.

x²+(5x/2)-(3/2)  =  0

Step 2 :

Write the coefficient of x as multiple of 2.

x²+2⋅x⋅(3/2)+1  =  0

Step 3 :

Here the first and second term is in the form a² + 2ab, in order to complete this formula, we need b².

Here b  =  3

2x² + 5x - 3 = 0

Now we are going to divide the whole equation by 2

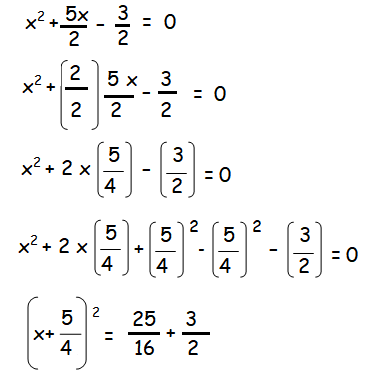
(x+5/4)²  =  49/16

(x+5/4)  =  √49/16

(x+5/4)  =  ±7/4

So, the solution is {-3, 1/2}.

Question 4 :

4x²+4bx–(a² - b²)  =  0

Solution :

4x²+4bx–(a² - b²)  =  0

Now we are going to divide the whole equation by 4

x²+bx–[(a² - b²)/4]  =  0

x²+2(x)(b/2)+(b/2)²–(b/2)² – [(a² - b²)/4]  =  0

[x + (b/2)]² – (b²/4) – [(a²-b²)/4]  =  0

[x + (b/2)]²  =  (b²/4)+[(a²-b²)/4]

[x + (b/2)]²  =  [(b²+a²-b²)/4]

[x + (b/2)]²  =  a²/4

[x + (b/2)]  =  √(a²/4)

[x + (b/2)]  =  ±(a/2)

So, the solution is {(a-b)/2, (-a-b)/2}.

Question 5 :

(5x+7)/(x–1)  =  3x+2

Solution :

(5x+7)  =  (3x+2)(x–1)

5x+7  =  3x²–x–2

3x²–x–5x–7-2  =  0

3x²–6x–9  =  0

x²–2x–3  =  0

(x–3)(x+1)  =  0

So, the solution is {-1, 3}.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.