CONSTRUCTION OF INCIRCLE OF A TRIANGLE

Key Concept - Incircle

The circle drawn with I (incenter) as center and touching all the three sides of the triangle is called as incircle. 

Before we learn how to  construct incircle of a triangle, first we have to learn how to construct angle bisector. 

So, let us learn how to construct angle bisector. 

Constructing Angle Bisector - Steps

To construct an angle bisector, you must need the following instruments.

1. Ruler

2. Compass

3. Protractor

The steps for the construction of an angle bisector are. 

Step 1 :

Construct an angle of given measure at O using protractor.

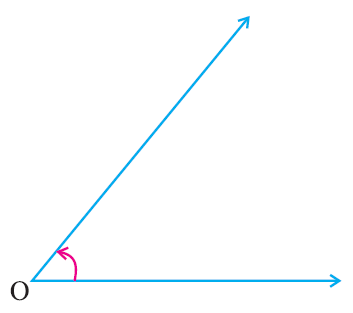
Step 2 :

With ‘O’ as center draw an arc of any radius to cut the rays of the angle at A and B.

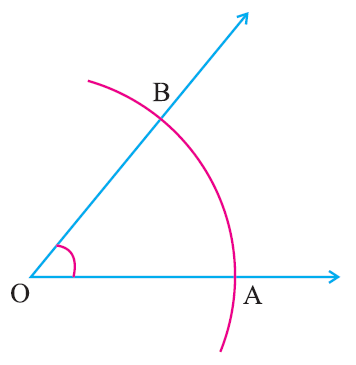
Step 3 :

With ‘A’ as center draw an arc of radius more than half of AB, in the interior of the given angle.

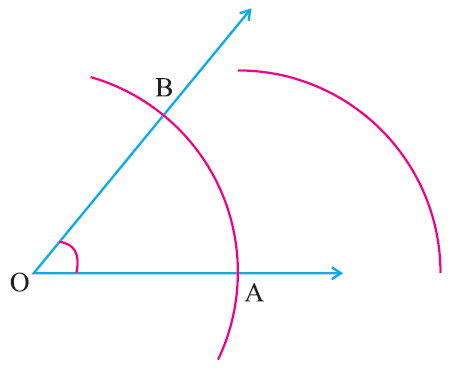
Step 4 :

With ‘B’ as center draw an arc of same radius to cut the previous arc at ‘C’.

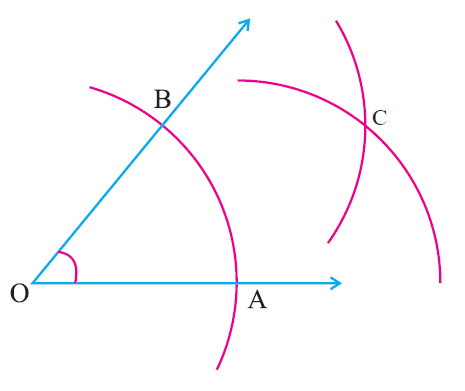
Step 5 :

Join OC.

OC is the angle bisector of the given angle.

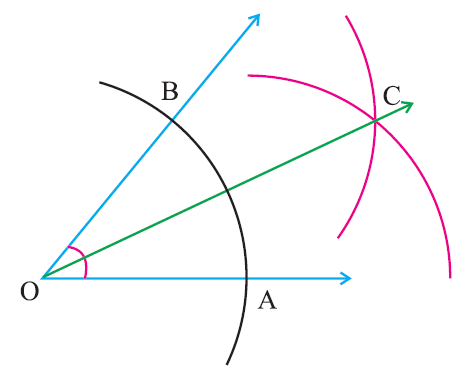
This construction clearly shows how to draw the angle bisector of a given angle with compass and straightedge or ruler. The angle bisector divides the given angle into two equal parts. 

For example, if we draw angle bisector for the angle 60°, the angle bisector will divide 60° in to two equal parts and each part will measure 30°.

Now, let us see how to construct incircle of a triangle. 

Constructing Incircle of a Triangle - Steps

To construct a incenter, we must need the following instruments.

1. Ruler

2. Compass

Let us see, how to construct incenter through the following example.  

Construct the incircle of the triangle ABC with AB = 7 cm, ∠B = 50° and BC = 6 cm. And also measure its radius.

Step 1 :

Draw triangle ABC with the given measurements.

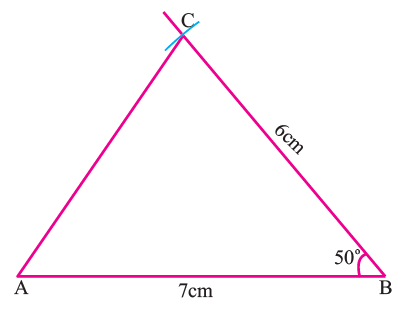
Step 2 :

Construct the angle bisectors of any two angles (A and B) and let them meet at I. 

In the above figure,  I is the incenter of triangle ABC.

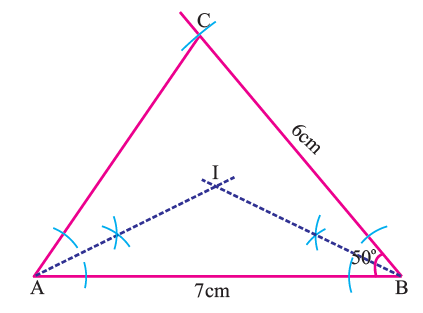
Step 3 :

With I as an external point drop a perpendicular to any one of the sides to meet at D.

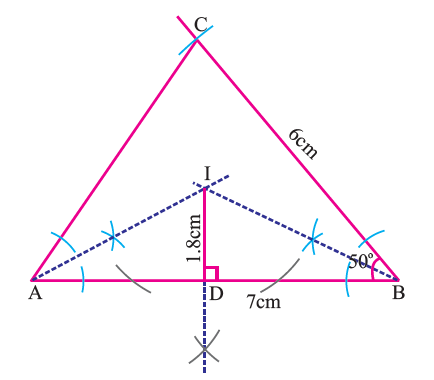
Step 4 :

With I as center and ID as radius draw the circle. This circle touches all the sides of the triangle.

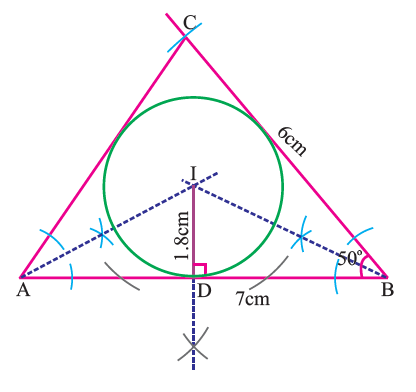
In the above figure, circumradius  =  1.8 cm.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.