CONVERSE OF THE PYTHAGOREAN THEOREM PROOF

Converse of the Pythagorean Theorem

If the square of the length of the longest side of a triangle is equal to the sum of the squares of the lengths of the other two sides, then the triangle is a right triangle.

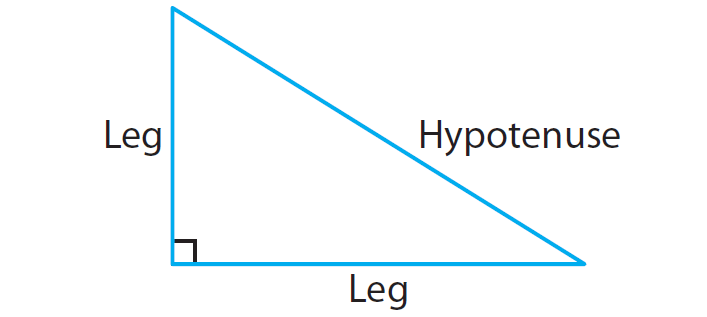
In the diagram above, if

c²  =  a² + b²

then,  ΔABC is a right triangle.

Converse of the Pythagorean Theorem Proof

Given :

A triangle ABC with the longest side AC and 

AB² + BC²  =  AC²

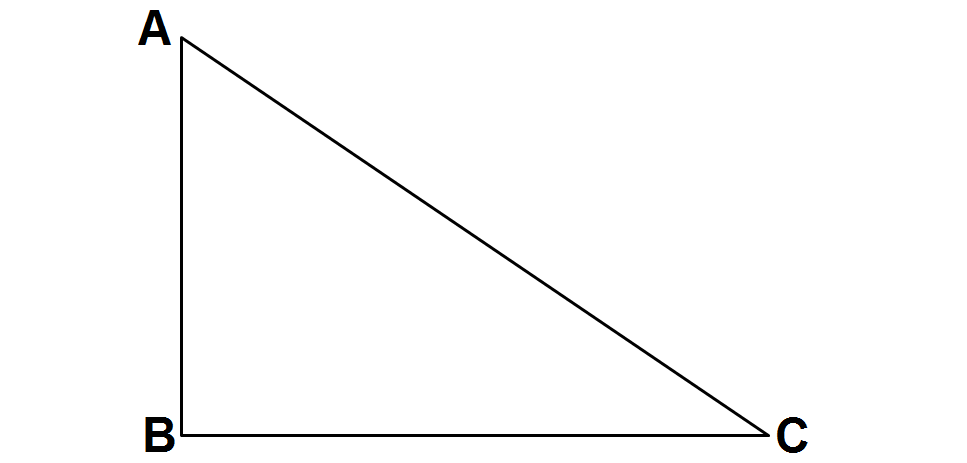
To Prove :

ΔABC is right a right triangle.

Construction :

Construct a right-angled triangle PQR, right-angled at Q such that PQ  =  AB and QR  =  BC.

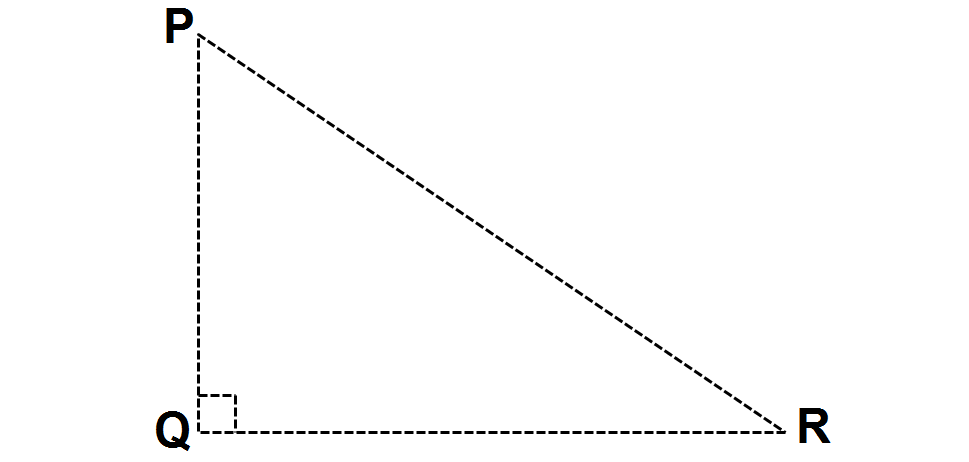
Proof :

Step 1 :

In ΔPQR, ∠Q = 90°.

Using Pythagorean theorem in ΔPQR, we have

PQ² + QR²  =  PR² -----(1)

Step 2 :

In ΔABC (given), we have

AB² + BC²  =  AC² -----(2)

Step 3 :

By construction,  PQ  =  AB and QR  =  BC.

So, from (1) and (2), we have

PR²  =  AC²

Get rid of the square from both sides.

PR  =  AC

Step 4 :

Therefore, by SSS congruence criterion, we get

ΔABC  ≅  ΔPQR

which gives

∠B  =  ∠Q

Step 5 :

But, we have ∠Q = 90° by construction.

Therefore ∠B = 90°.

Hence, ΔABC is a right triangle, right angled at B.

Thus, the theorem is proved.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.