CONVERTING BETWEEN LOGARITHMIC AND EXPONENTIAL FORM

Logarithmic to Exponential Form

Consider the following equation in logarithmic form.

log_am = x

The picture below illustrates how to convert the above equation from logarithmic to exponential form.

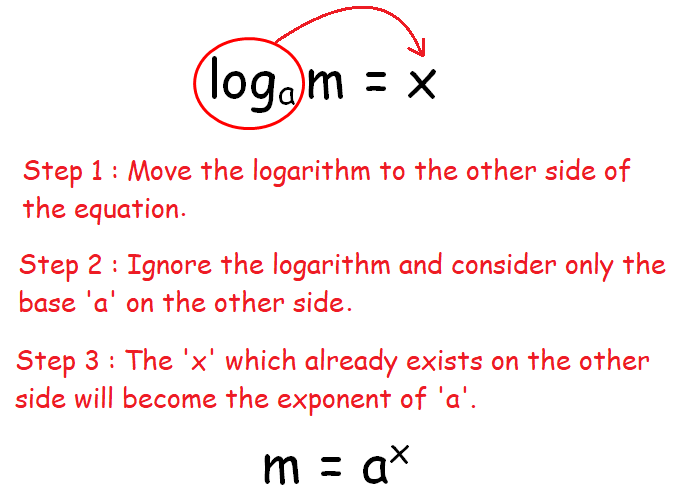
Exponential to Logarithmic Form

Consider the following equation in exponential form.

b^y = a

The picture below illustrates how to convert the above equation from exponential to logarithmic form.

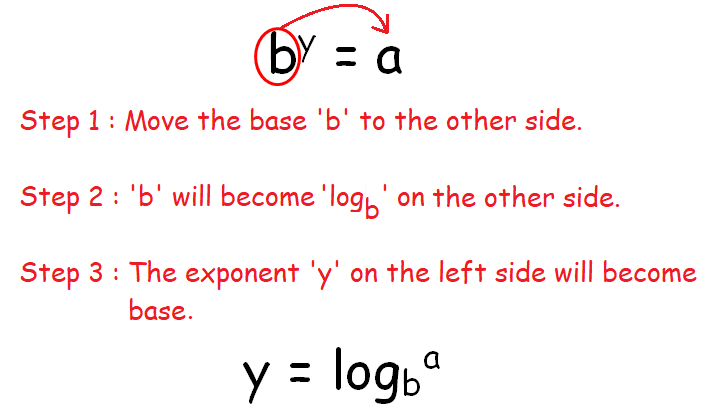
Still don't understand what is explained above, please watch the video below for step by step live explanation.

Solved Problems

Problem 1  :

Find the value of d if log_x5 = 1/2.

Solution :

log_x5 = 1/2

The above equation is in logarithmic form. Convert it to exponential form to solve for d.

5 = x^1/2

Raise the exponent to 2 on both sides.

(x^1/2)² = 5²

Using power of a power rule,

x¹ = 25

x = 25

Problem 2  :

Find the value of d if log_a√2 = 1/6.

Solution :

log_a√2 = 1/6

The above equation is in logarithmic form. Convert it to exponential form to solve for d.

√2 = a^1/2

Raise the exponent to 2 on both sides.

(a^1/2)² = (√5)²

Using power of a power rule,

a¹ = 5

a = 5

Problem 3  :

Find the value of x if 3^x = 1/9.

Solution :

3^x = 1/9

The above equation is in exponential form. Convert it to logarithmic form to solve for x.

x = log₃(1/9)

x = log₃1 - log₃9

x = 0 - log₃(3²)

x = -2log₃3

x = -2(1)

x = -2

Problem 4  :

Find the value of x if 2^{3x - 1} = 1/16.

Solution :

2^{3x - 1} = 1/16

The above equation is in exponential form. Convert it to logarithmic form to solve for x.

3x - 1 = log₂(1/16)

3x - 1 = log₂1 - log₂16

3x - 1 = 0 - log₂(2⁴)

3x - 1 = -4log₂2

3x - 1 = -4(1)

3x - 1 = -4

Add 1 to both sides.

3x = -3

Divide both sides by 3.

x = -1

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.