COORDINATE OF THE FOOT OF THE PERPENDICULAR FROM THE POINT TO THE LINE

Question 1 :

Find the coordinates of the foot of the perpendicular from the origin on the straight line 3x + 2 y = 13

Solution :

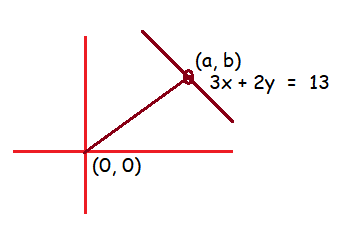
Equation of the line joining the points (0, 0) and (a, b)

Slope of the line joining the points (0, 0) and (a, b)

  m  =  (b - 0)/(a - 0)

  m  =  b/a

Slope of the line 3x + 2y  =  13

m  =  -3/2

Product of slopes  =  -1

(b/a) ⋅ (-3/2)  =  -1

3b/2a  =  1

3b  =  2a

b  =  2a/3

The line 3x + 2y  =  13 passing through the point (a, b)

3a + 2(2a/3)  =  13

3a + (4a/3)  =  13

(9a + 4a)/3  =  13

13a/3  =  13

a  =  3

b  =  2(3)/3

b  =  2

Hence the foot of perpendicular is (3, 2).

Question 2 :

If x + 2y = 7 and 2 x + y = 8 are the equations of the lines of two diameters of a circle, find the radius of the circle if the point (0,-2) lies on the circle.

Solution :

To find the center of the circle we need to find the point of intersection of two given lines 

x + 2y = 7 ---- (1)

2 x + y = 8 ---- (2)

(1) - (2)

(1) x 2 => 2x + 4y = 14

              2x  + y = 8

               (-)  (-)  (-)

        ----------------- 

              3y = 6

               y = 2

Substitute y = 2 in the first equation

x + 2(2) = 7

x + 4 = 7 

x = 3

the point of intersection is (3,2)

Distance between the points (3,2) and (0,-2)

  =  √(x₂ - x₁)²  + (y₂ - y₁)²

  =  √(0 - 3)²  + (-2 - 2)²

  =  √(- 3)²  + (-4)²

  =  √9  + 16

  =  √25

  = 5 units

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.