CUBOIDS

It is a three dimensional solid having six rectangular faces.

Example: Bricks, Books etc.,

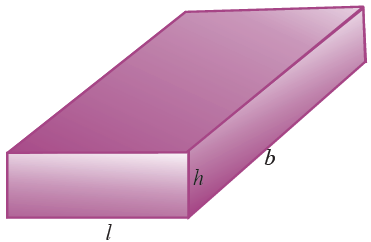
Surface area of a cuboid

Let l, b and h be the length, breadth and height of a cuboid respectively. To find the

total surface area, we split the faces into three pairs.

(i) The total area of the front and back faces is

lh + lh  =  2lh square units.

(ii) The total area of the side faces is

bh + bh  =  2bh square units.

(iii) The total area of the top and bottom faces is

lb + lb  =  2lb square units.

Volume of cuboid

If the length, breadth and height of a cuboid are l, b and h respectively, then the volume V of the cuboid is given by the formula

V  =  l ⋅ b ⋅ h cubic units

Example problems of surface area and volume of cuboid

Example 1 :

Find the total surface area of a cuboid whose length, breadth and height are 20 cm, 12 cm and 9 cm  respectively.

Solution :

Given that l = 20 cm, b = 12 cm, h = 9 cm

T.S.A = 2 (lb + bh + lh)

  =  2[(20 ⋅ 12) + (12 ⋅ 9) + (20 ⋅ 9)]

  =  2(240 + 108 + 180)

  =  2 (528)

  =  1056 cm²

Example 2 :

Find the L.S.A of a cuboid whose dimensions are given by 3m ⋅ 5m ⋅ 4m

Solution :

Given that l  =  3 m, b  =  5 m, h  =  4 m

L.S.A  =  2h (l + b)

  =  2(4) (3 + 5)

  =  8 (8)

  =  64 sq. m

Hence the required lateral surface area is 64 sq. m.

Example 3 :

Find the volume of a cuboid whose dimensions are given by 11 m, 10 m and 7 m.

Solution :

Given that l = 11 m, b = 10 m, h = 7 m

Volume of cuboid  =  lbh

  =  11 ⋅ 10 ⋅ 7

  =  770 cu.m.

Example 4 :

Two cubes each of volume 216 cm³ are joined to form a cuboid as shown in the figure.

Find the T.S.A of the resulting cuboid.

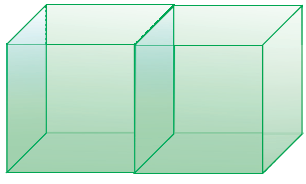
Solution :

Let the side of each cube be a. Then a³  =  216

 a = ∛216 = 6 cm

Now the two cubes of side 6 cm are joined to form a cuboid.

So,

l = 6 + 6 = 12 cm, b = 6 cm, h = 6 cm

Total surface area  =  2 (lb + bh + lh)

  =  2 [(12 ⋅ 6) + (6 ⋅ 6) + (12 ⋅ 6)]

  =  2 [72 + 36 + 72]

  =  2 ⋅ 180 

=  360 cm²

Hence the required total surface area is 360 cm²

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.