DIFFERENCE BETWEEN SIMPLE AND COMPOUND INTEREST

Simple interest is only based on the principal amount of a loan, while compound interest is based on the principal amount and the accumulated interest.

We can explain the difference between simple interest and compound interest in simple words as given below.

Example :

$1000 is invested at 10% simple interest and 10% compound interest where the interest is compounded annually.

The picture given below explains the difference between simple interest and compound interest for the above investment.

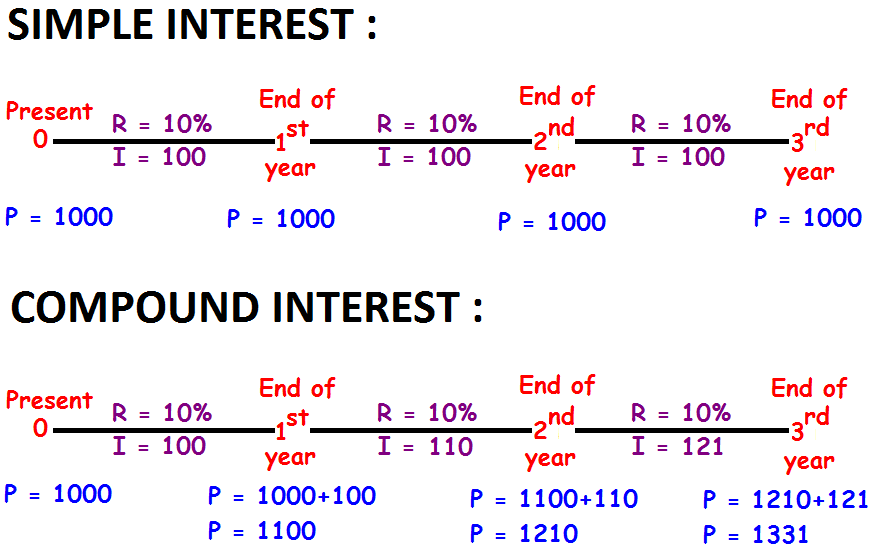
Important Note :

When we look at the above picture, it is clear that interest earned in simple interest and compound interest is same ($100) for the 1st year when interest is compounded annually in compound interest.

Formula for Difference between Simple and Compound Interest (2 years and 3 years)

The above two formulas are applicable only in the following conditions.

1. The principal in simple interest and compound interest must be same.

2. Rate of interest must be same in simple interest and compound interest.

3. In compound interest, interest has to be compounded annually.

Solved Questions

Question 1 :

The difference between the compound interest and simple interest on a certain investment at 10% per year for 2 years is $631. Find the value of the investment.

Answer :

The difference between compound interest and simple interest for 2 years is 631.

P(R/100)² = 631

Substitute R = 10.

P(10/100)² = 631

P(1/10)² = 631

P(1/100) = 631

Multiply each side by 100.

P = 631 x 100

P = 63100

So, the value of the investment is $63100.

Question 2 :

The difference between the compound interest and simple interest on a certain principal is at 10% per year for 3 years is $31. Find the principal.

Answer :

The difference between compound interest and simple interest for three years is 31.

P(R/100)²(R/100 + 3) = 31

Substitute R = 10.

P(10/100)²(10/100 + 3) = 31

P(1/10)²(1/10 + 3) = 31

P(1/10)²(31/10) = 31

Multiply each side by 1000/31.

P = 31 x (1000/31)

P = 1000

So, the principal is $1000.

Question 3 :

The compound interest and simple interest on a certain sum for 2 years is $ 1230 and $ 1200 respectively. The rate of interest is same for both compound interest and simple interest and it is compounded annually. What is the principal ?

Answer :

To find the principal, we need rate of interest. So, let us find the rate of interest first.

Step 1 :

Simple interest for two years is $1200. So interest per year in simple interest is $600.

So, C.I for 1st year is $600 and for 2nd year is $630.

(Since it is compounded annually, S.I and C.I for 1st year would be same)

Step 2 :

When we compare the C.I for 1st year and 2nd year, it is clear that the interest earned in 2nd year is 30 more than the first year.

Because, in C.I, interest $600 earned in 1st year earned this $30 in 2nd year.

It can be considered as simple interest for one year.

That is principle = 600, interest = 30.

I = PRT/100

30 = (600 x R x 1)/100

30 = 6R

Divide each side by 6.

30/6 = R

5 = R

So, R = 5%.

Step 3 :

The difference between compound interest and simple interest for two years is

= 1230 - 1200

= 30

Then we have,

P(R/100)² = 30

Substitute R = 5.

P(5/100)² = 30

P(1/20)² = 30

P(1/400) = 30

Multiply each side by 400.

P = 30 x 400

P = 12000

So, the principal is $12000.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.