DIRECTION COSINES AND RATIOS

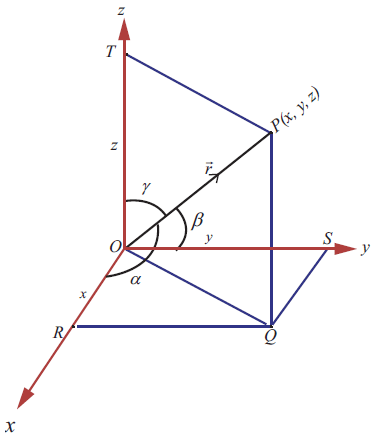
Let P be a point in the space with coordinates (x, y, z) and of distance r from the origin. 

Let R, S and T be the foots of the perpendiculars drawn from P to the x, y and z axes respectively. Then

∠PRO  =  90°

∠PSO  =  90°

∠PTO  =  90° 

OR = x, OS = y, OT = z and OP = r.

OR = x, OS = y, OT = z and OP = r.

(It may be difficult to visualize that ∠PRO  =  ∠PSO  =  ∠PTO  =  90° in the figure; as they are foot of the perpendiculars to the axes from P; in a three dimensional model we can easily visualize the fact.)

Let α, β, γ be the angles made by the vector OP vector with the positive x, y and z axes respectively. That is,

∠PRO   =  α, ∠POS   =  β, ∠POT   =  γ

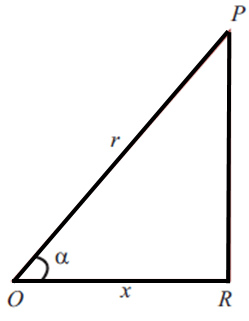In triangle OPR, ∠PRO = 90°, ∠POR =  α, OR = x, and OP = r. Hence cos α = OR/OP  =  x/r

In a similar way we can find that cos β  =  y/r and cos γ  =  z/r .

Direction Angles :

Here the angles α, β, γ are called direction angles of the vector OP vector = r vector.

Direction Cosines :

Cos α, cos β, cos γ are called direction cosines of the vector OP = xi vector + yj vector + zk vector.

Thus (x/r, y/r, z/r), where r  =  √(x² + y² + z²) are the direction cosines of the vector r vector  =  xi vector + yj vector + zk vector.

How to verify the direction ratios are direction cosines of a vector ?

Question 1 :

Verify whether the following ratios are direction cosines of some vector or not.

(i) 1/5 , 3/5 , 4/5

Solution :

In order to verify the given ratios are direction cosines, it has to satisfy the condition given below.

The sum of the squares of the direction cosines of r vector is 1.

That is , 

x² + y² + z²  =  1

r vector = xi vector + yj vector + zk vector 

r vector = (1/5)i vector + (3/5)j vector + (4/5)k vector 

x² + y² + z²  =  (1/5)² + (3/5)² + (4/5)²

   =  (1/25) + (9/25) + (16/25)

  =  (1 + 9 + 16)/25

  =  26/25 

x² + y² + z²  ≠ 1

Hence the direction ratios are not direction cosines of some vector.

(ii) 1/√2, 1/2 , 1/2

Solution :

r vector = (1/√2)i vector + (1/2)j vector + (1/2)k vector 

x² + y² + z²  =  (1/√2)² + (1/2)² + (1/2)²

   =  (1/2) + (1/4) + (1/4)

  =  (2 + 1 + 1)/4

  =  1

Hence the direction ratios are the direction cosines of some vector.

(iii) 4/3, 0, 3/4

Solution :

r vector = (4/3)i vector + (0)j vector + (3/4)k vector 

x² + y² + z²  =  (4/3)² + (0)² + (3/4)²

   =  (16/9) + 0 + (9/16)

  =  (256 + 0 + 81)/144

x² + y² + z²  ≠ 1

Hence the direction ratios are not direction cosines of some vector.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.