EQUATION OF A PARABOLA WITH VERTEX AND FOCUS
The following steps would be useful to find the equation of a parabola when vertex and focus are given.
Step 1 :
Draw a rough diagram of the parabola with given vertex and focus.
Step 2 :
From step 1, you can know the side to which the parabola opens (right or left or up or down) and the axis (x-axis and y-axis) about which the parabola is symmetric.
Step 3 :
Using the given vertex, focus and result received in step 2, write the equation of the parabola.
Example 1 :
Find the equation of the parabola, if the vertex is (4, 1) and the focus is (4, -3).
Solution :
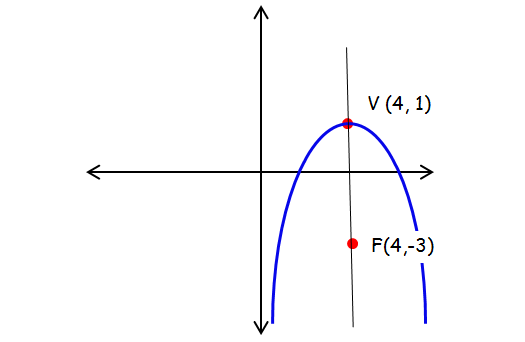
From the given information the parabola is symmetric about y -axis and it opens down.
Distance between vertex and focus = a.
a = VF
= √[(4 - 4)2 + (1 + 3)2]
= √[0 + 42]
= √16
a = 4
Equation of the parabola :
(x - h)2 = -4a(y - k)
Here, vertex (h, k) = (4, 1) and a = 4.
(x - 4)2 = -4(4)(y - 1)
(x - 4)2 = -16(y - 1)
Example 2 :
Find the equation of the parabola if the vertex is (0, 0) and the focus is (0, 4).
Solution :
From the given information the parabola is symmetric about y -axis and it opens up.
Distance between vertex and focus = a.
a = VF
= √[(0 - 0)2 + (0 - 4)2]
= √(0 + 42)
= √16
a = 4
Equation of the parabola :
(x - h)2 = 4a(y - k)
Here, vertex (h, k) = (0, 0) and a = 4.
(x - 0)2 = 4(4)(y - 0)
x2 = 16y
Example 3 :
Find the equation of the parabola if the vertex is (1, 4) and the focus is (-2, 4).
Solution :
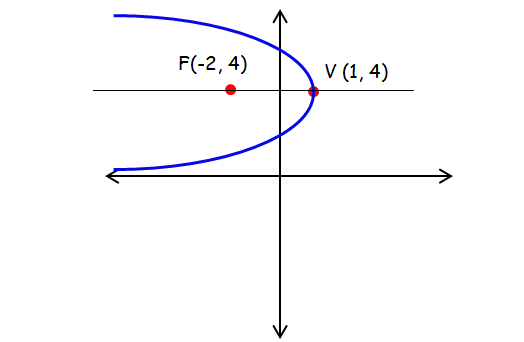
From the given information the parabola is symmetric about x -axis and it opens to the left.
Distance between vertex and focus = a
a = VF
= √[(1 + 2)2 + (4 - 4)2]
= √(32 + 0)
= √9
a = 3
Equation of the parabola :
(y - k)2 = -4a(x - h)
Here, vertex (h, k) = (1, 4) and a = 3.
(y - 4)2 = -4(3)(x - 1)
(y - 4)2 = -12(x - 1)
Example 4 :
Find the equation of the parabola if the vertex is (0, 0) and the focus is (5, 0).
Solution :
From the given information the parabola is symmetric about x -axis and it opens to the right.
Distance between vertex and focus = a
a = VF
= √[(0 - 5)2 + (0 - 0)2]
= √(52 + 0)
= √25
a = 5
Equation of the parabola :
(y - k)2 = 4a(x - h)
Here, vertex (h, k) = (0, 0) and a = 5.
(y - 0)2 = 4(5)(x - 0)
y2 = 20x
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Digital SAT Math Problems and Solutions (Part - 108)
Feb 10, 25 07:03 AM
Digital SAT Math Problems and Solutions (Part - 108) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Feb 10, 25 07:01 AM
SAT Math Resources (Videos, Concepts, Worksheets and More) -
Digital SAT Math Problems and Solutions (Part - 109)
Feb 10, 25 06:59 AM
Digital SAT Math Problems and Solutions (Part - 109)