EQUATION OF PARABOLA WORKSHEET

Use the information provided to write the standard form equation of each parabola.

Problem 1 : 

Vertex at origin, Focus (0, 1)

Problem 2 : 

Vertex at origin, Focus (2, 0)

Problem 3 : 

Vertex at (1, 2), Focus (1, -1)

Problem 4 : 

Vertex at (2, -1), Focus (-1, -1)

Problem 5 : 

Opens up or down, Vertex at origin, Passes through (5, 75)

Problem 6 : 

Opens left or right, Vertex (0,0), Passes through (-16, 2)

Problem 7 : 

Opens up or down, Vertex (3, 1), Passes through (1, 9)

Problem 8 : 

Opens left or right, Vertex (-1, -2), Passes through (11, 0)

Problem 9 : 

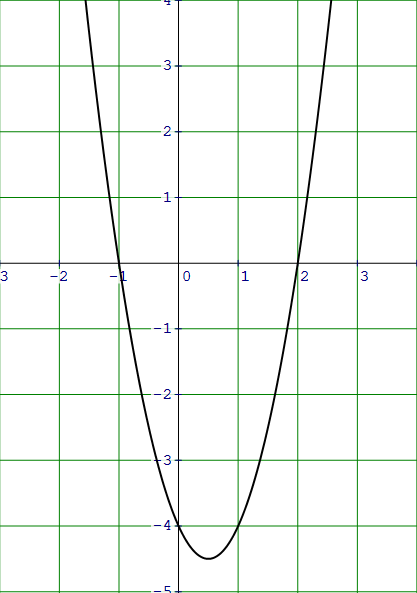
Write the intercept form equation of the parabola shown below.

Use the information provided to write the standard form equation of each parabola.

Problem 10 : 

Vertex (2, 3), Directrix : y = 6

Problem 11 : 

Vertex (2, -2), Latus rectum : x = 1

Problem 12 : 

Opens up, Vertex (-3, 4), Length of Latus rectum : 8 units

Detailed Answer Key

Use the information provided to write the standard form equation of each parabola.

Problem 1 : 

Vertex at origin, Focus (0, 1)

Solution : 

Plot the vertex (0, 0) and focus (0, 1) on the xy-plane. 

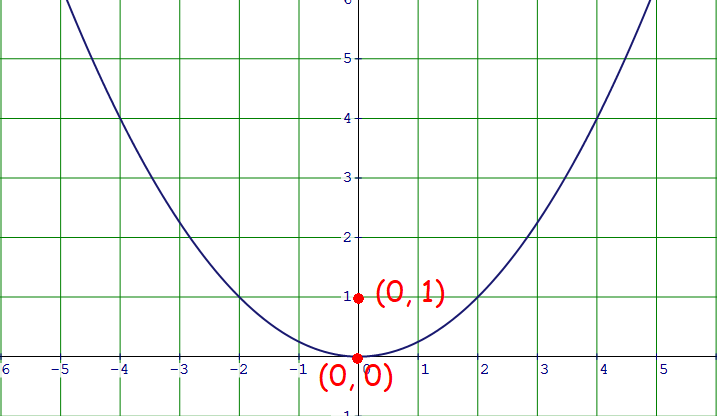
The parabola is open up with vertex at origin. 

Standard form equation of a parabola that opens up with vertex at origin :

x²  =  4ay

Distance between the vertex and focus is 1 unit. 

That is, a = 1. 

x²  =  4(1)y

x²  =  4y

Problem 2 : 

Vertex at origin, Focus (2, 0)

Solution : 

Plot the vertex (0, 0) and focus (2, 0) on the xy-plane.

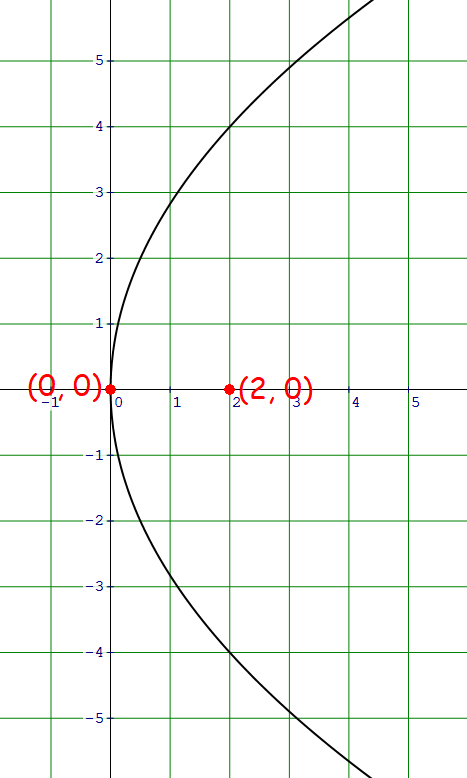
The parabola is open to the right with vertex at origin. 

Standard form equation of a parabola that opens right with vertex at origin : 

y²  =  4ax

Distance between the vertex and focus is 2 units. 

That is, a = 2. 

y²  =  4(2)x

y²  =  8x

Problem 3 : 

Vertex at (1, 2), Focus (1, -1)

Solution : 

Plot the vertex (1, 2) and focus (1, -1) on the xy-plane.

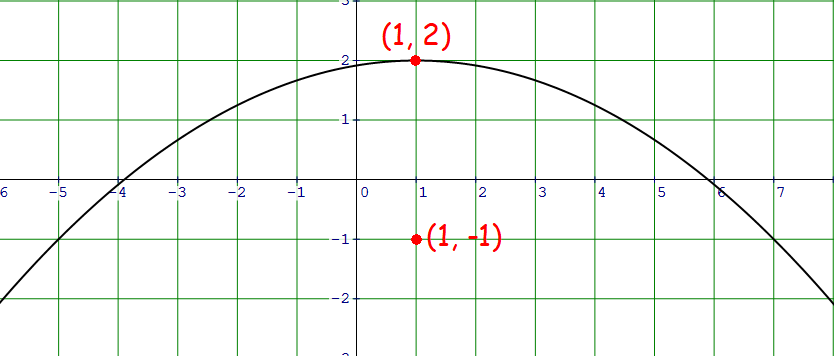
The parabola is open down with vertex at (1, 2). 

Standard form equation of a parabola that opens down with vertex at (h, k) : 

(x - k)²  =  -4a(y - h)

Vertex (h, k) = (1, 2).

(x - 1)²  =  -4a(y - 2)

Distance between the vertex and focus is 3 units. 

That is, a = 3. 

(x - 1)²  =  -4(3)(y - 2)

(x - 1)²  =  -12(y - 2)

Problem 4 : 

Vertex at (2, -1), Focus (-1, -1)

Solution : 

Plot the vertex (2, -1) and focus (-1, -1) on the xy-plane.

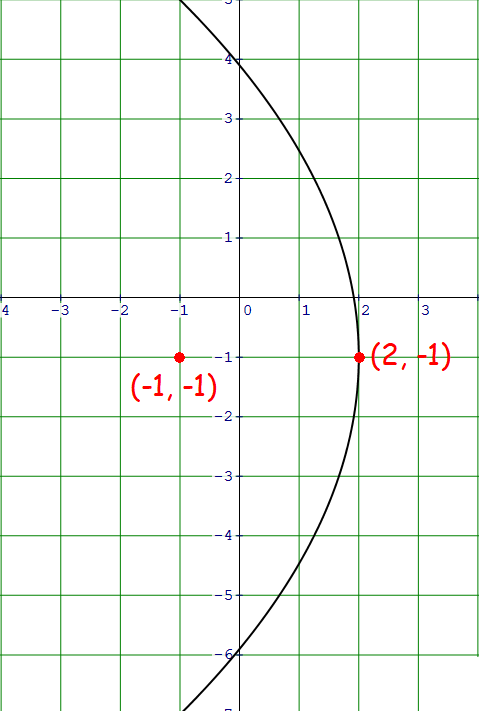
The parabola is open to the left with vertex at (2, -1).

Standard equation of a parabola that opens left with vertex at (h, k) : 

(y - k)²  =  -4a(x - h)

Vertex (h, k) = (2, -1).

(y + 1)²  =  -4a(x - 2)

Distance between the vertex and focus is 3 units. 

That is, a = 3. 

(y + 1)²  =  -4(3)(x - 2)

(y + 1)²  =  -12(x - 2)

Use the information provided to write the vertex form equation of each parabola.

Problem 5 : 

Opens up or down, Vertex at origin, Passes through (5, 75)

Solution : 

Vertex form equation of a parabola that opens up or down with vertex at origin : 

y  =  ax²

It passes through (5, 75). Substitute (x, y) = (5, 75). 

75  =  a(5)²

75  =  25a

Divide each side by 25.

3  =  a

Vertex form equation of the parabola : 

y  =  3x²

Problem 6 : 

Opens left or right, Vertex (0,0), Passes through (-16, 2)

Solution : 

Vertex form equation of a parabola that opens left or right with vertex at origin : 

x  =  ay²

It passes through (-16, 2). Substitute (x, y) = (-16, 2). 

-16  =  a(2)²

-16  =  a(4)

Divide each side by 4.

-4  =  a

Vertex form equation of the parabola : 

x  =  -4y²

Problem 7 : 

Opens up or down, Vertex (3, 1), Passes through (1, 9)

Solution : 

Vertex form equation of a parabola that opens up or down with vertex at (h, k) :

y  =  a(x - h)² + k

Vertex (h, k) = (3, 1).

y  =  a(x - 3)² + 1

It passes through (1, 9). Substitute (x, y) = (1, 9). 

9  =  a(1 - 3)² + 1

9  =  a(-2)² + 1

9  =  4a + 1

Subtract 1 from each side. 

8  =  4a

Divide each side by 4.

2  =  a

Vertex form equation of the parabola : 

y  =  2(x - 3)² + 1

Problem 8 : 

Opens left or right, Vertex (-1, -2), Passes through (11, 0)

Solution : 

Vertex form equation of a parabola that opens left or right with vertex at (h, k) : 

x  =  a(y - k)² + h

Vertex (h, k) = (-1, -2).

x  =  a(y + 2)² - 1

It passes through (11, 0). Substitute (x, y) = (11, 0). 

11  =  a(0 + 2)² - 1

11  =  a(2)² - 1

11  =  4a - 1

Add 1 to each side. 

12  =  4a

Divide each side by 4.

3  =  a

Vertex form equation of the parabola :

x  =  3(y + 2)² - 1

Problem 9 : 

Write the intercept form equation of the parabola shown below.

Solution : 

Intercept form equation of the above parabola :

y  =  a(x - p)(x - q)

Because x-intercepts are (-1, 0) and (2, 0), 

x  =  -1 -----> x + 1  =  0

x  =  2 -----> x - 2  =  0

Then, 

y  =  a(x + 1)(x - 2)

It passes through (0, -4). Substitute (x, y) = (0, -4). 

-4  =  a(0 + 1)(0 - 2)

-4  =  a(1)(-2)

-4  =  -2a

Divide each side by -2.

2  =  a

Intercept form equation of the parabola :

y  =  2(x + 1)(x - 2)

Use the information provided to write the standard form equation of each parabola.

Problem 10 : 

Vertex (2, 3), Directrix : y = 6

Solution : 

Plot the vertex (2, 3) and directrix y = 6 on the xy-plane.

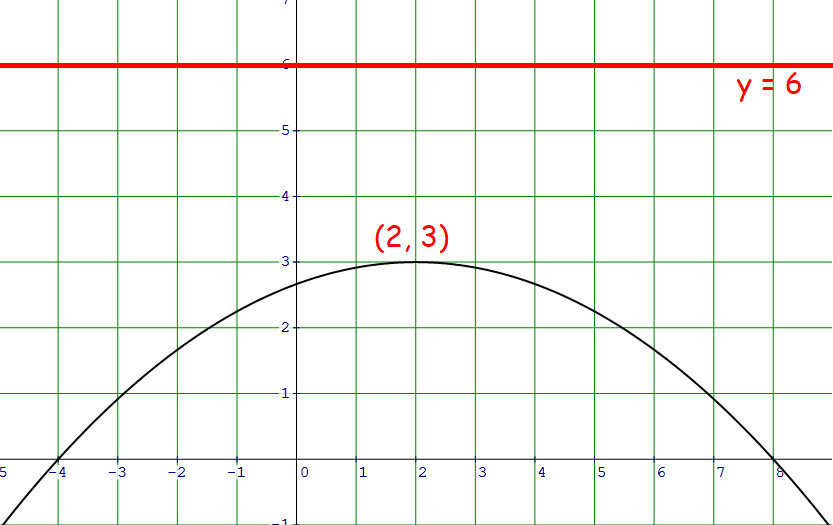
The parabola is open down with vertex at (2, 3).

Standard equation of a parabola that opens down with vertex at (h, k) : 

(x - h)²  =  -4a(y - k)

Vertex (h, k) = (2, 3).

(x - 2)²  =  -4a(y - 3)

Distance between the directrix and vertex is 3 units. 

That is, a = 3. 

(x - 2)²  =  -4(3)(y - 3)

(x - 2)²  =  -12(y - 3)

Problem 11 : 

Vertex (2, -2), Latus rectum : x = 1

Solution : 

Plot the vertex (2, -2) and latus rectum x = 1 on the xy-plane.

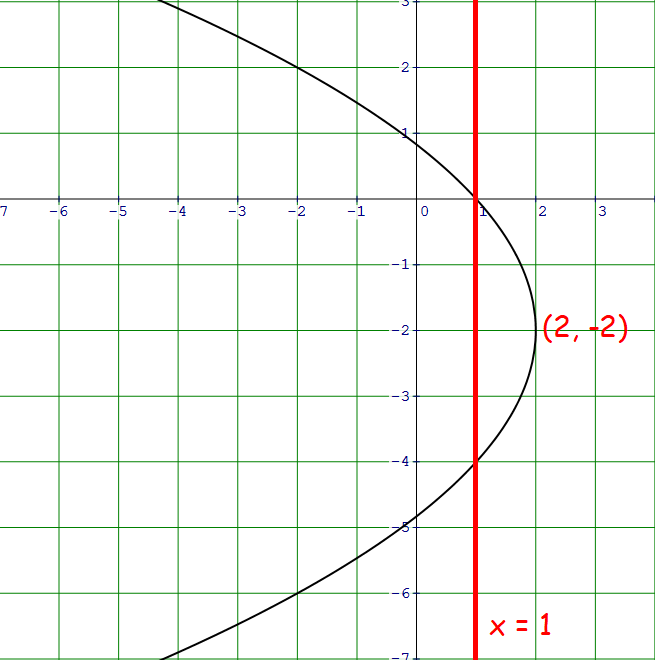
The parabola is open left with vertex at (2, -2).

Standard equation of a parabola that opens left with vertex at (h, k) : 

(y - k)²  =  -4a(x - h)

Vertex (h, k) = (2, -2).

(y + 2)²  =  -4a(x - 2)

Distance between the latus rectum and vertex is 1 unit. 

That is, a = 1. 

(y + 2)²  =  -4(1)(x - 2)

(y + 2)²  =  -4(x - 2)

Problem 12 : 

Opens up, Vertex (-3, 4), Length of Latus rectum : 8 units

Solution : 

Standard equation of a parabola that opens up with vertex at (h, k) : 

(x - h)²  =  4a(y - k)

Vertex (h, k) = (-3, 4).

(x + 3)²  =  4a(y - 4) -----(1)

Length of Latus rectum : 8. 

4a  =  8 

Divide each side by 4.

a  =  2

Substitute 2 for a in (1).

(x + 3)²  =  4(2)(y - 4)

(x + 3)²  =  8(y - 4)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.