EQUATION OF PERPENDICULAR BISECTOR
Consider the line AB and its perpendicular bisector CD shown below.
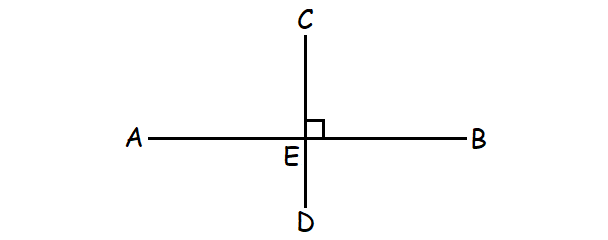
The following steps would be useful to find the equation of the perpendicular bisector CD.
Step 1 :
Find the slope of AB.
Step 2 :
Find the midpoint of AB which is E.
Step 3 :
Since AB and CD are perpendicular,
slope of AB x slope of CD = -1
slope of CD = -1/slope of AB
Step 4 :
Perpendicular bisector CD is passing through the mid point of AB which is E. In slope intercept form equation of a line 'y = mx + b', using the slope of AD and point E, find the y-intercept 'b'.
Step 5 :
Write the equation of perpendicular bisector CD using the slope of CD, 'm' and y-intercept 'b'.
Example :
Find the equation of perpendicular bisector of the line joining the points A(-4, 2) and B(6, -4) in general form.
Solution :
Let E be the mid point and CD be the perpendicular bisector of AB.
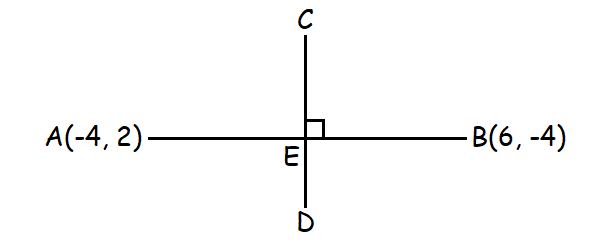
Mid point of AB :
= E((x1 + x2)/2, (y1 + y2)/2)
Substitute (x1, y1) = A(-4, 2) and (x2, y2) = B(6, -4).
= E((-4 + 6)/2, (2 - 4)/2)
= E(2/2, -2/2)
= E(1, -1)
Slope of AB :
= (y2 - y1)/(x2 - x1)
Substitute (x1, y1) = A(-4, 2) and (x2, y2) = B(6, -4).
= (-4 - 2)/(6 + 4)
= -6/10
= -3/5
Slope of AD :
= -1/slope of AB
= -1/(-3/5)
= 5/3
Equation of the perpendicular bisector CD :
y = mx + b
Substitute m = 5/3.
y = (5/3)x + b ----(1)
Substitute (x, y) = E(1, -1).
-1 = (5/3)(1) + b
-1 = 5/3 + b
-1 - 5/3 = b
-8/3 = b
(1)----> y = (5/3)x - 8/3
Multiply each side by 5.
3y = 5x - 8
-5x + 3y + 8 = 0
5x - 3y - 8 = 0

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Geometry : Two-Column Proofs Problems and Solutions
Feb 27, 25 11:57 AM
Geometry : Two-Column Proofs Problems and Solutions -
Digital SAT Math Problems and Solutions (Part - 117)
Feb 26, 25 12:09 PM
Digital SAT Math Problems and Solutions (Part - 117) -
SAT Math Resources (Videos, Concepts, Worksheets and More)
Feb 26, 25 12:08 PM
SAT Math Resources (Videos, Concepts, Worksheets and More)